6.4 Strengths of Ionic and Covalent Bonds

A Collective Work

Chapter 6: Chemical Bonding and Molecular Geometry

6.4 Strengths of Ionic and Covalent Bonds

Learning Outcomes

Describe the energetics of covalent and ionic bond formation and breakage
Use the Born-Haber cycle to compute lattice energies for ionic compounds
Use average covalent bond energies to estimate enthalpies of reaction

A bond’s strength describes how strongly each atom is joined to another atom, and therefore how much energy is required to break the bond between the two atoms. In this section, you will learn about the bond strength of covalent bonds, and then compare that to the strength of ionic bonds, which is related to the lattice energy of a compound.

Bond Strength: Covalent Bonds

Stable molecules exist because covalent bonds hold the atoms together. We measure the strength of a covalent bond by the energy required to break it, that is, the energy necessary to separate the bonded atoms. Separating any pair of bonded atoms requires energy (see Figure 6.4.1). The stronger a bond, the greater the energy required to break it.

A graph is shown with the x-axis labeled, “Internuclear distance ( p m )” while the y-axis is labeled, “Energy ( J ).” One value, “0,” is labeled midway up the y-axis and two values: “0” at the far left and “0.74” to the left, are labeled on the x-axis. The point “0.74” is labeled, “H bond H distance.” A line is graphed that begins near the top of the y-axis and to the far left on the x-axis and drops steeply to a point labeled, “negative 7.24 times 10 superscript negative 19 J” on the y-axis and 0.74 on the x-axis. This low point on the graph corresponds to a drawing of two spheres that overlap considerably. The line then rises to zero on the y-axis and levels out. The point where it almost reaches zero corresponds to two spheres that overlap slightly. The line at zero on the y-axis corresponds to two spheres that are far from one another. — Figure 6.4.1 The potential energy of two separate hydrogen atoms (right) decreases as they approach each other, and the single electrons on each atom are shared to form a covalent bond. The bond length is the internuclear distance at which the lowest potential energy is achieved.

The energy required to break a specific covalent bond in one mole of gaseous molecules is called the bond energy (D) or the bond dissociation energy. The bond energy for a diatomic molecule, D_X–Y, is defined as the standard enthalpy change for the endothermic reaction:

$XY (g) \to X (g) + Y (g) D_{X-Y} = Δ H^{\circ}$

For example, the bond energy of the pure covalent $H - H$ bond, D_{$H - H$}, is 436 kJ per mole of $H - H$ bonds broken:

$H_{2} (g) \to 2 H (g) D_{H - H} = Δ H^{\circ} = 436 kJ$

Molecules with three or more atoms have two or more bonds. The sum of all bond energies in such a molecule is equal to the standard enthalpy change for the endothermic reaction that breaks all the bonds in the molecule. For example, the sum of the four $C - H$ bond energies in $CH_{4}$ , 1660 kJ, is equal to the standard enthalpy change of the reaction:

The average $C - H$ bond energy, D_{$C - H$}, is 1660/4 = 415 kJ/mol because there are four moles of $C - H$ bonds broken per mole of the reaction. Although the four $C - H$ bonds are equivalent in the original molecule, they do not each require the same energy to break; once the first bond is broken (which requires 439 kJ/mol), the remaining bonds are easier to break. The 415 kJ/mol value is the average, not the exact value required to break any one bond.

The strength of a bond between two atoms increases as the number of electron pairs in the bond increases. Generally, as the bond strength increases, the bond length decreases. Thus, we find that triple bonds are stronger and shorter than double bonds between the same two atoms; likewise, double bonds are stronger and shorter than single bonds between the same two atoms. Average bond energies for some common bonds appear in Table 6.4.1, and a comparison of bond lengths and bond strengths for some common bonds appears in Table 6.4.2. When one atom bonds to various atoms in a group, the bond strength typically decreases as we move down the group. For example, $C - F$ is 439 kJ/mol, $C - Cl$ is 330 kJ/mol, and $C - Br$ is 275 kJ/mol.

Table 6.4.1. Bond Energies (kJ/mol)
Bond	Bond Energy	Bond	Bond Energy	Bond	Bond Energy
$H - H$	436	$C - S$	260	$F - Cl$	255
$H - C$	415	$C - Cl$	330	$F - Br$	235
$H - N$	390	$C - Br$	275	$Si - Si$	230
$H - O$	464	$C - I$	240	$Si - P$	215
$H - F$	569	$N - N$	160	$Si - S$	225
$H - Si$	395	$N = N$	418	$Si - Cl$	359
$H - P$	320	$N \equiv N$	946	$Si - Br$	290
$H - S$	340	$N - O$	200	$Si - I$	215
$H - Cl$	432	$N - F$	270	$P - P$	215
$H - Br$	370	$N - P$	210	$P - S$	230
$H - I$	295	$N - Cl$	200	$P - Cl$	330
$C - C$	345	$N - Br$	245	$P - Br$	270
$C = C$	611	$O - O$	140	$P - I$	215
$C \equiv C$	837	$O = O$	498	$S - S$	215
$C - N$	290	$O - F$	160	$S - Cl$	250
$C = N$	615	$O - Si$	370	$S - Br$	215
$C \equiv N$	891	$O - P$	350	$Cl - Cl$	243
$C - O$	350	$O - Cl$	205	$Cl - Br$	220
$C = O$	799	$O - I$	200	$Cl - I$	210
$C \equiv O$	1080	$F - F$	160	$Br - Br$	190
$C - F$	439	$F - Si$	540	$Br - I$	180
$C - Si$	360	$F - P$	489	$I - I$	150
$C - P$	265	$F - S$	285

Table 6.4.2. Average Bond Lengths and Bond Energies for Some Common Bonds
Bond	Bond Length (Å)	Bond Energy (kJ/mol)
$C–C$	1.54	345
$C = C$	1.34	611
$C \equiv C$	1.20	837
$C - N$	1.43	290
$C = N$	1.38	615
$C \equiv N$	1.16	891
$C - O$	1.43	350
$C = O$	1.23	741
$C \equiv O$	1.13	1080

We can use bond energies to calculate approximate enthalpy changes for reactions where enthalpies of formation are not available. Calculations of this type will also tell us whether a reaction is exothermic or endothermic. An exothermic reaction ( $Δ$ H negative, heat produced) results when the bonds in the products are stronger than the bonds in the reactants. An endothermic reaction ( $Δ$ H positive, heat absorbed) results when the bonds in the products are weaker than those in the reactants.

The enthalpy change, ΔH, for a chemical reaction is approximately equal to the sum of the energy required to break all bonds in the reactants (energy “in,” positive sign) plus the energy released when all bonds are formed in the products (energy “out,” negative sign). This can be expressed mathematically in the following way:

$Δ H = {Σ D}_{bonds broken} - {Σ D}_{bonds formed}$

In this expression, the symbol Ʃ means “the sum of” and D represents the bond energy in kilojoules per mole, which is always a positive number. The bond energy is obtained from a table (like Table 6.4.2) and will depend on whether the particular bond is a single, double, or triple bond. Thus, in calculating enthalpies in this manner, it is important that we consider the bonding in all reactants and products. Because D values are typically averages for one type of bond in many different molecules, this calculation provides a rough estimate, not an exact value, for the enthalpy of reaction.

Consider the following reaction:

$H_{2} (g) + {Cl}_{2} (g) \to 2 HCl (g)$
or
$H - H (g) + Cl - Cl (g) \to 2 H - Cl (g)$

To form two moles of $HCl$ , one mole of $H - H$ bonds and one mole of $Cl - Cl$ bonds must be broken. The energy required to break these bonds is the sum of the bond energy of the $H - H$ bond (436 kJ/mol) and the $Cl - Cl$ bond (243 kJ/mol). During the reaction, two moles of $H - Cl$ bonds are formed (bond energy = 432 kJ/mol), releasing 2 × 432 kJ; or 864 kJ. Because the bonds in the products are stronger than those in the reactants, the reaction releases more energy than it consumes:

$\begin{array}{lll} Δ H & = & {Σ D}_{bonds broken} - {Σ D}_{bonds formed} \\ Δ H & = & [D_{H - H} + D_{Cl - Cl}] - 2 D_{H - Cl} \\ = & [436 + 243] - 2 (432) = - 185 kJ \end{array}$

This excess energy is released as heat, so the reaction is exothermic. Standard Thermodynamic Properties for Selected Substances gives a value for the standard molar enthalpy of formation of $HCl (g)$ , $Δ H_{f}^{\circ}$ of $- 92.307$ kJ/mol. Twice that value is $- 184.6$ kJ, which agrees well with the answer obtained earlier for the formation of two moles of $HCl$ .

Example 6.4.1: Using Bond Energies to Calculate Approximate Enthalpy Changes

Methanol, $CH_{3} OH$ , may be an excellent alternative fuel. The high-temperature reaction of steam and carbon produces a mixture of the gases carbon monoxide, $CO$ , and hydrogen, $H_{2}$ , from which methanol can be produced. Using the bond energies in Table 2, calculate the approximate enthalpy change, ΔH, for the reaction here:

$CO (g) + 2 H_{2} (g) \to {CH}_{3} OH (g)$

Show Solution

First, we need to write the Lewis structures of the reactants and the products:

From this, we see that ΔH for this reaction involves the energy required to break a $C - O$ triple bond and two H–H single bonds, as well as the energy produced by the formation of three $C - H$ single bonds, a $C - O$ single bond, and an $O - H$ single bond. We can express this as follows:

$\begin{array}{rcl} Δ H & = & Σ_{bonds broken} - Σ D_{bonds formed} \\ Δ H & = & [D_{C \equiv O} + 2 (D_{H - H})] - [3 (D_{C - H}) + D_{C - O} + D_{O - H}] \end{array}$

Using the bond energy values in Table 2, we obtain:

$\begin{array}{ll} Δ H & = [1080 + 2 (436)] - [3 (415) + 350 + 464] \\ = - 107 kJ \end{array}$

We can compare this value to the value calculated based on $Δ H_{f}^{\circ}$ data from Standard Thermodynamic Properties for Selected Substances:

$\begin{array}{ll} Δ H & = [Δ H_{f}^{\circ} {CH}_{3} OH (g)] - [Δ H_{f}^{\circ} CO (g) + 2 \times Δ H_{f}^{\circ} H_{2}] \\ = [- 201.0] - [- 110.52 + 2 \times 0] \\ = - 90.5 kJ \end{array}$

Note that there is a fairly significant gap between the values calculated using the two different methods. This occurs because D values are the average of different bond strengths; therefore, they often give only rough agreement with other data.

Check Your Learning

Ionic Bond Strength and Lattice Energy

An ionic compound is stable because of the electrostatic attraction between its positive and negative ions. The lattice energy of a compound is a measure of the strength of this attraction. The lattice energy (ΔH_lattice) of an ionic compound is defined as the energy required to separate one mole of the solid into its component gaseous ions. For the ionic solid MX, the lattice energy is the enthalpy change of the process:

$MX (s) \to M^{n +} (g) + X^{n -} (g) Δ H_{lattice}$

Note that we are using the convention where the ionic solid is separated into ions, so our lattice energies will be endothermic (positive values). Some texts use the equivalent but opposite convention, defining lattice energy as the energy released when separate ions combine to form a lattice and giving negative (exothermic) values. Thus, if you are looking up lattice energies in another reference, be certain to check which definition is being used. In both cases, a larger magnitude for lattice energy indicates a more stable ionic compound. For sodium chloride, ΔH_lattice = 769 kJ. Thus, it requires 769 kJ to separate one mole of solid $NaCl$ into gaseous $Na^{+}$ and $Cl^{-}$ ions. When one mole each of gaseous $Na^{+}$ and $Cl^{-}$ ions form solid $NaCl$ , 769 kJ of heat is released.

The lattice energy ΔH_lattice of an ionic crystal can be expressed by the following equation (derived from Coulomb’s law, governing the forces between electric charges):

$Δ H_{lattice} = \frac{C (Z^{+}) (Z^{-})}{R_{o}}$

in which $C$ is a constant that depends on the type of crystal structure; Z⁺ and Z^– are the charges on the ions; and R_o is the interionic distance (the sum of the radii of the positive and negative ions). Thus, the lattice energy of an ionic crystal increases rapidly as the charges of the ions increase and the sizes of the ions decrease. When all other parameters are kept constant, doubling the charge of both the cation and anion quadruples the lattice energy. For example, the lattice energy of $LiF$ (Z⁺ and Z^– = 1) is 1023 kJ/mol, whereas that of MgO (Z⁺ and Z^– = 2) is 3900 kJ/mol (R_o is nearly the same—about 200 pm for both compounds).

Different interatomic distances produce different lattice energies. For example, we can compare the lattice energy of $MgF_{2}$ (2957 kJ/mol) to that of $MgI_{2}$ (2327 kJ/mol) to observe the effect on lattice energy of the smaller ionic size of F^– as compared to I^–.

Example 6.4.2: Lattice Energy Comparisons

The precious gem ruby is aluminum oxide, $Al_{2} O_{3}$ , containing traces of $Cr^{3 +}$ . The compound $Al_{2} Se_{3}$ is used in the fabrication of some semiconductor devices. Which has the larger lattice energy, $Al_{2} O_{3}$ or $Al_{2} Se_{3}$ ?

Show Solution

In these two ionic compounds, the charges Z⁺ and Z^– are the same, so the difference in lattice energy will depend upon R_o. The $O^{2 -}$ ion is smaller than the $Se^{2 -}$ ion. Thus, $Al_{2} O_{3}$ would have a shorter interionic distance than $Al_{2} Se_{3}$ , and $Al_{2} O_{3}$ would have the larger lattice energy.

Check Your Understanding

The Born-Haber Cycle

It is not possible to measure lattice energies directly. However, the lattice energy can be calculated using the equation given in the previous section above or by using a thermochemical cycle. The Born-Haber cycle is an application of Hess’s law that breaks down the formation of an ionic solid into a series of individual steps:

$Δ H_{f}^{\circ}$ , the standard enthalpy of formation of the compound
$I E$ , the ionization energy of the metal
$E A$ , the electron affinity of the nonmetal
$Δ H_{s}^{\circ}$ , the enthalpy of sublimation of the metal
$D$ , the bond dissociation energy of the nonmetal
$Δ H_{lattice}$ , the lattice energy of the compound

Figure 6.4.2 diagrams the Born-Haber cycle for the formation of solid cesium fluoride.

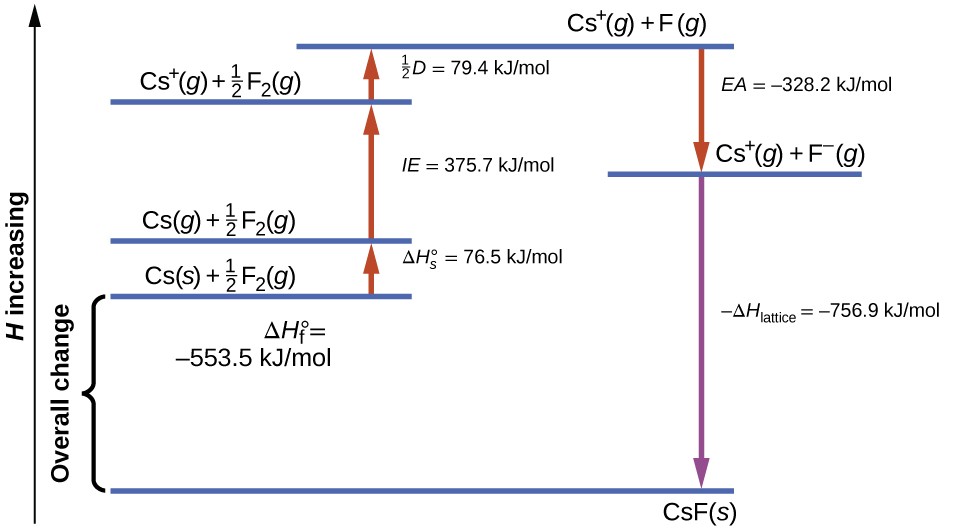
A diagram is shown. An upward facing arrow is drawn to the far left of the chart and is labeled “H increasing.” A horizontal line is drawn at the bottom of the chart. A downward-facing, vertical arrow to the left side of this line is labeled, “Overall change.” Beside this arrow is another label, “capital delta H subscript f, equals negative 553.5 k J per mol, ( Enthalpy of formation ).” Three horizontal lines, one above the other, and all above the bottom line, are labeled, from bottom to top, as: “C s ( s ), plus sign, one half F subscript 2, ( g ),” “C s ( g ), plus sign, one half F subscript 2, ( g ),” and “C s, superscript positive sign, ( g ), plus sign, one half F subscript 2, ( g ).” Each of these lines is connected by an upward-facing vertical arrow. Each arrow is labeled, “capital delta H subscript 1, equals 76.5 k J per mol, ( Enthalpy of sublimation ),” “capital delta H subscript 2, equals 375.7 k J per mol, ( ionization energy ),” and “capital delta H subscript 3 equals 79.4 k J / mol ( one half dissociation energy ).” Another horizontal line is drawn in the center top portion of the diagram and is labeled “C s, superscript positive sign, ( g ), plus sign, F, ( g ).” There is one more horizontal line drawn to the right of the overall diagram and located halfway down the image. An arrow connects the top line to this line and is labeled, “capital delta H equals negative 328.2 k J / mol ( electron affinity ).” The line is labeled, “C s superscript positive sign ( g ) plus F superscript negative sign ( g ).” The arrow connecting this line to the bottom line is labeled, “negative capital delta H subscript lattice equals negative 756.9 k J / mol.” The arrow points to a label on the bottom line which reads, “C s F ( s ).” — Figure 6.4.2. The Born-Haber cycle shows the relative energies of each step involved in the formation of an ionic solid from the necessary elements in their reference states.

We begin with the elements in their most common states, $Cs$ (s) and $F_{2}$ (g). The $Δ H_{s}^{\circ}$ represents the conversion of solid cesium into a gas, and then the ionization energy converts the gaseous cesium atoms into cations. In the next step, we account for the energy required to break the $F - F$ bond to produce fluorine atoms. Converting one mole of fluorine atoms into fluoride ions is an exothermic process, so this step gives off energy (the electron affinity) and is shown as decreasing along the y-axis. We now have one mole of $Cs$ cations and one mole of $F$ anions. These ions combine to produce solid cesium fluoride. The enthalpy change in this step is the negative of the lattice energy, so it is also an exothermic quantity. The total energy involved in this conversion is equal to the experimentally determined enthalpy of formation, $Δ H_{f}^{\circ},$ of the compound from its elements. In this case, the overall change is exothermic.

Hess’s law can also be used to show the relationship between the enthalpies of the individual steps and the enthalpy of formation. Table 6.4.3 shows this for cesium chloride, $CsF$ .

Table 6.4.3

Enthalpy of sublimation of

Cs

(s)

Cs (s) \to Cs (g)

Δ H = Δ H_{s}^{\circ} = 76.5 kJ/mol

One-half of the bond energy of

F_{2}

\frac{1}{2} F_{2} (g) \to F (g)

Δ H = \frac{1}{2} D = 79.4 kJ/mol

Ionization energy of

Cs

(g)

Cs (g) \to {Cs}^{+} (g) + e^{-}

Δ H = I E = 375.7 kJ/mol

Negative of the electron affinity of

F

F (g) + e^{-} \to F^{-} (g)

Δ H = E A = - 328.2 kJ/mol

Negative of the lattice energy of

CsF

(s)

{Cs}^{+} (g) + F^{-} (g) \to CsF (s)

Δ H = - {Δ H}_{lattice} = ?

Enthalpy of formation of

CsF

(s), add steps 1–5

\begin{array}{l} Δ H = Δ H_{f}^{\circ} = Δ H_{s}^{\circ} + \frac{1}{2} D + I E + (E A) + (- {Δ H}_{lattice}) \\ Cs (s) + \frac{1}{2} F_{2} (g) \to CsF (s) \end{array}

Δ H = - 553.5 kJ/mol

Thus, the lattice energy can be calculated from other values. For cesium chloride, using this data, the lattice energy is:

$Δ H_{lattice} = 76.5 + 79.4 + 375.7 + (- 328.2) - (- 553.5) = 756.9 kJ/mol$

The Born-Haber cycle may also be used to calculate any one of the other quantities in the equation for lattice energy, provided that the remainder is known. For example, if the relevant enthalpy of sublimation $Δ H_{s}^{\circ},$ ionization energy (IE), bond dissociation enthalpy (D), lattice energy $Δ H_{lattice}$ _, and standard enthalpy of formation $Δ H_{f}^{\circ}$ are known, the Born-Haber cycle can be used to determine the electron affinity of an atom.

Lattice energies calculated for ionic compounds are typically much higher than bond dissociation energies measured for covalent bonds. Whereas lattice energies typically fall in the range of 600–4000 kJ/mol (some even higher), covalent bond dissociation energies are typically between 150–400 kJ/mol for single bonds. Keep in mind, however, that these are not directly comparable values. For ionic compounds, lattice energies are associated with many interactions, as cations and anions pack together in an extended lattice. For covalent bonds, the bond dissociation energy is associated with the interaction of just two atoms.

Key Concepts and Summary

The strength of a covalent bond is measured by its bond dissociation energy, that is, the amount of energy required to break that particular bond in a mole of molecules. Multiple bonds are stronger than single bonds between the same atoms. The enthalpy of a reaction can be estimated based on the energy input required to break bonds and the energy released when new bonds are formed. For ionic bonds, the lattice energy is the energy required to separate one mole of a compound into its gas phase ions. Lattice energy increases for ions with higher charges and shorter distances between ions. Lattice energies are often calculated using the Born-Haber cycle, a thermochemical cycle including all of the energetic steps involved in converting elements into an ionic compound.

Key Equations

Bond energy for a diatomic molecule: $XY (g) \to X (g) + Y (g) D_{X-Y} = Δ H^{\circ}$
Enthalpy change: $Δ H = {Σ D}_{bonds broken} - {Σ D}_{bonds formed}$
Lattice energy for a solid MX: $MX (s) \to M^{n +} (g) + X^{n -} (g) Δ H_{lattice}$
Lattice energy for an ionic crystal: $Δ H_{lattice} = \frac{C (Z^{+}) (Z^{-})}{R_{o}}$

Try It

Which bond in each of the following pairs of bonds is the strongest?
1. $C - C$ or $C = C$
2. $C - N$ or $C \equiv N$
3. $C \equiv O$ or $C = O$
4. $H - F$ or $H - Cl$
5. $C - H$ or $O - H$
6. $C - N$ or $C - O$
How does the bond energy of $HCl$ (g) differ from the standard enthalpy of formation of $HCl$ (g)?
Using the standard enthalpy of formation data in Standard Thermodynamic Properties for Selected Substances, show how can the standard enthalpy of formation of $HCl$ (g) can be used to determine the bond energy.
Using the standard enthalpy of formation data in Standard Thermodynamic Properties for Selected Substances, determine which bond is stronger: the $S - F$ bond in $SF_{4}$ (g) or in $SF_{6}$ (g)?

Selected Answers

In general, a multiple bond between the same two elements is stronger than a single bond. The greater the electronegativity difference between two similar elements, the greater the bond energy
1. $C = C$
2. $C \equiv N$
3. $C \equiv O$
4. $H - F$
5. $O - H$
6. $C - O$
The bond energy involves breaking $HCl$ into $H$ and $Cl$ atoms. The enthalpy of formation involves making $HCl$ from $H_{2}$ and $Cl_{2}$ molecules.

Glossary

bond energy: (also, bond dissociation energy) energy required to break a covalent bond in a gaseous substance

Born-Haber cycle: thermochemical cycle relating the various energetic steps involved in the formation of an ionic solid from the relevant elements

lattice energy (ΔH_lattice): energy required to separate one mole of an ionic solid into its component gaseous ions

Licenses and Attributions (Click to expand)

CC licensed content, Shared previously

Chemistry 2e. Provided by: OpenStax. Located at: https://openstax.org/. License: CC BY: Attribution. License Terms: Access for free at
https://openstax.org/books/chemistry-2e/pages/1-introduction

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chemistry Fundamentals Copyright © by Dr. Julie Donnelly, Dr. Nicole Lapeyrouse, and Dr. Matthew Rex is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.