Chapter 2: Atoms, Molecules, and Ions
2.5 The Mole Concept
Learning Outcomes
- Determine the atomic mass of elements on the periodic table
- Define the amount unit mole and the related quantity Avogadro’s number
- Explain the relation between mass, moles, and numbers of atoms or molecules, and perform calculations deriving these quantities from one another
We can argue that modern chemical science began when scientists started exploring the quantitative as well as the qualitative aspects of chemistry. For example, Dalton’s atomic theory was an attempt to explain the results of measurements that allowed him to calculate the relative masses of elements combined in various compounds. Understanding the relationship between the masses of atoms and the chemical formulas of compounds allows us to quantitatively describe the composition of substances.
The Mole
The identity of a substance is defined not only by the types of atoms or ions it contains, but by the quantity of each type of atom or ion. For example, water, [latex]\ce{H2O}[/latex], and hydrogen peroxide, [latex]\ce{H2O2}[/latex], are alike in that their respective molecules are composed of hydrogen and oxygen atoms. However, because a hydrogen peroxide molecule contains two oxygen atoms, as opposed to the water molecule, which has only one, the two substances exhibit very different properties. Today, we possess sophisticated instruments that allow the direct measurement of these defining microscopic traits; however, the same traits were originally derived from the measurement of macroscopic properties (the masses and volumes of bulk quantities of matter) using relatively simple tools (balances and volumetric glassware). This experimental approach required the introduction of a new unit for amount of substances, the mole, which remains indispensable in modern chemical science.
The mole is an amount unit similar to familiar units like pair, dozen, gross, etc. It provides a specific measure of the number of atoms or molecules in a bulk sample of matter. One Latin connotation for the word “mole” is “large mass” or “bulk,” which is consistent with its use as the name for this unit. The mole provides a link between an easily measured macroscopic property, bulk mass, and an extremely important fundamental property, number of atoms, molecules, and so forth. A mole of a substance is that amount in which there are 6.02214179 × 1023 discrete entities (atoms or molecules). This larger number is a fundamental constant known as Avogadro’s number (NA) or the Avogadro constant in honor of Italian scientist Amedeo Avogadro. This constant is properly reported with an explicit unit of “per mole,” a conveniently rounded version being 6.022 × 1023 mol.
Consistent with its definition as an amount unit, 1 mole of any element contains the same number of atoms as 1 mole of any other element. The masses of 1 mole of different elements, however, are different, since the masses of the individual atoms are drastically different. The molar mass of an element (or compound) is the mass in grams of 1 mole of that substance, a property expressed in units of grams per mole (g/mol) (see Figure 2.5.1).
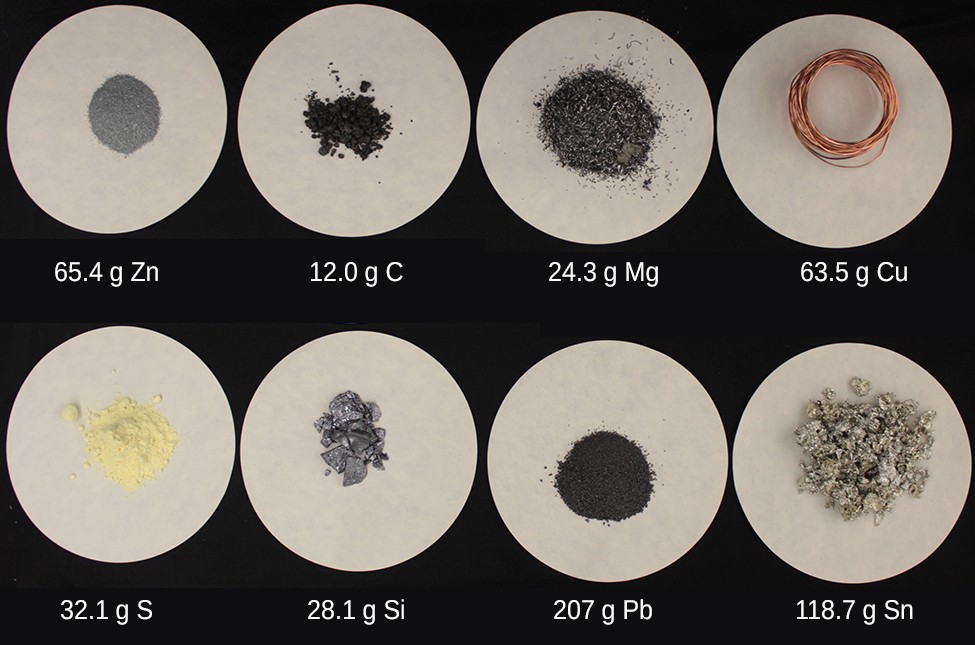
The atomic mass of any substance is numerically equivalent to its atomic or formula weight in amu. Per the amu definition, a single [latex]\ce{^{12}C}[/latex] atom weighs 12 amu (its atomic mass is 12 amu). A mole of [latex]\ce{^{12}C}[/latex] weighs 12 g (its molar mass is 12 g/mol). This relationship holds for all elements, since their atomic masses are measured relative to that of the amu-reference substance, [latex]\ce{^{12}C}[/latex]. Extending this principle, the molar mass of a compound in grams is likewise numerically equivalent to its formula mass in amu (Figure 2.5.2).
| Element | Average Atomic Mass (amu) | Molar Mass (g/mol) | Atoms/Mole |
|---|---|---|---|
| C | 12.01 | 12.01 | 6.022 × 1023 |
| H | 1.008 | 1.008 | 6.022 × 1023 |
| O | 16.00 | 16.00 | 6.022 × 1023 |
| Na | 22.99 | 22.99 | 6.022 × 1023 |
| Cl | 35.45 | 35.45 | 6.022 × 1023 |

While atomic mass and molar mass are numerically equivalent, keep in mind that they are vastly different in terms of scale, as represented by the vast difference in the magnitudes of their respective units (amu versus g). To appreciate the enormity of the mole, consider a small drop of water weighing about 0.03 g (see Figure 2.5.3). Although this represents just a tiny fraction of 1 mole of water (~18 g), it contains more water molecules than can be clearly imagined. If the molecules were distributed equally among the roughly seven billion people on earth, each person would receive more than 100 billion molecules.
Worked out examples
The relationships between molar mass, the mole, and Avogadro’s number can be applied to compute various quantities that describe the composition of substances and compounds, as demonstrated in the next several example problems.
Example 2.5.1: Deriving Moles from Grams for an Element
According to nutritional guidelines from the US Department of Agriculture, the estimated average requirement for dietary potassium is 4.7 g. What is the estimated average requirement of potassium in moles?
Show Solution
The mass of [latex]\ce{K}[/latex] is provided, and the corresponding amount of [latex]\ce{K}[/latex] in moles is requested. Referring to the periodic table, the atomic mass of [latex]\ce{K}[/latex] is 39.10 amu, and so its molar mass is 39.10 g/mol. The given mass of [latex]\ce{K}[/latex] (4.7 g) is a bit more than one-tenth the molar mass (39.10 g), so a reasonable “ballpark” estimate of the number of moles would be slightly greater than 0.1 mol.

The molar amount of a substance may be calculated by dividing its mass (g) by its molar mass (g/mol):
The factor-label method supports this mathematical approach since the unit “g” cancels and the answer has units of “mol:”
[latex]4.7\cancel{\text{g}}\ce{K}\left(\dfrac{\text{mol } \ce{K}}{39.10\cancel{\text{g}}}\right)=0.12\text{mol } \ce{K}[/latex]
The calculated magnitude (0.12 mol [latex]\ce{K}[/latex]) is consistent with our ballpark expectation, since it is a bit greater than 0.1 mol.
Check Your Learning
Example 2.5.2: Deriving Grams from Moles for an Element
A liter of air contains 9.2 × 10-4 mol argon. What is the mass of [latex]\ce{Ar}[/latex] in a liter of air?
Show Solution

The molar amount of [latex]\ce{Ar}[/latex] is provided and must be used to derive the corresponding mass in grams. Since the amount of [latex]\ce{Ar}[/latex] is less than 1 mole, the mass will be less than the mass of 1 mole of [latex]\ce{Ar}[/latex], approximately 40 g. The molar amount in question is approximately one-one thousandth 10-3 of a mole, and so the corresponding mass should be roughly one-one thousandth of the molar mass 0.04 g:
In this case, logic dictates (and the factor-label method supports) multiplying the provided amount (mol) by the molar mass (g/mol):
[latex]9.2\times {10}^{-4}\cancel{\text{mol}}\text{Ar}\left(\dfrac{39.95\text{g}}{\cancel{\text{mol}}\text{Ar}}\right)=0.037\text{g Ar}[/latex]
The result is in agreement with our expectations as noted above, around 0.04 g [latex]\ce{Ar}[/latex].
Check Your Learning
Example 2.5.3: Deriving Number of Atoms from Mass for an Element
Copper is commonly used to fabricate electrical wire (Figure 2.5.4). How many copper atoms are in 5.00 g of copper wire?
Show Solution

The number of [latex]\ce{Cu}[/latex] atoms in the wire may be conveniently derived from its mass by a two-step computation: first calculating the molar amount of [latex]\ce{Cu}[/latex], and then using Avogadro’s number (NA) to convert this molar amount to number of [latex]\ce{Cu}[/latex] atoms. Considering that the provided sample mass (5.00 g) is a little less than one-tenth the mass of 1 mole of [latex]\ce{Cu}[/latex] (~64 g), a reasonable estimate for the number of atoms in the sample would be on the order of one-tenth NA, or approximately 1022 [latex]\ce{Cu}[/latex] atoms. Carrying out the two-step computation yields:
[latex]5.00\cancel{\text{g}}\ce{Cu}\left(\dfrac{\cancel{\text{mol}}\ce{Cu}}{63.55\cancel{\text{g}}}\right)\left(\dfrac{6.022\times {10}^{23}\text{atoms}}{\cancel{\text{mol}}}\right)=4.74\times {10}^{22}\text{atoms of copper}[/latex]
The factor-label method yields the desired cancellation of units, and the computed result is on the order of 1022 as expected.
Check Your Learning
Key Concepts and Summary
The formula mass of a substance is the sum of the average atomic masses of each atom represented in the chemical formula and is expressed in atomic mass units. The formula mass of a compound is also called the molecular mass. A convenient amount unit for expressing very large numbers of atoms or molecules is the mole. Experimental measurements have determined the number of entities composing 1 mole of substance to be 6.022 × 1023, a quantity called Avogadro’s number. The mass in grams of 1 mole of substance is its molar mass. Due to the use of the same reference substance in defining the atomic mass unit and the mole, the formula mass (amu) and molar mass (g/mol) for any substance are numerically equivalent (for example, one [latex]\ce{H2O}[/latex] molecule weighs approximately18 amu and 1 mole of [latex]\ce{H2O}[/latex] molecules weighs approximately 18 g).
Try It
- Determine the mass in grams of each of the following:
- 0.600 mol of oxygen atoms
- A 55-kg woman has 7.5 × 10-3 mol of hemoglobin (molar mass = 64,456 g/mol) in her blood. How many hemoglobin molecules is this? What is this quantity in grams?
- Determine the number of atoms and the mass of zirconium, silicon, and oxygen found in 0.3384 mol of zircon, [latex]\ce{ZrSiO4}[/latex], a semiprecious stone.
- Diamond is one form of elemental carbon. An engagement ring contains a diamond weighing 1.25 carats (1 carat = 200 mg). How many atoms are present in the diamond?
- The Cullinan diamond was the largest natural diamond ever found (January 25, 1905). It weighed 3104 carats (1 carat = 200 mg). How many carbon atoms were present in the stone
- One 55-gram serving of a particular cereal supplies 270 mg of sodium, 11% of the recommended daily allowance. How many moles and atoms of sodium are in the recommended daily allowance?
Show Solutions
- The mass of each compound is as follows:
- [latex]0.600\cancel{\text{mol}}\times 15.9994\text{g/}\cancel{\text{mol}}=9.60\text{g}[/latex]
- Determine the number of moles of each component. From the moles, calculate the number of atoms and the mass of the elements involved.
- Zirconium: [latex]0.3384\cancel{\text{mol}}\times 6.022\times {10}^{23}{\cancel{\text{mol}}}^{\cancel{-1}}=2.038\times 1023\text{atoms;}0.3384\cancel{\text{mol}}\times 91.224\text{g/}\cancel{\text{mol}}=30.87\text{g;}[/latex]
- Silicon: [latex]0.3384\cancel{\text{mol}}\times 6.022\times {10}^{23}{\cancel{\text{mol}}}^{\cancel{-1}}=2.038\times {10}^{23}\text{atoms;}0.3384\cancel{\text{mol}}\times 28.0855\text{g/}\cancel{\text{mol}}=9.504\text{g;}[/latex]
- Oxygen: [latex]4\times 0.3384\cancel{\text{mol}}\times 6.022\times {10}^{23}{\cancel{\text{mol}}}^{\cancel{-1}}=8.151\times {10}^{23}\text{atoms;}4\times 0.3384\cancel{\text{mol}}\times 15.9994\text{g/}\cancel{\text{mol}}=21.66\text{g}[/latex]
- Determine the number of grams present in the diamond and from that the number of moles. Find the number of carbon atoms by multiplying Avogadro’s number by the number of moles:
- [latex]\dfrac{3104\cancel{\text{carats}}\times \dfrac{200\cancel{\text{mg}}}{1\cancel{\text{carat}}}\times \dfrac{1\cancel{\text{g}}}{1000\cancel{\text{mg}}}}{12.011\cancel{\text{g}}\cancel{{\text{mol}}^{-1}}\left(6.022\times {10}^{23}\cancel{{\text{mol}}^{-1}}\right)}=3.113\times {10}^{25}\text{C atoms}[/latex]
Glossary
Avogadro’s number (NA): experimentally determined value of the number of entities comprising 1 mole of substance, equal to 6.022 × 1023 mol-1
Atomic mass: mass in grams of 1 mole of a substance
mole: amount of substance containing the same number of atoms, molecules, ions, or other entities as the number of atoms in exactly 12 grams of [latex]\ce{^{12}C}[/latex]
amount of substance containing the same number of atoms, molecules, ions, or other entities as the number of atoms in exactly 12 grams of 12C
experimentally determined value of the number of entities comprising 1 mole of substance, equal to [latex]6.022\times {10}^{23}{\text{mol}}^{-1}[/latex]

