Chapter 9: Gases
9.3 Applications of the Ideal Gas Law
Learning Outcomes
- Use the ideal gas law to calculate the density and molar mass of an ideal gas
The study of the chemical behavior of gases was part of the basis of perhaps the most fundamental chemical revolution in history. French nobleman Antoine Lavoisier, widely regarded as the “father of modern chemistry,” changed chemistry from a qualitative to a quantitative science through his work with gases. He discovered the law of conservation of matter, discovered the role of oxygen in combustion reactions, determined the composition of air, explained respiration in terms of chemical reactions, and more. He was a casualty of the French Revolution, guillotined in 1794. Of his death, mathematician and astronomer Joseph-Louis Lagrange said, “It took the mob only a moment to remove his head; a century will not suffice to reproduce it.”
As described in an earlier chapter of this text, we can turn to chemical stoichiometry for answers to many of the questions that ask “How much?” We can answer the question with masses of substances or volumes of solutions. However, we can also answer this question another way: with volumes of gases. We can use the ideal gas equation to relate the pressure, volume, temperature, and number of moles of a gas. Here we will combine the ideal gas equation with other equations to find gas density and molar mass. We will deal with mixtures of different gases, and calculate amounts of substances in reactions involving gases. This section and the following two sections will not introduce any new material or ideas, but will provide examples of applications and ways to integrate concepts we have already discussed.
Gas Density and Molar Mass
The ideal gas law described previously in this chapter relates the properties of pressure P, volume V, temperature T, and molar amount n. This law is universal, relating these properties in identical fashion regardless of the chemical identity of the gas:
PV=nRT
The density d of a gas, on the other hand, is determined by its identity. As described in another chapter of this text, the density of a substance is a characteristic property that may be used to identify the substance.
[latex]d=\dfrac{m}{v}[/latex]
Rearranging the ideal gas equation to isolate V and substituting into the density equation yields
[latex]d=\dfrac{mP}{nRT}=(\dfrac{m}{n})\dfrac{P}{RT}[/latex]
The ratio m/n is the definition of molar mass, ℳ:
[latex]ℳ=\dfrac{m}{n}[/latex]
The density equation can then be written
[latex]d=\dfrac{\mathscr{M}P}{RT}[/latex]
This relation may be used for calculating the densities of gases of known identities at specified values of pressure and temperature as demonstrated in Example 9.3.1.
Example 9.3.1: Measuring Gas Density
What is the density of molecular nitrogen gas at STP?
Show Solution
The molar mass of molecular nitrogen, [latex]\ce{N2}[/latex], is 28.01 g/mol. Substituting this value along with standard temperature and pressure into the gas density equation yields
[latex]d=\dfrac{ℳP}{RT}=\dfrac{(28.01 \text{g/mol})(1.00 \text{atm})}{(0.721 \text{L}*\text{atm}*\text{mol}^-1\text{K}^-1)(273 \text{K})} = 1.25 \text{g/L}[/latex]
Check Your Learning
When the identity of a gas is unknown, measurements of the mass, pressure, volume, and temperature of a sample can be used to calculate the molar mass of the gas (a useful property for identification purposes). Combining the ideal gas equation
[latex]PV=nRT[/latex]
and the definition of molarity
[latex]ℳ=\dfrac{m}{n}[/latex]
yields the following equation:
[latex]ℳ=\dfrac{mRT}{PV}[/latex]
Determining the molar mass of a gas via this approach is demonstrated in Example 9.3.2.
Example 9.3.2: Empirical/Molecular Formula Problems Using the Ideal Gas Law and Density of a Gas
Cyclopropane, a gas once used with oxygen as a general anesthetic, is composed of 85.7% carbon and 14.3% hydrogen by mass. Find the empirical formula. If 1.56 g of cyclopropane occupies a volume of 1.00 L at 0.984 atm and 50 °C, what is the molecular formula for cyclopropane?
Show Solution
Strategy: First solve the empirical formula problem using methods discussed earlier. Assume 100 g and convert the percentage of each element into grams. Determine the number of moles of carbon and hydrogen in the 100-g sample of cyclopropane. Divide by the smallest number of moles to relate the number of moles of carbon to the number of moles of hydrogen. In the last step, realize that the smallest whole number ratio is the empirical formula:
[latex]\text{85.7 g } \ce{C}\times \dfrac{\text{1 mol } \ce{C}}{\text{12.01 g } \ce{C}}=\text{7.136 mol } \ce{C}\qquad\dfrac{7.136}{7.136}=\text{1.00 mol} \ce{C}[/latex]
[latex]\text{14.3 g } \ce{H}\times \dfrac{\text{1 mol } \ce{H}}{\text{1.01 g } \ce{H}}=\text{14.158 mol } \ce{H}\qquad\dfrac{14.158}{7.136}=\text{1.98 mol } \ce{H}[/latex]
Empirical formula is [latex]\ce{CH2}[/latex] [empirical mass [latex](EM)[/latex] of 14.03 g/empirical unit].
Next, use the provided values for mass, pressure, temperature and volume to compute the molar mass of the gas:
[latex]ℳ=\dfrac{\text{mRT}}{PV}=\dfrac{(1.56\text{ g})(0.0821\text{L}\cdot\text{atm}\cdot\text{mol}^{-1}K^{-1})(323 K)}{(0.984\text{atm}(1.00\text{L})}=42.0\text{ g/mol}[/latex]
Comparing the molar mass to the empirical formula mass shows how many empirical formula units make up a molecule:
[latex]\dfrac{ℳ}{EM}=\dfrac{42.0\text{g/mol}}{14.0\text{g/mol}}=3[/latex]
The molecular formula is thus derived from the empirical formula by multiplying each of its subscripts by three:
[latex]\ce{(CH2)3} = \ce{C3H6}[/latex]
Check Your Learning
Example 9.3.3: Determining the Molar Mass of a Volatile Liquid
The approximate molar mass of a volatile liquid can be determined by:
- Heating a sample of the liquid in a flask with a tiny hole at the top, which converts the liquid into gas that may escape through the hole
- Removing the flask from heat at the instant when the last bit of liquid becomes gas, at which time the flask will be filled with only gaseous sample at ambient pressure
- Sealing the flask and permitting the gaseous sample to condense to liquid, and then weighing the flask to determine the sample’s mass (see Figure 9.3.1)
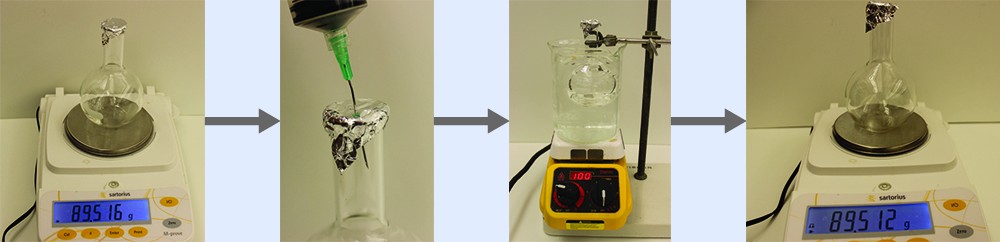
Using this procedure, a sample of chloroform gas weighing 0.494 g is collected in a flask with a volume of 129 cm3 at 99.6 °C when the atmospheric pressure is 742.1 mm Hg. What is the approximate molar mass of chloroform?
Show Solution
Since [latex]ℳ=\dfrac{m}{n}[/latex] and [latex]n=\dfrac{PV}{RT},[/latex] substituting and rearranging gives [latex]ℳ=\dfrac{mRT}{PV},[/latex] then
[latex]ℳ=\dfrac{mRT}{PV}=\dfrac{\left(\text{0.494 g}\right)\times \text{0.08206 L}\cdot\text{ atm/mol K}\times \text{372.8 K}}{\text{0.976 atm}\times \text{0.129 L}}=120\text{ g/mol}[/latex]
Check Your Learning
Key Concepts and Summary
The ideal gas law can be used to derive a number of convenient equations relating directly measured quantities to properties of interest for gaseous substances and mixtures. Appropriate rearrangement of the ideal gas equation may be made to permit the calculation of gas densities and molar masses.
Try It
- Calculate the density of Freon 12, [latex]\ce{CF2Cl2}[/latex], at 30.0°C and 0.954 atm.
- What is the molar mass of a gas if 0.281 g of the gas occupies a volume of 125 mL at a temperature 126 °C and a pressure of 777 torr?
Selected Answers
- [latex]\rho =\frac{P\mathcal{M}}{RT}=\frac{0.954\cancel{\text{atm}}\left[12.011+2\left(18.9954\right)+2\left(35.453\right)\right]\text{g}\cancel{{\text{mol}}^{-\text{1}}}}{\text{0.08206 L}\cancel{\text{atm}}\cancel{{\text{mol}}^{-\text{1}}}\cancel{{\text{K}}^{-\text{1}}}\times 303.15\cancel{\text{K}}}=\text{4.64 g}{\text{L}}^{-\text{1}}[/latex]
- From the ideal gas law, PV = nRT, set [latex]n=\frac{\text{mass}}{\text{molar mass}}[/latex] and solve the molar mass. [latex]\text{molar mass}=\frac{\left(\text{0.281 g}\right)\left(0.08206\cancel{\text{L}}\cancel{\text{atm}}{\text{mol}}^{-\text{1}}\cancel{{\text{K}}^{-\text{1}}}\right)\left(399.15\cancel{\text{K}}\right)}{\left(\frac{777\cancel{\text{torr}}}{760\cancel{\text{torr}}\cancel{{\text{atm}}^{-\text{1}}}}\right)\left(0.125\cancel{\text{L}}\right)}=\text{72.0 g}{\text{mol}}^{-\text{1}}[/latex]
Glossary
Dalton’s law of partial pressures: total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases.
mole fraction: concentration unit defined as the ratio of the molar amount of a mixture component to the total number of moles of all mixture components
partial pressure: pressure exerted by an individual gas in a mixture
vapor pressure of water: pressure exerted by water vapor in equilibrium with liquid water in a closed container at a specific temperature
