Chapter 15: Acid-Based Equilibria

15.6 Buffers

Learning Outcomes

  • Describe the composition and function of acid–base buffers
  • Calculate the pH of a buffer before and after the addition of added acid or base

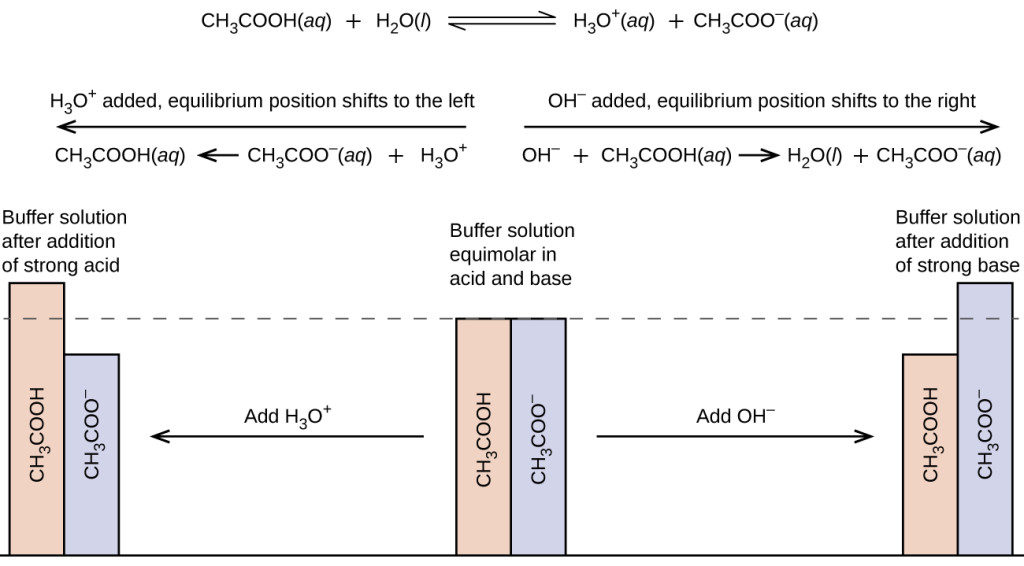
A solution containing appreciable amounts of a weak conjugate acid-base pair is called a buffer solution, or a buffer. Buffer solutions resist a change in pH when small amounts of a strong acid or a strong base are added (Figure 15.6.1). A solution of acetic acid and sodium acetate (CH3COOH + CH3COONa) is an example of a buffer that consists of a weak acid and its salt. An example of a buffer that consists of a weak base and its salt is a solution of ammonia and ammonium chloride (NH3(aq) + NH4Cl(aq)).

Two images are shown. Image a on the left shows two beakers that each contain yellow solutions. The beaker on the left is labeled “Unbuffered” and the beaker on the right is labeled “p H equals 8.0 buffer.” Image b similarly shows 2 beakers. The beaker on the left contains a bright orange solution and is labeled “Unbuffered.” The beaker on the right is labeled “p H equals 8.0 buffer.”
Figure 15.6.1. (a) The unbuffered solution on the left and the buffered solution on the right have the same pH (pH 8); they are basic, showing the yellow color of the indicator methyl orange at this pH. (b) After the addition of 1 mL of a 0.01-M HCl solution, the buffered solution has not detectably changed its pH but the unbuffered solution has become acidic, as indicated by the change in color of the methyl orange, which turns red at a pH of about 4. (credit: modification of work by Mark Ott)

How Buffers Work

To illustrate the function of a buffer solution, consider a mixture of roughly equal amounts of acetic acid and sodium acetate. The presence of a weak conjugate acid-base pair in the solution imparts the ability to neutralize modest amounts of added strong acid or base. For example, adding strong base to this solution will neutralize hydronium ion and shift the acetic acid ionization equilibrium to the right, partially restoring the decreased H3O+ concentration:

[latex]{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\leftrightharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\left(aq\right)[/latex]

Likewise, adding strong acid to this buffer solution will neutralize acetate ion, shifting the above ionization equilibrium right and returning [H3O+] to near its original value. Figure 15.6.2 provides a graphical illustration of the changes in conjugate-partner concentration that occur in this buffer solution when strong acid and base are added. The buffering action of the solution is essentially a result of the added strong acid and base being converted to the weak acid and base that make up the buffer’s conjugate pair. The weaker acid and base undergo only slight ionization, as compared with the complete ionization of the strong acid and base, and the solution pH, therefore, changes much less drastically than it would in an unbuffered solution.

This figure begins with a chemical reaction at the top: C H subscript 3 C O O H ( a q ) plus H subscript 2 O ( l ) equilibrium arrow H subscript 3 O superscript positive sign ( a q ) plus C H subscript 3 C O O superscript negative sign ( a q ). Below this equation are two arrows: one pointing left and other pointing right. The arrow pointing left has this phrase written above it, “H subscript 3 O superscript positive sign added, equilibrium position shifts to the left.” Below the arrow is the reaction: C H subscript 3 C O O H ( a q ) left-facing arrow C H subscript 3 C O O superscript negative sign ( a q ) plus H subscript 3 O superscript positive sign. The arrow pointing right has this phrase written above it, “O H subscript negative sign added, equilibrium position shifts to the right.” Below the arrow is the reaction: O H superscript negative sign plus C H subscript 3 C O O H ( a q ) right-facing arrow H subscript 2 O ( l ) plus C H subscript 3 C O O superscript negative sign ( a q ). Below all the text is a figure that resembles a bar graph. In the middle are two bars of equal height. One is labeled, “C H subscript 3 C O O H,” and the other is labeled, “C H subscript 3 C O O superscript negative sign.” There is a dotted line at the same height of the bars which extends to the left and right. Above these two bars is the phrase, “Buffer solution equimolar in acid and base.” There is an arrow pointing to the right which is labeled, “Add O H superscript negative sign.” The arrow points to two bars again, but this time the C H subscript 3 C O O H bar is shorter than that C H subscript 3 C O O superscript negative sign bar. Above these two bars is the phrase, “Buffer solution after addition of strong base.” From the middle bars again, there is an arrow that points left. The arrow is labeled, “Add H subscript 3 O superscript positive sign.” This arrow points to two bars again, but this time the C H subscript 3 C O O H bar is taller than the C H subscript 3 C O O superscript negative sign bar. These two bars are labeled, “Buffer solution after addition of strong acid.”
Figure 15.6.2. Buffering action in a mixture of acetic acid and acetate salt.

Example 15.6.1: pH Changes in Buffered and Unbuffered Solutions

Acetate buffers are used in biochemical studies of enzymes and other chemical components of cells to prevent pH changes that might affect the biochemical activity of these compounds.

  1. Calculate the pH of an acetate buffer that is a mixture with 0.10 M acetic acid and 0.10 M sodium acetate.
  2. Calculate the pH after 1.0 mL of 0.10 NaOH is added to 100 mL of this buffer.
  3. For comparison, calculate the pH after 1.0 mL of 0.10 M NaOH is added to 100 mL of a solution of an unbuffered solution with a pH of 4.74.
Show Solution

(a) To determine the pH of the buffer solution we use a typical equilibrium calculation (as illustrated in earlier Examples):

Four tan rectangles are shown that are connected with right pointing arrows. The first is labeled “Determine the direction of change.” The second is labeled “Determine x and the equilibrium concentrations.” The third is labeled “Solve for x and the equilibrium concentrations.” The fourth is labeled “Check the math.”

Step 1. Determine the direction of change.

[latex]{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\left(aq\right)[/latex]

We look it up in Ionization Constants of Weak Acids: Ka = 1.8 [latex]\times[/latex] 10−5.

With [CH3CO2H] = [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] = 0.10 M and [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = ~0 M, the reaction shifts to the right to form [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex].

Step 2. Determine x and equilibrium concentrations. A table of changes and concentrations follows:
This table has two main columns and four rows. The first row for the first column does not have a heading and then has the following in the first column: Initial concentration ( M ), Change ( M ), Equilibrium concentration ( M ). The second column has the header of “[ C H subscript 3 C O subscript 2 H ] [ H subscript 2 O ] equilibrium arrow H subscript 3 O superscript plus sign [ C H subscript 3 C O subscript 2 superscript negative sign ].” Under the second column is a subgroup of four columns and three rows. The first column has the following: 0.10, negative x, 0.10 minus sign x. The second column is blank. The third column has the following: approximately 0, positive x, x. The fourth column has the following: 0.10, positive x, 0.10 plus sign x.

Step 3. Solve for x and the equilibrium concentrations.

[latex]x=1.8\times {10}^{-5}M[/latex]

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=0+x=1.8\times {10}^{-5}M[/latex]

[latex]\text{pH}=-\text{log}\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=-\text{log}\left(1.8\times {10}^{-5}\right)[/latex]

[latex]=4.74[/latex]

Step 4. Check the work. If we calculate all calculated equilibrium concentrations, we find that the equilibrium value of the reaction coefficient, Q = Ka.

(b) Adding strong acid will neutralize some of the acetic acid, yielding the conjugate base acetate ion. Compute the new concentrations of these two buffer components, then repeat the equilibrium calculation of part (a) using these new concentrations.Eight tan rectangles are shown in four columns of two rectangles each that are connected with right pointing arrows. The first rectangle in the upper left is labeled “Volume of N a O H solution.” An arrow points right to a second rectangle labeled “Moles of N a O H added.” A second arrow points right to a third rectangle labeled “Additional moles of N a C H subscript 3 C O subscript 2.” Just beneath the first rectangle in the upper left is a rectangle labeled “Volume of buffer solution.” An arrow points right to another rectangle labeled “Initial moles of C H subscript 3 C O subscript 2 H.” This rectangle points to the same third rectangle, which is labeled “ Additional moles of N a C H subscript 3 C O subscript 2.” An arrow points right to a rectangle labeled “ Unreacted moles of C H subscript 3 C O subscript 2 H.” An arrow points from this rectangle to a rectangle below labeled “[ C H subscript 3 C O subscript 2 H ].” An arrow extends below the “Additional moles of N a C H subscript 3 C O subscript 2” rectangle to a rectangle labeled “[ C H subscript 3 C O subscript 2 ].” This rectangle points right to the rectangle labeled “[ C H subscript 3 C O subscript 2 H ].”

Step 1. Determine the moles of NaOH. One milliliter (0.0010 L) of 0.10 M NaOH contains

[latex]0.0010\cancel{\text{L}}\times \left(\dfrac{0.10\text{mol NaOH}}{1\cancel{\text{L}}}\right)=1.0\times {10}^{-4}\text{mol NaOH}[/latex]

Step 2. Determine the moles of CH2CO2H. Before reaction, 0.100 L of the buffer solution contains

[latex]0.100\cancel{\text{L}}\times \left(\dfrac{0.100\text{mol}{\text{ CH}}_{3}{\text{CO}}_{2}\text{H}}{1\cancel{\text{L}}}\right)=1.00\times {10}^{-2}\text{mol}{\text{CH}}_{3}{\text{CO}}_{2}\text{H}[/latex]

Step 3. Solve for the amount of NaCH3CO2 produced. The 1.0 [latex]\times[/latex] 10−4 mol of NaOH neutralizes 1.0 [latex]\times[/latex] 10−4 mol of CH3CO2H, leaving

[latex]\left(1.0\times {10}^{-2}\right)-\left(0.01\times {10}^{-2}\right)=0.99\times {10}^{-2}\text{mol}{\text{CH}}_{3}{\text{CO}}_{2}\text{H}[/latex]

and producing 1.0 [latex]\times[/latex] 10−4 mol of NaCH3CO2. This makes a total of

[latex]\left(1.0\times {10}^{-2}\right)+\left(0.01\times {10}^{-2}\right)=1.01\times {10}^{-2}\text{mol}{\text{NaCH}}_{3}{\text{CO}}_{2}[/latex]

Step 4. Find the molarity of the products. After reaction, CH3CO2H and NaCH3CO2 are contained in 101 mL of the intermediate solution, so:

[latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\right]=\dfrac{9.9\times {10}^{-3}\text{mol}}{0.101\text{L}}=0.098M[/latex]

[latex]\left[{\text{NaCH}}_{3}{\text{CO}}_{2}\right]=\dfrac{1.01\times {10}^{-2}\text{mol}}{0.101\text{L}}=0.100M[/latex]

Now we calculate the pH after the intermediate solution, which is 0.098 M in CH3CO2H and 0.100 M in NaCH3CO2, comes to equilibrium. The calculation is very similar to that in part (a) of this example:
Four tan rectangles are shown that are connected with right pointing arrows. The first is labeled “Determine the direction of change.” The second is labeled “Determine x and the equilibrium concentrations.” The third is labeled “Solve for x and the equilibrium concentrations.” The fourth is labeled “Check the math.”This series of calculations gives a pH = 4.75. Thus the addition of the base barely changes the pH of the solution (F).

(c) This 1.8 [latex]\times[/latex] 10−5–M solution of HCl has the same hydronium ion concentration as the 0.10-M solution of acetic acid-sodium acetate buffer described in part (a) of this example. The solution contains:

[latex]0.100\text{L}\times \left(\dfrac{1.8\times {10}^{-5}\text{mol HCl}}{1\text{L}}\right)=1.8\times {10}^{-6}\text{mol HCl}[/latex]

As shown in part (b), 1 mL of 0.10 M NaOH contains 1.0 [latex]\times[/latex] 10−4 mol of NaOH. When the NaOH and HCl solutions are mixed, the HCl is the limiting reagent in the reaction. All of the HCl reacts, and the amount of NaOH that remains is:

[latex]\left(1.0\times {10}^{-4}\right)-\left(1.8\times {10}^{-6}\right)=9.8\times {10}^{-5}M[/latex]

  • The concentration of NaOH is: [latex]\dfrac{9.8\times {10}^{-5}M\text{NaOH}}{0.101\text{L}}=9.7\times {10}^{-4}M[/latex]
  • The pOH of this solution is: [latex]\text{pOH}=-\text{log}\left[{\text{OH}}^{-}\right]=-\text{log}\left(9.7\times {10}^{-4}\right)=3.01[/latex]
  • The pH is: [latex]\text{pH}=14.00-\text{pOH}=10.99[/latex]

The pH changes from 4.74 to 10.99 in this unbuffered solution. This compares to the change of 4.74 to 4.75 that occurred when the same amount of NaOH was added to the buffered solution described in part (b).

Check Your Learning

If we add an acid or a base to a buffer that is a mixture of a weak base and its salt, the calculations of the changes in pH are analogous to those for a buffer mixture of a weak acid and its salt.

Buffer Capacity

Buffer solutions do not have an unlimited capacity to keep the pH relatively constant (Figure 15.6.3). If we add so much base to a buffer that the weak acid is exhausted, no more buffering action toward the base is possible. On the other hand, if we add an excess of acid, the weak base would be exhausted, and no more buffering action toward any additional acid would be possible. In fact, we do not even need to exhaust all of the acid or base in a buffer to overwhelm it; its buffering action will diminish rapidly as a given component nears depletion.

Figure 3. The indicator color (methyl orange) shows that a small amount of acid added to a buffered solution of pH 8 (beaker on the left) has little affect on the buffered system (middle beaker). However, a large amount of acid exhausts the buffering capacity of the solution and the pH changes dramatically (beaker on the right). (credit: modification of work by Mark Ott)
Figure 15.6.3. The indicator color (methyl orange) shows that a small amount of acid added to a buffered solution of pH 8 (beaker on the left) has little affect on the buffered system (middle beaker). However, a large amount of acid exhausts the buffering capacity of the solution and the pH changes dramatically (beaker on the right). (credit: modification of work by Mark Ott)

The buffer capacity is the amount of acid or base that can be added to a given volume of a buffer solution before the pH changes significantly, usually by one unit. Buffer capacity depends on the amounts of the weak acid and its conjugate base that are in a buffer mixture. For example, 1 L of a solution that is 1.0 M in acetic acid and 1.0 M in sodium acetate has a greater buffer capacity than 1 L of a solution that is 0.10 M in acetic acid and 0.10 M in sodium acetate even though both solutions have the same pH. The first solution has more buffer capacity because it contains more acetic acid and acetate ion.

Selection of Suitable Buffer Mixtures

There are two useful rules of thumb for selecting buffer mixtures:

  1. A good buffer mixture should have about equal concentrations of both of its components. A buffer solution has generally lost its usefulness when one component of the buffer pair is less than about 10% of the other. Figure 15.6.4 shows an acetic acid-acetate ion buffer as base is added. The initial pH is 4.74. A change of 1 pH unit occurs when the acetic acid concentration is reduced to 11% of the acetate ion concentration.

    A graph is shown with a horizontal axis labeled “Added m L of 0.10 M N a O H” which has markings and vertical gridlines every 10 units from 0 to 110. The vertical axis is labeled “p H” and is marked every 1 unit beginning at 0 extending to 11. A break is shown in the vertical axis between 0 and 4. A red curve is drawn on the graph which increases gradually from the point (0, 4.8) up to about (100, 7) after which the graph has a vertical section up to about (100, 11). The curve is labeled [ C H subscript 3 C O subscript 2 H ] is 11 percent of [ C H subscript 3 CO subscript 2 superscript negative].
    Figure 15.6.4. The graph, an illustration of buffering action, shows change of pH as an increasing amount of a 0.10-M NaOH solution is added to 100 mL of a buffer solution in which, initially, [CH3CO2H] = 0.10 M and [CH3CO2−]=0.10M. Note the greatly diminished buffering action occurring after the buffer capacity has been reached, resulting in drastic rises in pH on adding more strong base.
  2. Weak acids and their salts are better as buffers for pHs less than 7; weak bases and their salts are better as buffers for pHs greater than 7.

Blood is an important example of a buffered solution, with the principal acid and ion responsible for the buffering action being carbonic acid, H2CO3, and the bicarbonate ion, [latex]{\text{HCO}}_{3}{}^{-}[/latex]. When an excess of hydrogen ion enters the blood stream, it is removed primarily by the reaction:

[latex]{\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{HCO}}_{3}{}^{-}\left(aq\right)\longrightarrow {\text{H}}_{2}{\text{CO}}_{3}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)[/latex]

When an excess of the hydroxide ion is present, it is removed by the reaction:

[latex]{\text{OH}}^{-}\left(aq\right)+{\text{H}}_{2}{\text{CO}}_{3}\left(aq\right)\longrightarrow {\text{HCO}}_{3}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)[/latex]

The added strong acid or base is thus effectively converted to the much weaker acid or base of the buffer pair (H3O+ is converted to H2CO3 and OH– is converted to HCO3–). The pH of human blood thus remains very near the value determined by the buffer pairs pKa, in this case, 7.35. Normal variations in blood pH are usually less than 0.1, and pH changes of 0.4 or greater are likely to be fatal.

The Henderson-Hasselbalch Equation

Lawrence Joseph Henderson and Karl Albert Hasselbalch

Lawrence Joseph Henderson (1878–1942) was an American physician, biochemist and physiologist, to name only a few of his many pursuits. He obtained a medical degree from Harvard and then spent 2 years studying in Strasbourg, then a part of Germany, before returning to take a lecturer position at Harvard. He eventually became a professor at Harvard and worked there his entire life. He discovered that the acid-base balance in human blood is regulated by a buffer system formed by the dissolved carbon dioxide in blood. He wrote an equation in 1908 to describe the carbonic acid-carbonate buffer system in blood. Henderson was broadly knowledgeable; in addition to his important research on the physiology of blood, he also wrote on the adaptations of organisms and their fit with their environments, on sociology and on university education. He also founded the Fatigue Laboratory, at the Harvard Business School, which examined human physiology with specific focus on work in industry, exercise, and nutrition.

In 1916, Karl Albert Hasselbalch (1874–1962), a Danish physician and chemist, shared authorship in a paper with Christian Bohr in 1904 that described the Bohr effect, which showed that the ability of hemoglobin in the blood to bind with oxygen was inversely related to the acidity of the blood and the concentration of carbon dioxide. The pH scale was introduced in 1909 by another Dane, Sørensen, and in 1912, Hasselbalch published measurements of the pH of blood. In 1916, Hasselbalch expressed Henderson’s equation in logarithmic terms, consistent with the logarithmic scale of pH, and thus the Henderson-Hasselbalch equation was born.

The ionization-constant expression for a solution of a weak acid can be written as:

[latex]{K}_{\text{a}}=\dfrac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{A}}^{-}\right]}{\text{[HA]}}[/latex]

Rearranging to solve for [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex], we get:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]={K}_{\text{a}}\times \dfrac{\text{[HA]}}{\left[{\text{A}}^{-}\right]}[/latex]

Taking the negative logarithm of both sides of this equation, we arrive at:

[latex]-\text{log}\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=-\text{log}{K}_{\text{a}}\text{- log}\dfrac{\left[\text{HA}\right]}{\left[{\text{A}}^{-}\right]}[/latex],

which can be written as

[latex]\text{pH}=\text{p}{K}_{\text{a}}+\text{log}\dfrac{\left[{\text{A}}^{-}\right]}{\left[\text{HA}\right]}[/latex]

where pKa is the negative of the common logarithm of the ionization constant of the weak acid (pKa = −log Ka). This equation relates the pH, the ionization constant of a weak acid, and the concentrations of the weak acid and its salt in a buffered solution. Scientists often use this expression, called the Henderson-Hasselbalch equation, to calculate the pH of buffer solutions. It is important to note that the “x is small” assumption must be valid to use this equation.

Medicine: The Buffer System in Blood

The normal pH of human blood is about 7.4. The carbonate buffer system in the blood uses the following equilibrium reaction:

[latex]{\text{CO}}_{2}\left(g\right)+2{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{2}{\text{CO}}_{3}\left(aq\right)\rightleftharpoons {\text{HCO}}_{3}{}^{-}\left(aq\right)+{\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)[/latex]

The concentration of carbonic acid, H2CO3 is approximately 0.0012 M, and the concentration of the hydrogen carbonate ion, [latex]{\text{HCO}}_{3}{}^{-}[/latex], is around 0.024 M. Using the Henderson-Hasselbalch equation and the pKa of carbonic acid at body temperature, we can calculate the pH of blood:

[latex]\text{pH}=\text{p}{K}_{\text{a}}+\text{log}\dfrac{\left[\text{base}\right]}{\left[\text{acid}\right]}=6.1+\text{log}\dfrac{0.024}{0.0012}=7.4[/latex]

The fact that the H2CO3 concentration is significantly lower than that of the [latex]{\text{HCO}}_{3}{}^{-}[/latex] ion may seem unusual, but this imbalance is due to the fact that most of the by-products of our metabolism that enter our bloodstream are acidic. Therefore, there must be a larger proportion of base than acid, so that the capacity of the buffer will not be exceeded.

Lactic acid is produced in our muscles when we exercise. As the lactic acid enters the bloodstream, it is neutralized by the [latex]{\text{HCO}}_{3}{}^{-}[/latex] ion, producing H2CO3. An enzyme then accelerates the breakdown of the excess carbonic acid to carbon dioxide and water, which can be eliminated by breathing. In fact, in addition to the regulating effects of the carbonate buffering system on the pH of blood, the body uses breathing to regulate blood pH. If the pH of the blood decreases too far, an increase in breathing removes CO2 from the blood through the lungs driving the equilibrium reaction such that [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] is lowered. If the blood is too alkaline, a lower breath rate increases CO2 concentration in the blood, driving the equilibrium reaction the other way, increasing [H+] and restoring an appropriate pH.

Key Concepts and Summary

Solutions that contain appreciable amounts of a weak conjugate acid-base pair are called buffers. A buffered solution will experience only slight changes in pH when small amounts of acid or base are added. Addition of large amounts of acid or base can exceed the buffer capacity, consuming most of one conjugate partner and preventing further buffering action.

Key Equations

  • [latex]\text{p}K_{\text{a}}=−\text{log}K_{\text{a}}[/latex]
  • [latex]\text{p}K_{\text{b}}=−\text{log}K_{\text{b}}[/latex]
  • [latex]\text{pH}=\text{p}{K}_{\text{a}}+\text{log}\dfrac{\left[{\text{A}}^{-}\right]}{\left[\text{HA}\right]}[/latex]

Try It

  1. Explain why the pH does not change significantly when a small amount of an acid or a base is added to a solution that contains equal amounts of the acid H3PO4 and a salt of its conjugate base NaH2PO4.
  2. What is [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] in a solution of 0.25 M CH3CO2H and 0.030 M NaCH3CO2?
    [latex]{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\left(aq\right){K}_{\text{a}}=1.8\times {10}^{-5}[/latex]
  3. What is [OH−] in a solution of 0.125 M CH3NH2 and 0.130 M CH3NH3Cl?
    [latex]{\text{CH}}_{3}{\text{NH}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{CH}}_{3}{\text{NH}}_{3}{}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right){K}_{\text{b}}=4.4\times {10}^{-4}[/latex]
  4. What is the effect on the concentration of acetic acid, hydronium ion, and acetate ion when the following are added to an acidic buffer solution of equal concentrations of acetic acid and sodium acetate:
    1. HCl
    2. KCH3CO2
    3. NaCl
    4. KOH
    5. CH3CO2H
Show Solutions

1. Excess [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] is removed primarily by the reaction
[latex]{\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{H}}_{2}{\text{PO}}_{4}{}^{-}\left(aq\right)\longrightarrow {\text{H}}_{3}{\text{PO}}_{4}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)[/latex]

Excess base is removed by the reaction [latex]{\text{OH}}^{-}\left(aq\right)+{\text{H}}_{3}{\text{PO}}_{4}\left(aq\right)\longrightarrow {\text{H}}_{2}{\text{PO}}_{4}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)[/latex]

2. The equilibrium expression is [latex]{K}_{\text{a}}=\frac{\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\right]}=1.8\times {10}^{-5}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

[CH3CO2H] [H3O+] [CH3CO2]
Initial concentration (M) 0.25 0 0.030
Change (M) −x x x
Equilibrium (M) 0.25 − x x 0.030 + x

Substituting the equilibrium concentrations into the equilibrium expression, and making the assumptions that (0.25 − x) ≈ 0.25 and (0.030 − x) ≈ 0.030, gives:

[latex]\frac{\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\right]}=\frac{\left(x\right)\left(0.030-x\right)}{\left(0.25-x\right)}\approx \frac{\left(x\right)\left(0.030\right)}{0.25}=1.8\times {10}^{-5}[/latex]

Solving for x gives 1.50 [latex]\times[/latex] 10−4M. Because this value is less than 5% of both 0.25 and 0.030, our assumptions are correct. Therefore, [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 1.5 [latex]\times[/latex] 10−4M.

This problem can also be solved using the Henderson-Hasselbalch equation: [latex]\text{pH}=\text{p}{K}_{\text{a}}+\text{log}\frac{\left[{\text{A}}^{-}\right]}{\left[\text{HA}\right]}[/latex]; pKa = −log(Ka) = −log(1.8 [latex]\times[/latex] 10−5) = 4.74; [HA] ≈ [HA]0 = [CH3CO2H]0 = 0.25 M; [A−] ≈ [NaCH3CO2] = 0.030 M. Using these data: [latex]\text{pH}=4.74-\text{log}\left(\frac{0.030M}{0.25M}\right)=3.82[/latex]; [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 10−pHM = 10−3.82M = 1.5 [latex]\times[/latex] 10−4M

3. The equilibrium expression is: [latex]{K}_{\text{b}}=\frac{\left[{\text{CH}}_{3}{\text{NH}}_{3}{}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{CH}}_{3}{\text{NH}}_{2}\right]}=4.4\times {10}^{-4}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

[CH3NH2] [CH3NH3+] [OH−]
Initial concentration (M) 0.125 0.130 0
Change (M) −x x x
Equilibrium (M) 0.125 − x 0.130 + x x

Substituting the equilibrium concentrations into the equilibrium expression, and making the assumptions that (0.125 − x) ≈ 0.125 and (0.130 − x) ≈ 0.130, gives:

[latex]\frac{\left[{\text{CH}}_{3}{\text{NH}}_{3}{}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{CH}}_{3}{\text{NH}}_{2}\right]}=\frac{\left(0.130-x\right)\left(x\right)}{\left(0.125-x\right)}\approx \frac{\left(0.130\right)\left(x\right)}{0.125}=4.4\times {10}^{-4}[/latex]

Solving for x gives 4.23 [latex]\times[/latex] 10−4M. Because this value is less than 5% of both 0.125 and 0.130, our assumptions are correct. Therefore, [OH−] = 4.2 [latex]\times[/latex] 10−4M.

4. The reaction and equilibrium constant are
[latex]{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\left(aq\right)\,\,\,\,\,\,\,\,\,\,\,{K}_{\text{a}}=1.8\times {10}^{-5}[/latex]

  1. (a) The added HCl will increase the concentration of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] slightly, which will react with [latex]{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}[/latex] and produce CH3CO2H in the process. Thus, [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] decreases and [CH3CO2H] increases.
  2. (b) The added KCH3CO2 will increase the concentration of [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] which will react with [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] and produce CH3CO2 H in the process. Thus, [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] decreases slightly and [CH3CO2H] increases.
  3. (c) The added NaCl will have no effect on the concentration of the ions.
  4. (d) The added KOH will produce OH− ions, which will react with the [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex], thus reducing [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex]. Some additional CH3CO2H will dissociate, producing [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] ions in the process. Thus, [CH3CO2H] decreases slightly and [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] increases.
  5. (e) The added CH3CO2H will increase its concentration, causing more of it to dissociate and producing more [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] and [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] in the process. Thus, [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] increases slightly and [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] increases.

Glossary

buffer capacity: amount of an acid or base that can be added to a volume of a buffer solution before its pH changes significantly (usually by one pH unit)

buffer: mixture of a weak acid or a weak base and the salt of its conjugate; the pH of a buffer resists change when small amounts of acid or base are added

Henderson-Hasselbalch equation: equation used to calculate the pH of buffer solutions

Licenses and Attributions (Click to expand)

CC licensed content, Shared previously

  • Chemistry 2e. Provided by: OpenStax. Located at: https://openstax.org/. License: CC BY: Attribution. License Terms: Access for free at
    https://openstax.org/books/chemistry-2e/pages/1-introduction
definition

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chemistry Fundamentals Copyright © by Dr. Julie Donnelly, Dr. Nicole Lapeyrouse, and Dr. Matthew Rex is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book