9.5 Stoichiometry of Reactions Involving Gases

A Collective Work

Chapter 9: Gases

9.5 Stoichiometry of Reactions Involving Gases

Learning Outcomes

Use balanced chemical equations to relate the volumes of gas-phase reactants and products using the stoichiometry of the reaction and the ideal gas law

Chemical Stoichiometry and Gases

Chemical stoichiometry describes the quantitative relationships between reactants and products in chemical reactions.

We have previously measured quantities of reactants and products using masses for solids and volumes in conjunction with the molarity for solutions; now we can also use gas volumes to indicate quantities. If we know the volume, pressure, and temperature of a gas, we can use the ideal gas equation to calculate how many moles of the gas are present. If we know how many moles of a gas are involved, we can calculate the volume of a gas at any temperature and pressure.

Avogadro’s Law Revisited

Sometimes we can take advantage of a simplifying feature of the stoichiometry of gases that solids and solutions do not exhibit: All gases that show ideal behavior contain the same number of molecules in the same volume (at the same temperature and pressure). Thus, the ratios of volumes of gases involved in a chemical reaction are given by the coefficients in the equation for the reaction, provided that the gas volumes are measured at the same temperature and pressure.

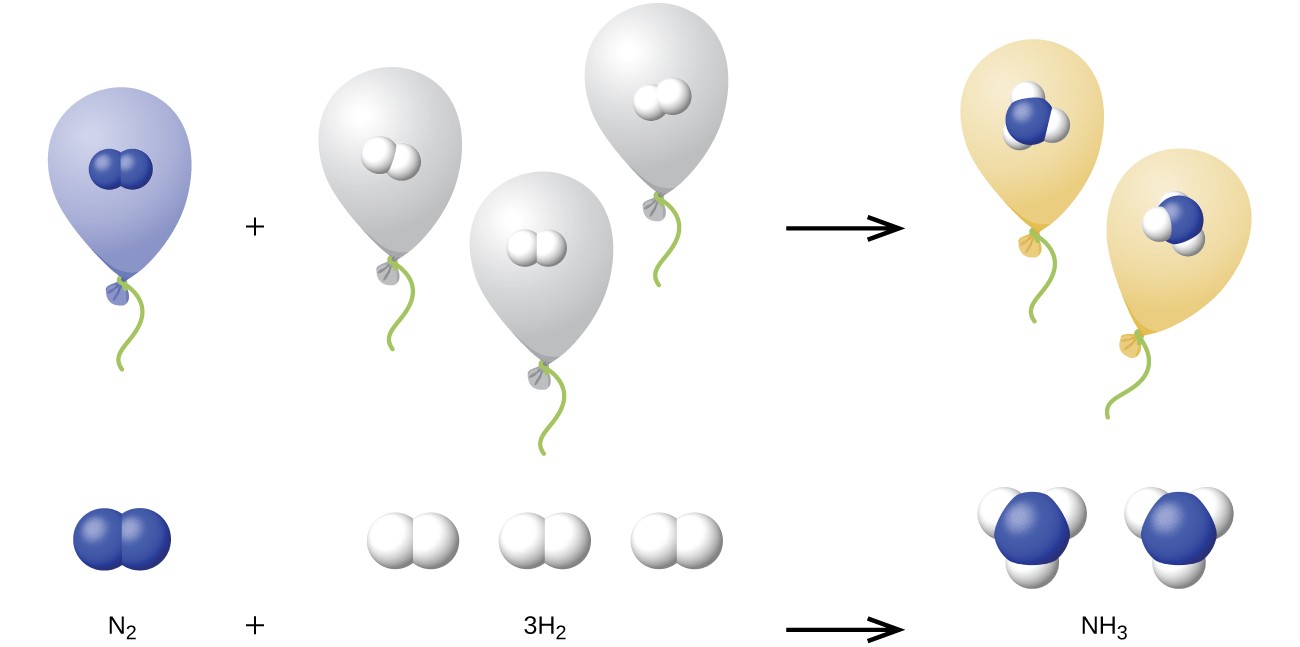
We can extend Avogadro’s law (that the volume of a gas is directly proportional to the number of moles of the gas) to chemical reactions with gases: Gases combine, or react, in definite and simple proportions by volume, provided that all gas volumes are measured at the same temperature and pressure. For example, since nitrogen and hydrogen gases react to produce ammonia gas according to $N_{2} (g) + 3 H_{2} (g) \to {2 NH}_{3} (g)$ , a given volume of nitrogen gas reacts with three times that volume of hydrogen gas to produce two times that volume of ammonia gas, if pressure and temperature remain constant.

The explanation for this is illustrated in Figure 9.5.1. According to Avogadro’s law, equal volumes of gaseous $N_{2}$ , $H_{2}$ , and $NH_{3}$ , at the same temperature and pressure, contain the same number of molecules. Because one molecule of $N_{2}$ reacts with three molecules of $H_{2}$ to produce two molecules of $NH_{3}$ , the volume of $H_{2}$ required is three times the volume of $N_{2}$ , and the volume of $NH_{3}$ produced is two times the volume of $N_{2}$ .

This diagram provided models of the chemical reaction written with formulas across the bottom of the figure. The reaction is written; N subscript 2 plus 3H subscript 2 followed by an arrow pointing right to NH subscript 3. Just above the formulas, space-filling models are provided. Above NH subscript 2, two blue spheres are bonded. Above 3H subscript 2, three pairs of two slightly smaller white spheres are bonded. Above NH subscript 3, two molecules are shown composed each of a central blue sphere to which three slightly smaller white spheres are bonded. Across the top of the diagram, the reaction is illustrated with balloons. To the left is a light blue balloon, which is labeled “N subscript 2”. This balloon contains a single space-filling model composed of two bonded blue spheres. This balloon is followed by a plus sign, then three grey balloons which are each labeled “H subscript 2.” Each of these balloons similarly contain a single space-filling model composed of two bonded white spheres. These white spheres are slightly smaller than the blue spheres. An arrow follows that points right to two light-green balloons, which are each labeled “2 NH subscript 3.” Each light-green balloon contains a space-filling model composed of a single central blue sphere to which three slightly smaller white spheres are bonded. This diagram provided models of the chemical reaction written with formulas across the bottom of the figure. The reaction is written; N subscript 2 plus 3H subscript 2 followed by an arrow pointing right to NH subscript 3. Just above the formulas, space-filling models are provided. Above NH subscript 2, two blue spheres are bonded. Above 3H subscript 2, three pairs of two slightly smaller white spheres are bonded. Above NH subscript 3, two molecules are shown composed each of a central blue sphere to which three slightly smaller white spheres are bonded. Across the top of the diagram, the reaction is illustrated with balloons. To the left is a light blue balloon, which is labeled “N subscript 2”. This balloon contains a single space-filling model composed of two bonded blue spheres. This balloon is followed by a plus sign, then three grey balloons which are each labeled “H subscript 2.” Each of these balloons similarly contain a single space-filling model composed of two bonded white spheres. These white spheres are slightly smaller than the blue spheres. An arrow follows that points right to two light-green balloons, which are each labeled “2 NH subscript 3.” Each light-green balloon contains a space-filling model composed of a single central blue sphere to which three slightly smaller white spheres are bonded. — Figure 9.5.1. One volume of $N_{2}$ combines with three volumes of $H_{2}$ to form two volumes of $NH_{3}$ .

Example 9.5.1: Reaction of Gases

Propane, $C_{3} H_{8}$ (g), is used in gas grills to provide the heat for cooking. What volume of $O_{2}$ (g) measured at 25 °C and 760 torr is required to react with 2.7 L of propane measured under the same conditions of temperature and pressure? Assume that the propane undergoes complete combustion.

Show Solution

The ratio of the volumes of $C_{3} H_{8}$ and $O_{2}$ will be equal to the ratio of their coefficients in the balanced equation for the reaction:

$\begin{array}{ccccccc} C_{3} H_{8} (g) & + & 5 O_{2} (g) & ⟶ & 3 {CO}_{2} (g) & + & 4 H_{2} O (l) \\ 1 volume & + & 5 volumes & 3 volumes & + & 4 volumes \end{array}$

From the equation, we see that one volume of $C_{2} H_{8}$ will react with five volumes of $O_{2}$ :

$2.7 L C_{3} H_{8} \times \frac{5 L O_{2}}{1 L C_{3} H_{8}} = 13.5 L O_{2}$

A volume of 13.5 L of $O_{2}$ will be required to react with 2.7 L of $C_{3} H_{8}$ .

Check Your Learning

Example 9.5.2: Volumes of Reacting Gases

Ammonia is an important fertilizer and industrial chemical. Suppose that a volume of 683 billion cubic feet of gaseous ammonia, measured at 25 °C and 1 atm, was manufactured. What volume of $H_{2}$ (g), measured under the same conditions, was required to prepare this amount of ammonia by reaction with $N_{2}$ ?

$N_{2} (g) + 3 H_{2} (g) \to 2 {NH}_{3} (g)$

Show Solution

Because equal volumes of $H_{2}$ and $NH_{3}$ contain equal numbers of molecules and each three molecules of $H_{2}$ that react produce two molecules of $NH_{3}$ , the ratio of the volumes of $H_{2}$ and $NH_{3}$ will be equal to 3:2. Two volumes of $NH_{3}$ , in this case in units of billion ft³, will be formed from three volumes of $H_{2}$ :

$683 billion {ft}^{3} {NH}_{3} \times \frac{3 billion {ft}^{3} H_{2}}{2 billion {ft}^{3} {NH}_{3}} = 1.02 \times 10^{3} billion {ft}^{3} H_{2}$

The manufacture of 683 billion ft³ of $NH_{3}$ required 1020 billion ft³ of $H_{2}$ . (At 25 °C and 1 atm, this is the volume of a cube with an edge length of approximately 1.9 miles.)

Check Your Learning

Example 9.5.3: Volume of Gaseous Product

What volume of hydrogen at 27 °C and 723 torr may be prepared by the reaction of 8.88 g of gallium with an excess of hydrochloric acid?

$2 Ga (s) + 6 HCl (a q) \to 2 {GaCl}_{3} (a q) + 3 H_{2} (g)$

Show Solution

To convert from the mass of gallium to the volume of $H_{2}$ (g), we need to do something like this:

The first two conversions are:

$8.88 g Ga \times \frac{1 mol Ga}{69.723 g Ga} \times \frac{3 mol H_{2}}{2 mol Ga} = 0.191 {mol H}_{2}$

Finally, we can use the ideal gas law:

$V_{H_{2}} = {(\frac{n R T}{P})}_{H_{2}} = \frac{0.191 mol \times 0.08206 L atm {mol}^{- 1} K^{- 1} \times 300 K}{0.951 atm} = 4.94 L$

Check Your Learning

Key Concepts and Summary

Avogadro’s law may be used in stoichiometric computations for chemical reactions involving gaseous reactants or products.

Try It

Joseph Priestley first prepared pure oxygen by heating mercuric oxide, $HgO$ : $2 HgO (s) \to 2 Hg (l) + O_{2} (g)$
1. Outline the steps necessary to answer the following question: What volume of $O_{2}$ at 23° C and 0.975 atm is produced by the decomposition of 5.36 g of $HgO$ ?
2. Answer the question.
Cavendish prepared hydrogen in 1766 by the novel method of passing steam through a red-hot gun barrel: $4 H_{2} O (g) + 3 Fe (s) \to {Fe}_{3} O_{4} (s) + 4 H_{2} (g)$
1. Outline the steps necessary to answer the following question: What volume of $H_{2}$ at a pressure of 745 torr and a temperature of 20 °C can be prepared from the reaction of 15.O g of $H_{2} O$ ?
2. Answer the question.

Selected Answers

The answers are as follows:
1. Determine the moles of $HgO$ that decompose; using the chemical equation, determine the moles of $O_{2}$ produced by decomposition of this amount of $HgO$ ; and determine the volume of $O_{2}$ from the moles of $O_{2}$ , temperature, and pressure.
2. $\begin{array}{l} 5.36 g HgO \times \frac{1 mol HgO}{(200.59 + 15.9994) g HgO} = 0.0247 mol HgO \\ 0.0247 mol HgO \times \frac{1 mol O_{2}}{2 mol HgO} = 0.01235 mol O_{2} \end{array}$

PV = nRT
P = 0.975 atm
T = (23.0 + 273.15) K

$V = \frac{n R T}{P} = \frac{0.01235 mol (0.08206 L atm {mol}^{- 1} K^{- 1}) (296.15 K)}{0.975 atm} = 0.308 L$

Glossary

Dalton’s law of partial pressures: total pressure of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases.

mole fraction: concentration unit defined as the ratio of the molar amount of a mixture component to the total number of moles of all mixture components

partial pressure: pressure exerted by an individual gas in a mixture

vapor pressure of water: pressure exerted by water vapor in equilibrium with liquid water in a closed container at a specific temperature

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Chemistry Fundamentals Copyright © by Dr. Julie Donnelly, Dr. Nicole Lapeyrouse, and Dr. Matthew Rex is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Learning Outcomes

Chemical Stoichiometry and Gases

Avogadro’s Law Revisited

Example 9.5.1: Reaction of Gases

Check Your Learning

Example 9.5.2: Volumes of Reacting Gases

Check Your Learning

Example 9.5.3: Volume of Gaseous Product

Check Your Learning

Key Concepts and Summary

Try It

Glossary

License

Share This Book