14.1 Mutual Inductance

Inductance is the property of a device that tells us how effectively it induces an emf in another device. In other words, it is a physical quantity that expresses the effectiveness of a given device.

When two circuits carrying time-varying currents are close to one another, the magnetic flux through each circuit varies because of the changing current I in the other circuit. Consequently, an emf is induced in each circuit by the changing current in the other. This type of emf is therefore called a mutually induced emf, and the phenomenon that occurs is known as mutual inductance (M). As an example, let’s consider two tightly wound coils (Figure 14.2). Coils 1 and 2 have $N_1$ and $N_2$ turns and carry currents $I_1$ and $I_2,$ respectively. The flux through a single turn of coil 2 produced by the magnetic field of the current in coil 1 is ${\text{Φ}}_{21},$ whereas the flux through a single turn of coil 1 due to the magnetic field of $I_2$ is ${\text{Φ}}_{12}.$

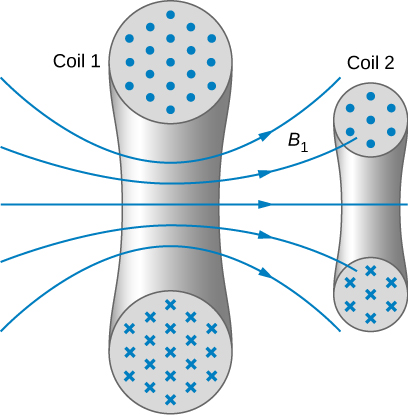
The mutual inductance $M_{21}$ of coil 2 with respect to coil 1 is the ratio of the flux through the $N_2$ turns of coil 2 produced by the magnetic field of the current in coil 1, divided by that current, that is,

Similarly, the mutual inductance of coil 1 with respect to coil 2 is

Like capacitance, mutual inductance is a geometric quantity. It depends on the shapes and relative positions of the two coils, and it is independent of the currents in the coils. The SI unit for mutual inductance M is called the henry (H) in honor of Joseph Henry (1799–1878), an American scientist who discovered induced emf independently of Faraday. Thus, we have $1\phantom{}\text{H}=1\phantom{}\text{V}·\text{s/A}$. From Equation 14.1 and Equation 14.2, we can show that $M_{21}=M_{12},$ so we usually drop the subscripts associated with mutual inductance and write

The emf developed in either coil is found by combining Faraday’s law and the definition of mutual inductance. Since $N_2{\text{Φ}}_{21}$ is the total flux through coil 2 due to $I_1$, we obtain

where we have used the fact that M is a time-independent constant because the geometry is time-independent. Similarly, we have

In Equation 14.5, we can see the significance of the earlier description of mutual inductance (M) as a geometric quantity. The value of M neatly encapsulates the physical properties of circuit elements and allows us to separate the physical layout of the circuit from the dynamic quantities, such as the emf and the current. Equation 14.5 defines the mutual inductance in terms of properties in the circuit, whereas the previous definition of mutual inductance in Equation 14.1 is defined in terms of the magnetic flux experienced, regardless of circuit elements. You should be careful when using Equation 14.4 and Equation 14.5 because ${ϵ}_1\phantom{}\text{and}\phantom{}{ϵ}_2$ do not necessarily represent the total emfs in the respective coils. Each coil can also have an emf induced in it because of its self-inductance (self-inductance will be discussed in more detail in a later section).

A large mutual inductance M may or may not be desirable. We want a transformer to have a large mutual inductance. But an appliance, such as an electric clothes dryer, can induce a dangerous emf on its metal case if the mutual inductance between its coils and the case is large. One way to reduce mutual inductance is to counter-wind coils to cancel the magnetic field produced (Figure 14.3).

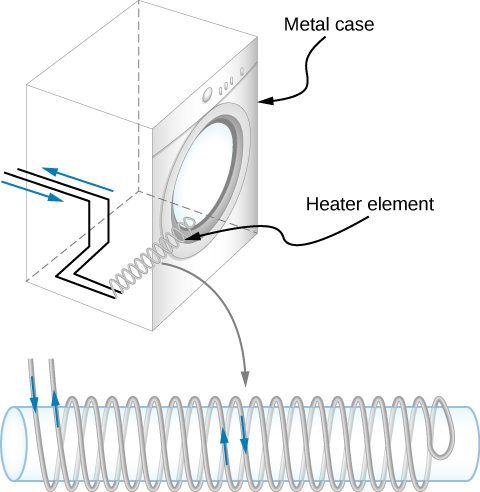
Digital signal processing is another example in which mutual inductance is reduced by counter-winding coils. The rapid on/off emf representing 1s and 0s in a digital circuit creates a complex time-dependent magnetic field. An emf can be generated in neighboring conductors. If that conductor is also carrying a digital signal, the induced emf may be large enough to switch 1s and 0s, with consequences ranging from inconvenient to disastrous.

Example

Mutual Inductance

Figure 14.4 shows a coil of $N_2$ turns and radius $R_2$ surrounding a long solenoid of length $l_1,$ radius $R_1,$ and $N_1$ turns. (a) What is the mutual inductance of the two coils? (b) If $N_1=500\phantom{}\text{turns}$, $N_2=10\phantom{}\text{turns}$, $R_1=3.10\phantom{}\text{cm}$, $l_1=75.0\phantom{}\text{cm}$, and the current in the solenoid is changing at a rate of 200 A/s, what is the emf induced in the surrounding coil?

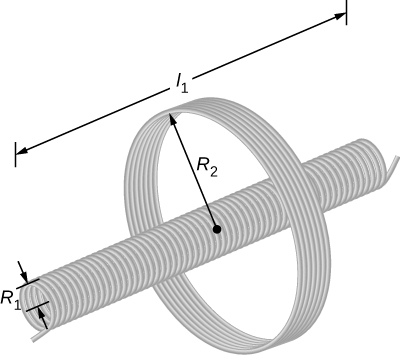
Strategy

There is no magnetic field outside the solenoid, and the field inside has magnitude $B_1={\mu }_0\left(N_1\text{/}{l}_1\right)I_1$ and is directed parallel to the solenoid’s axis. We can use this magnetic field to find the magnetic flux through the surrounding coil and then use this flux to calculate the mutual inductance for part (a), using Equation 14.3. We solve part (b) by calculating the mutual inductance from the given quantities and using Equation 14.4 to calculate the induced emf.

Solution

Show Answer

1. The magnetic flux ${\text{Φ}}_{21}$ through the surrounding coil is
   
   ${\text{Φ}}_{21}=B_1\pi R_1^2=\frac{{\mu }_0{N}_1{I}_1}{l_1}\pi R_1^2.$

   
   Now from Equation 14.3, the mutual inductance is
   

   $M=\frac{N_2{\text{Φ}}_{21}}{I_1}=\left(\frac{N_2}{I_1}\right)\left(\frac{{\mu }_0{N}_1{I}_1}{l_1}\right)\pi R_1^2=\frac{{\mu }_0{N}_1{N}_2\pi R_1^2}{l_1}.$
2. Using the previous expression and the given values, the mutual inductance is
   
   $\begin{array}{cc}\hfill M& =\frac{(4\pi \phantom{}\times \phantom{}{10}^{-7}\phantom{}\text{T}·\text{m/A})\left(500\right)\left(10\right)\pi {\left(0.0310\phantom{}\text{m}\right)}^2}{0.750\phantom{}\text{m}}\hfill \\ & =2.53\phantom{}\times \phantom{}{10}^{-5}\phantom{}\text{H}.\hfill \end{array}$

   
   Thus, from Equation 14.4, the emf induced in the surrounding coil is
   

   $\begin{array}{cc}\hfill {ϵ}_2& =\text{−}M\frac{d{I}_1}{dt}=\text{−}\left(2.53\phantom{}\times \phantom{}{10}^{-5}\phantom{}\text{H}\right)\left(200\phantom{}\text{A/s}\right)\hfill \\ & =-5.06\phantom{}\times \phantom{}{10}^{-3}\phantom{}\text{V}.\hfill \end{array}$

Significance

Notice that M in part (a) is independent of the radius $R_2$ of the surrounding coil because the solenoid’s magnetic field is confined to its interior. In principle, we can also calculate M by finding the magnetic flux through the solenoid produced by the current in the surrounding coil. This approach is much more difficult because ${\text{Φ}}_{12}$ is so complicated. However, since $M_{12}=M_{21},$ we do know the result of this calculation.