5.3 Coulomb’s Law

Samuel J. Ling; William Moebs; Jeff Sanny

Chapter 5. Electric Charges and Fields

5.3 Coulomb’s Law

Learning Objectives

By the end of this section, you will be able to:

Describe the electric force, both qualitatively and quantitatively
Calculate the force that charges exert on each other
Determine the direction of the electric force for different source charges
Correctly describe and apply the superposition principle for multiple source charges

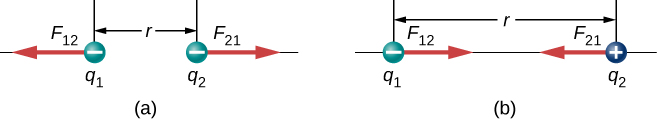
Experiments with electric charges have shown that if two objects each have electric charge, then they exert an electric force on each other. The magnitude of the force is linearly proportional to the net charge on each object and inversely proportional to the square of the distance between them. (Interestingly, the force does not depend on the mass of the objects.) The direction of the force vector is along the imaginary line joining the two objects and is dictated by the signs of the charges involved.

Let

$q_{1}, q_{2} =$ the net electric charges of the two objects;
${\vec{r}}_{12} =$ the vector displacement from $q_{1}$ to $q_{2}$ .

The electric force $\vec{F}$ on one of the charges is proportional to the magnitude of its own charge and the magnitude of the other charge, and is inversely proportional to the square of the distance between them:

F \propto \frac{q_{1} q_{2}}{r_{12}^{2}} .

This proportionality becomes an equality with the introduction of a proportionality constant. For reasons that will become clear in a later chapter, the proportionality constant that we use is actually a collection of constants. (We discuss this constant shortly.)

Coulomb’s Law

The magnitude of the electric force (or Coulomb force) between two electrically charged particles is equal to

| F_{12} | = \frac{1}{4 π ϵ_{0}} \frac{| q_{1} q_{2} |}{r_{12}^{2}}

We use absolute value signs around the product $q_{1} q_{2}$ because one of the charges may be negative, but the magnitude of the force is always positive. The direction of the force vector depends on the sign of the charges. If the charges are the same, the force points away from the other charge. If the charges have different signs, the force points toward the other charge(Figure 5.14).

In part a, two charges q one and q two are shown separated by a distance r. Force vector arrow F one two points toward left and acts on q one. Force vector arrow F two one points toward right and acts on q two. Both forces act in opposite directions and are represented by arrows of same length. In part b, two charges q one and q two are shown at a distance r. Force vector arrow F one two points toward right and acts on q one. Force vector arrow F two one points toward left and acts on q two. Both forces act toward each other and are represented by arrows of same length. — **Figure 5.14** The electrostatic force $\vec{F}$ between point charges $q_{1}$ and $q_{2}$ separated by a distance r is given by Coulomb’s law. Note that Newton’s third law (every force exerted creates an equal and opposite force) applies as usual—the force on $q_{1}$ is equal in magnitude and opposite in direction to the force it exerts on $q_{2}$ . (a) Like charges; (b) unlike charges.

It is important to note that the electric force is not constant; it is a function of the separation distance between the two charges. If either the test charge or the source charge (or both) move, then $\vec{r}$ changes, and therefore so does the force. An immediate consequence of this is that direct application of Newton’s laws with this force can be mathematically difficult, depending on the specific problem at hand. It can (usually) be done, but we almost always look for easier methods of calculating whatever physical quantity we are interested in. (Conservation of energy is the most common choice.)

Finally, the new constant $ϵ_{0}$ in Coulomb’s law is called the permittivity of free space, or (better) the permittivity of vacuum. It has a very important physical meaning that we will discuss in a later chapter; for now, it is simply an empirical proportionality constant. Its numerical value (to three significant figures) turns out to be

ϵ_{0} = 8.85 \times 10^{- 12} \frac{C^{2}}{N \cdot m^{2}} .

These units are required to give the force in Coulomb’s law the correct units of newtons. Note that in Coulomb’s law, the permittivity of vacuum is only part of the proportionality constant. For convenience, we often define a Coulomb’s constant:

k_{e} = \frac{1}{4 π ϵ_{0}} = 8.99 \times 10^{9} \frac{N \cdot m^{2}}{C^{2}} .

Example

The Force on the Electron in Hydrogen

A hydrogen atom consists of a single proton and a single electron. The proton has a charge of $+ e$ and the electron has $− e$ . In the “ground state” of the atom, the electron orbits the proton at most probable distance of $5.29 \times 10^{- 11} m$ (Figure 5.15). Calculate the electric force on the electron due to the proton.

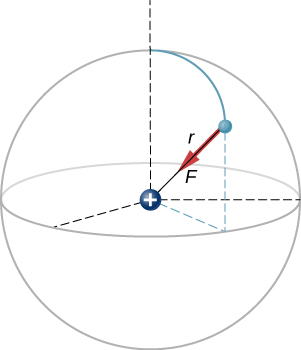
A positive charge is shown at the center of a sphere of radius r. An electron is depicted as a particle on the sphere. The force on the electron is along the radius, toward the nucleus. — **Figure 5.15** A schematic depiction of a hydrogen atom, showing the force on the electron. This depiction is only to enable us to calculate the force; the hydrogen atom does not really look like this. Recall Figure 5.7.

Strategy

For the purposes of this example, we are treating the electron and proton as two point particles, each with an electric charge, and we are told the distance between them; we are asked to calculate the force on the electron. We thus use Coulomb’s law.

Solution

Show Answer

Our two charges and the distance between them are,

\begin{array}{ccc} q_{1} & = & + e = + 1.602 \times 10^{- 19} C \\ q_{2} & = & − e = - 1.602 \times 10^{- 19} C \\ r & = & 5.29 \times 10^{- 11} m . \end{array}

The magnitude of the force on the electron is

F = \frac{1}{4 π ϵ_{0}} \frac{{| e |}^{2}}{r^{2}} = \frac{1}{4 π (8.85 \times 10^{- 12} \frac{C^{2}}{N \cdot m^{2}})} \frac{{(1.602 \times 10^{- 19} C)}^{2}}{{(5.29 \times 10^{- 11} m)}^{2}} = 8.25 \times 10^{- 8} N .

As for the direction, since the charges on the two particles are opposite, the force is attractive; the force on the electron points radially directly toward the proton, everywhere in the electron’s orbit. The force is thus expressed as

\vec{F} = (8.25 \times 10^{- 8} N) \hat{r} .

Significance

This is a three-dimensional system, so the electron (and therefore the force on it) can be anywhere in an imaginary spherical shell around the proton. In this “classical” model of the hydrogen atom, the electrostatic force on the electron points in the inward centripetal direction, thus maintaining the electron’s orbit. But note that the quantum mechanical model of hydrogen (discussed in Quantum Mechanics) is utterly different.

Check Your Understanding

What would be different if the electron also had a positive charge?

Show Solution

The force would point outward.

Multiple Source Charges

The analysis that we have done for two particles can be extended to an arbitrary number of particles; we simply repeat the analysis, two charges at a time. Specifically, we ask the question: Given N charges (which we refer to as source charge), what is the net electric force that they exert on some other point charge (which we call the test charge)? Note that we use these terms because we can think of the test charge being used to test the strength of the force provided by the source charges.

Like all forces that we have seen up to now, the net electric force on our test charge is simply the vector sum of each individual electric force exerted on it by each of the individual test charges. Thus, we can calculate the net force on the test charge Q by calculating the force on it from each source charge, taken one at a time, and then adding all those forces together (as vectors). This ability to simply add up individual forces in this way is referred to as the principle of superposition, and is one of the more important features of the electric force. In mathematical form, this becomes

\vec{F} (r) = \frac{1}{4 π ϵ_{0}} Q \sum_{i = 1}^{N} \frac{q_{i}}{r_{i}^{2}} {\hat{r}}_{i} .

In this expression, Q represents the charge of the particle that is experiencing the electric force $\vec{F}$ , and is located at $\vec{r}$ from the origin; the $q_{i} ’s$ are the N source charges, and the vectors ${\vec{r}}_{i} = r_{i} {\hat{r}}_{i}$ are the displacements from the position of the ith charge to the position of Q. Each of the N unit vectors points directly from its associated source charge toward the test charge. All of this is depicted in Figure 5.16. Please note that there is no physical difference between Q and $q_{i}$ ; the difference in labels is merely to allow clear discussion, with Q being the charge we are determining the force on.

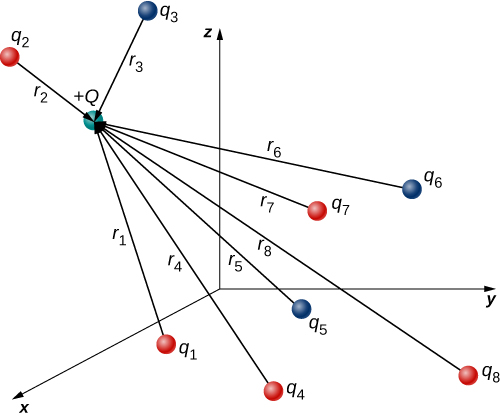
Eight source charges are shown as small spheres distributed within an x y z coordinate system. The sources are labeled q sub 1, q sub 2, and so on. Sources 1, 2, 4, 7 and 8 are shaded red and sources 3, 5, and 6 are shaded blue. A test charge is also shown, shaded in green and labeled as plus Q. The r vectors from each source to the test charge Q are shown as arrows with tails at the sources and heads at the test charge. The vector from q sub 1 to the test charge is labeled as r sub 1. The vector from q sub 2 to the test charge is labeled as r sub 2, and so on for all eight vectors. — **Figure 5.16** The eight source charges each apply a force on the single test charge Q. Each force can be calculated independently of the other seven forces. This is the essence of the superposition principle.

(Note that the force vector ${\vec{F}}_{i}$ does not necessarily point in the same direction as the unit vector ${\hat{r}}_{i}$ ; it may point in the opposite direction, $− {\hat{r}}_{i}$ . The signs of the source charge and test charge determine the direction of the force on the test charge.)

There is a complication, however. Just as the source charges each exert a force on the test charge, so too (by Newton’s third law) does the test charge exert an equal and opposite force on each of the source charges. As a consequence, each source charge would change position. However, by Equation 5.2, the force on the test charge is a function of position; thus, as the positions of the source charges change, the net force on the test charge necessarily changes, which changes the force, which again changes the positions. Thus, the entire mathematical analysis quickly becomes intractable. Later, we will learn techniques for handling this situation, but for now, we make the simplifying assumption that the source charges are fixed in place somehow, so that their positions are constant in time. (The test charge is allowed to move.) With this restriction in place, the analysis of charges is known as electrostatics, where “statics” refers to the constant (that is, static) positions of the source charges and the force is referred to as an electrostatic force.

Example

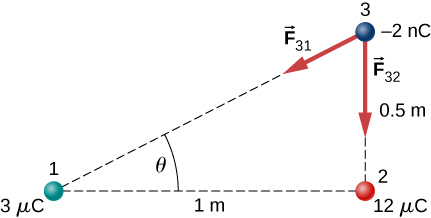
The Net Force from Two Source Charges

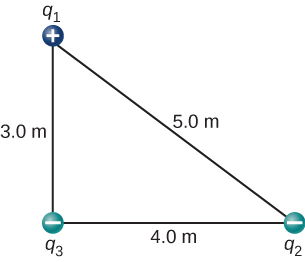
Three different, small charged objects are placed as shown in Figure 5.17. The charges $q_{1}$ and $q_{3}$ are fixed in place; $q_{2}$ is free to move. Given $q_{1} = 2 e$ , $q_{2} = - 3 e$ , and $q_{3} = - 5 e$ , and that $d = 2.0 \times 10^{- 7} m$ , what is the net force on the middle charge $q_{2}$ ?

Three charges are shown in an x y coordinate system. Charge q sub 1 is at x=0, y=d. Charge q sub 2 is at x=2 d, y=0. Charge q sub 3 is at the origin. Force F 1 2 is exerted on charge q sub 2 and points up. Force F 2 3 is exerted on charge q sub 2 and points to the left. Force F is exerted on charge q sub 2 and points at an angle theta above the minus x direction. — **Figure 5.17** Source charges $q_{1}$ and $q_{3}$ each apply a force on $q_{2}$ .

Strategy

We use Coulomb’s law again. The way the question is phrased indicates that $q_{2}$ is our test charge, so that ( $q_{1}$ and $q_{3}$ ) are source charges. The principle of superposition says that the force on $q_{2}$ from each of the other charges is unaffected by the presence of the other charge. Therefore, we write down the force on $q_{2}$ from each and add them together as vectors.

Solution

Show Answer

We have two source charges ( $q_{1}$ and $q_{3}$ ), a test charge $(q_{2}),$ distances $r_{21}$ and $r_{23}$ , and we are asked to find a force. This calls for Coulomb’s law and superposition of forces. There are two forces:

\vec{F} = {\vec{F}}_{21} + {\vec{F}}_{23} = \frac{1}{4 π ϵ_{0}} [\frac{q_{2} q_{1}}{r_{21}^{2}} \hat{j} + (- \frac{q_{2} q_{3}}{r_{23}^{2}} \hat{i})] .

We can’t add these forces directly because they don’t point in the same direction: ${\vec{F}}_{23}$ points only in the −x-direction, while ${\vec{F}}_{21}$ points only in the +y-direction. The net force is obtained from applying the Pythagorean theorem to its x– and y-components:

F = \sqrt{F_{x}^{2} + F_{y}^{2}}

where

\begin{array}{cc} F_{x} & = − F_{23} = - \frac{1}{4 π ϵ_{0}} \frac{q_{2} q_{3}}{r_{23}^{2}} \\ = − (8.99 \times 10^{9} \frac{N \cdot m^{2}}{C^{2}}) \frac{(4.806 \times 10^{- 19} C) (8.01 \times 10^{- 19} C)}{{(4.00 \times 10^{- 7} m)}^{2}} \\ = − 2.16 \times 10^{−14} N \end{array}

and

\begin{array}{cc} F_{y} & = F_{21} = \frac{1}{4 π ϵ_{0}} \frac{q_{2} q_{1}}{r_{21}^{2}} \\ = (8.99 \times 10^{9} \frac{N \cdot m^{2}}{C^{2}}) \frac{(4.806 \times 10^{- 19} C) (3.204 \times 10^{- 19} C)}{{(2.00 \times 10^{- 7} m)}^{2}} \\ = 3.46 \times 10^{- 14} N . \end{array}

We find that

F = \sqrt{F_{x}^{2} + F_{y}^{2}} = 4.08 \times 10^{- 14} N

at an angle of

φ = {tan}^{- 1} (\frac{F_{y}}{F_{x}}) = {tan}^{- 1} (\frac{3.46 \times 10^{- 14} N}{- 2.16 \times 10^{- 14} N}) = - 58 °,

that is, $58 °$ above the −x-axis, as shown in the diagram.

Significance

Notice that when we substituted the numerical values of the charges, we did not include the negative sign of either $q_{2}$ or $q_{3}$ . Recall that negative signs on vector quantities indicate a reversal of direction of the vector in question. But for electric forces, the direction of the force is determined by the types (signs) of both interacting charges; we determine the force directions by considering whether the signs of the two charges are the same or are opposite. If you also include negative signs from negative charges when you substitute numbers, you run the risk of mathematically reversing the direction of the force you are calculating. Thus, the safest thing to do is to calculate just the magnitude of the force, using the absolute values of the charges, and determine the directions physically.

It’s also worth noting that the only new concept in this example is how to calculate the electric forces; everything else (getting the net force from its components, breaking the forces into their components, finding the direction of the net force) is the same as force problems you have done earlier.

Check Your Understanding

What would be different if $q_{1}$ were negative?

Show Solution

The net force would point $58 °$ below the −x-axis.

Summary

Coulomb’s law gives the magnitude of the force between point charges. It is

${\vec{F}}_{12} (r) = \frac{1}{4 π ϵ_{0}} \frac{q_{1} q_{2}}{r_{12}^{2}} {\hat{r}}_{12}$

where $q_{2}$ and $q_{2}$ are two point charges separated by a distance r. This Coulomb force is extremely basic, since most charges are due to point-like particles. It is responsible for all electrostatic effects and underlies most macroscopic forces.

Conceptual Questions

Would defining the charge on an electron to be positive have any effect on Coulomb’s law?

An atomic nucleus contains positively charged protons and uncharged neutrons. Since nuclei do stay together, what must we conclude about the forces between these nuclear particles?

Show Solution

The force holding the nucleus together must be greater than the electrostatic repulsive force on the protons.

Is the force between two fixed charges influenced by the presence of other charges?

Problems

Two point particles with charges $+3 μ C$ and $+5 μ C$ are held in place by 3-N forces on each charge in appropriate directions. (a) Draw a free-body diagram for each particle. (b) Find the distance between the charges.

Two charges $+3 μ C$ and $+12 μ C$ are fixed 1 m apart, with the second one to the right. Find the magnitude and direction of the net force on a −2-nC charge when placed at the following locations: (a) halfway between the two (b) half a meter to the left of the $+3 μ C$ charge (c) half a meter above the $+12 μ C$ charge in a direction perpendicular to the line joining the two fixed charges

Show Solution

a. charge 1 is $3 μ C$ ; charge 2 is $12 μ C$ , $F_{31} = 2.16 \times 10^{- 4} N$ to the left,
$F_{32} = 8.63 \times 10^{- 4} N$ to the right,
$F_{net} = 6.47 \times 10^{- 4} N$ to the right;
b. $F_{31} = 2.16 \times 10^{- 4} N$ to the right,
$F_{32} = 9.59 \times 10^{- 5} N$ to the right,
$F_{net} = 3.12 \times 10^{- 4} N$ to the right,

;
c. ${\vec{F}}_{31 x} = - 2.76 \times 10^{- 5} N \hat{i}$ ,
${\vec{F}}_{31 y} = - 1.38 \times 10^{- 5} N \hat{j}$ ,
${\vec{F}}_{32 y} = - 8.63 \times 10^{- 4} N \hat{j}$
$\begin{array}{cc} {\vec{F}}_{net} & = - 3.86 \times 10^{- 5} N \hat{i} - 8.83 \times 10^{- 4} N \hat{j} \end{array}$

In a salt crystal, the distance between adjacent sodium and chloride ions is $2.82 \times 10^{- 10} m .$ What is the force of attraction between the two singly charged ions?

Protons in an atomic nucleus are typically $10^{- 15} m$ apart. What is the electric force of repulsion between nuclear protons?

Show Solution

$F = 230.7 N$

Suppose Earth and the Moon each carried a net negative charge −Q. Approximate both bodies as point masses and point charges.

(a) What value of Q is required to balance the gravitational attraction between Earth and the Moon?

(b) Does the distance between Earth and the Moon affect your answer? Explain.

(c) How many electrons would be needed to produce this charge?

Point charges $q_{1} = 50 μ C$ and $q_{2} = - 25 μ C$ are placed 1.0 m apart. What is the force on a third charge $q_{3} = 20 μ C$ placed midway between $q_{1}$ and $q_{2}$ ?

Show Solution

$F = 53.94 N$

Where must $q_{3}$ of the preceding problem be placed so that the net force on it is zero?

Two small balls, each of mass 5.0 g, are attached to silk threads 50 cm long, which are in turn tied to the same point on the ceiling, as shown below. When the balls are given the same charge Q, the threads hang at $5.0 °$ to the vertical, as shown below. What is the magnitude of Q? What are the signs of the two charges?

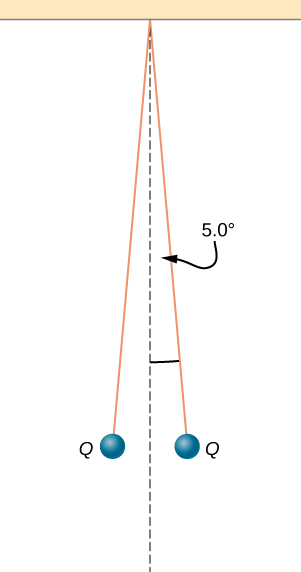
Two small balls are attached to threads which are in turn tied to the same point on the ceiling. The threads hang at an angle of 5.0 degrees to either side of the vertical. Each ball has a charge Q.

Show Solution

The tension is $T = 0.049 N$ . The horizontal component of the tension is $0.0043 N$
$d = 0.088 m, q = 6.1 \times 10^{- 8} C$ .
The charges can be positive or negative, but both have to be the same sign.

Point charges $Q_{1} = 2.0 μ C$ and $Q_{2} = 4.0 μ C$ are located at ${\vec{r}}_{1} = (4.0 \hat{i} - 2.0 \hat{j} + 5.0 \hat{k}) m$ and ${\vec{r}}_{2} = (8.0 \hat{i} + 5.0 \hat{j} - 9.0 \hat{k}) m$ . What is the force of $Q_{2}$ on $Q_{1}$ ?

The net excess charge on two small spheres (small enough to be treated as point charges) is Q. Show that the force of repulsion between the spheres is greatest when each sphere has an excess charge Q/2. Assume that the distance between the spheres is so large compared with their radii that the spheres can be treated as point charges.

Show Solution

Let the charge on one of the spheres be nQ, where n is a fraction between 0 and 1. In the numerator of Coulomb’s law, the term involving the charges is $n Q (1 - n) Q .$ This is equal to $(n - n^{2}) Q^{2}$ . Finding the maximum of this term gives $1 - 2 n = 0 \Rightarrow n = \frac{1}{2}$

Two small, identical conducting spheres repel each other with a force of 0.050 N when they are 0.25 m apart. After a conducting wire is connected between the spheres and then removed, they repel each other with a force of 0.060 N. What is the original charge on each sphere?

A charge $q = 2.0 μ C$ is placed at the point P shown below. What is the force on q?

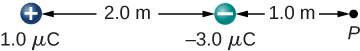
Two charges are shown, placed on a horizontal line and separated by 2.0 meters. The charge on the left is a positive 1.0 micro Coulomb charge. The charge on the right is a negative 2.0 micro Coulomb charge. Point P is 1.0 to the right of the negative charge.

Show Solution

Define right to be the positive direction and hence left is the negative direction, then $F = - 0.05 N$

What is the net electric force on the charge located at the lower right-hand corner of the triangle shown here?

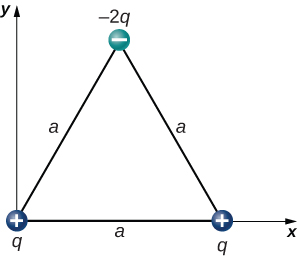
Charges are shown at the vertices of an equilateral triangle with sides length a. The bottom of the triangle is on the x axis of an x y coordinate system, and the bottom left vertex is at the origin. The charge at the origin is positive q. The charge at the bottom right hand corner is also positive q. The charge at the top vertex is negative two q.

Two fixed particles, each of charge $5.0 \times 10^{- 6} C,$ are 24 cm apart. What force do they exert on a third particle of charge $- 2.5 \times 10^{- 6} C$ that is 13 cm from each of them?

Show Solution

The particles form triangle of sides 13, 13, and 24 cm. The x-components cancel, whereas there is a contribution to the y-component from both charges 24 cm apart. The y-axis passing through the third charge bisects the 24-cm line, creating two right triangles of sides 5, 12, and 13 cm.
$F_{y} = 2.56 N$ in the negative y-direction since the force is attractive. The net force from both charges is ${\vec{F}}_{net} = - 5.12 N \hat{j}$ .

The charges $q_{1} = 2.0 \times 10^{- 7} C, q_{2} = - 4.0 \times 10^{- 7} C,$ and $q_{3} = - 1.0 \times 10^{- 7} C$ are placed at the corners of the triangle shown below. What is the force on $q_{1} ?$

Charges are shown at the vertices of a right triangle. The bottom of the triangle is length 4 meters, the vertical side on the left is length 3 meters, and the hypotenuse is length 5 meters. The charge at the top is q sub one and positive, the charge at the bottom left is q sub 3 and negative and the charge at the bottom right is q sub 2 and negative.

What is the force on the charge q at the lower-right-hand corner of the square shown here?

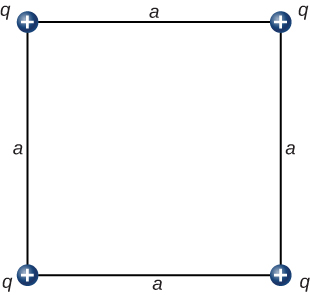
Charges are shown at the corners of a square with sides length a. All of the charges are positive and all are magnitude q.

Show Solution

The diagonal is $\sqrt{2} a$ and the components of the force due to the diagonal charge has a factor $cos θ = \frac{1}{\sqrt{2}}$ ;
${\vec{F}}_{net} = [k \frac{q^{2}}{a^{2}} + k \frac{q^{2}}{2 a^{2}} \frac{1}{\sqrt{2}}] \hat{i} - [k \frac{q^{2}}{a^{2}} + k \frac{q^{2}}{2 a^{2}} \frac{1}{\sqrt{2}}] \hat{j}$

Point charges $q_{1} = 10 μ C$ and $q_{2} = - 30 μ C$ are fixed at $r_{1} = (3.0 \hat{i} - 4.0 \hat{j}) m$ and $r_{2} = (9.0 \hat{i} + 6.0 \hat{j}) m .$ What is the force of $q_{2} on q_{1}$ ?

Glossary

Coulomb force: another term for the electrostatic force

Coulomb’s law: mathematical equation calculating the electrostatic force vector between two charged particles

electrostatic force: amount and direction of attraction or repulsion between two charged bodies; the assumption is that the source charges remain motionless

electrostatics: study of charged objects which are not in motion

permittivity of vacuum: also called the permittivity of free space, and constant describing the strength of the electric force in a vacuum

principle of superposition: useful fact that we can simply add up all of the forces due to charges acting on an object

Licenses and Attributions

Coulomb’s Law. Authored by: OpenStax College. Located at: https://openstax.org/books/university-physics-volume-2/pages/5-3-coulombs-law. License: CC BY: Attribution. License Terms: Download for free at https://openstax.org/books/university-physics-volume-2/pages/1-introduction

License

Icon for the Creative Commons Attribution 4.0 International License

Learning Objectives

Coulomb’s Law

Example

The Force on the Electron in Hydrogen

Strategy

Solution

Significance

Check Your Understanding

Multiple Source Charges

Example

The Net Force from Two Source Charges

Strategy

Solution

Significance

Check Your Understanding

Summary

Conceptual Questions

Problems

Glossary

License

Share This Book