5.5 Calculating Electric Fields of Charge Distributions

Samuel J. Ling; William Moebs; Jeff Sanny

Chapter 5. Electric Charges and Fields

5.5 Calculating Electric Fields of Charge Distributions

Learning Objectives

By the end of this section, you will be able to:

Explain what a continuous source charge distribution is and how it is related to the concept of quantization of charge
Describe line charges, surface charges, and volume charges
Calculate the field of a continuous source charge distribution of either sign

The charge distributions we have seen so far have been discrete: made up of individual point particles. This is in contrast with a continuous charge distribution, which has at least one nonzero dimension. If a charge distribution is continuous rather than discrete, we can generalize the definition of the electric field. We simply divide the charge into infinitesimal pieces and treat each piece as a point charge.

Note that because charge is quantized, there is no such thing as a “truly” continuous charge distribution. However, in most practical cases, the total charge creating the field involves such a huge number of discrete charges that we can safely ignore the discrete nature of the charge and consider it to be continuous. This is exactly the kind of approximation we make when we deal with a bucket of water as a continuous fluid, rather than a collection of $H_{2} O$ molecules.

Our first step is to define a charge density for a charge distribution along a line, across a surface, or within a volume, as shown in Figure 5.22.

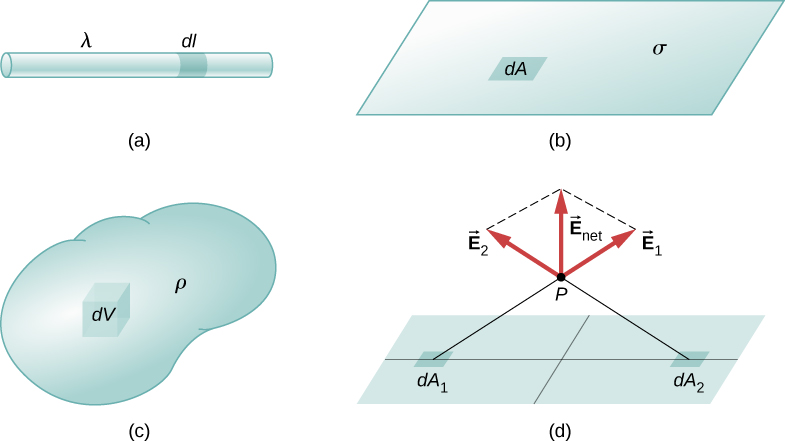
Figure a shows a long rod with linear charge density lambda. A small segment of the rod is shaded and labeled d l. Figure b shows a surface with surface charge density sigma. A small area within the surface is shaded and labeled d A. Figure c shows a volume with volume charge density rho. A small volume within it is shaded and labeled d V. Figure d shows a surface with two regions shaded and labeled q 1 and q2. A point P is identified above (not on) the surface. A thin line indicates the distance from each of the shaded regions. The vectors E 1 and E 2 are drawn at point P and point away from the respective shaded region. E net is the vector sum of E 1 and E 2. In this case, it points up, away from the surface. — **Figure 5.22** The configuration of charge differential elements for a (a) line charge, (b) sheet of charge, and (c) a volume of charge. Also note that (d) some of the components of the total electric field cancel out, with the remainder resulting in a net electric field.

Definitions of charge density:

$λ \equiv$ charge per unit length (linear charge density); units are coulombs per meter (C/m)
$σ \equiv$ charge per unit area (surface charge density); units are coulombs per square meter $(C / m^{2})$
$ρ \equiv$ charge per unit volume (volume charge density); units are coulombs per cubic meter $(C / m^{3})$

Then, for a line charge, a surface charge, and a volume charge, the summation in Equation 5.4 becomes an integral and $q_{i}$ is replaced by $d q = λ d l$ , $σ d A$ , or $ρ d V$ , respectively:

Point charge: \vec{E} (P) = \frac{1}{4 π ϵ_{0}} \sum_{i = 1}^{N} (\frac{q_{i}}{r^{2}}) \hat{r}

Line charge: \vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{line} (\frac{λ d l}{r^{2}}) \hat{r}

Surface charge: \vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{surface} (\frac{σ d A}{r^{2}}) \hat{r}

Volume charge: \vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{volume} (\frac{ρ d V}{r^{2}}) \hat{r}

The integrals are generalizations of the expression for the field of a point charge. They implicitly include and assume the principle of superposition. The “trick” to using them is almost always in coming up with correct expressions for dl, dA, or dV, as the case may be, expressed in terms of r, and also expressing the charge density function appropriately. It may be constant; it might be dependent on location.

Note carefully the meaning of r in these equations: It is the distance from the charge element $(q_{i}, λ d l, σ d A, ρ d V)$ to the location of interest, $P (x, y, z)$ (the point in space where you want to determine the field). However, don’t confuse this with the meaning of $\hat{r}$ ; we are using it and the vector notation $\vec{E}$ to write three integrals at once. That is, Equation 5.9 is actually

E_{x} (P) = \frac{1}{4 π ϵ_{0}} {\int_{line} (\frac{λ d l}{r^{2}})}_{x}, E_{y} (P) = \frac{1}{4 π ϵ_{0}} {\int_{line} (\frac{λ d l}{r^{2}})}_{y}, E_{z} (P) = \frac{1}{4 π ϵ_{0}} {\int_{line} (\frac{λ d l}{r^{2}})}_{z} .

Example

Electric Field of a Line Segment

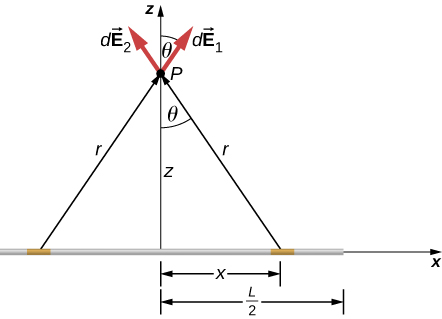
Find the electric field a distance z above the midpoint of a straight line segment of length L that carries a uniform line charge density $λ$ .

Strategy

Since this is a continuous charge distribution, we conceptually break the wire segment into differential pieces of length dl, each of which carries a differential amount of charge $d q = λ d l$ . Then, we calculate the differential field created by two symmetrically placed pieces of the wire, using the symmetry of the setup to simplify the calculation (Figure 5.23). Finally, we integrate this differential field expression over the length of the wire (half of it, actually, as we explain below) to obtain the complete electric field expression.

A long, thin wire is on the x axis. The end of the wire is a distance z from the center of the wire. A small segment of the wire, a distance x to the right of the center of the wire, is shaded. Another segment, the same distance to the left of center, is also shaded. Point P is a distance z above the center of the wire, on the z axis. Point P is a distance r from each shaded region. The r vectors point from each shaded region to point P. Vectors d E 1 and d E 2 are drawn at point P. d E 1 points away from the left side shaded region and points up and right, at an angle theta to the z axis. d E 2 points away from the right side shaded region and points up and r left, making the same angle with the vertical as d E 1. The two d E vectors are equal in length. — **Figure 5.23** A uniformly charged segment of wire. The electric field at point P can be found by applying the superposition principle to symmetrically placed charge elements and integrating.

Solution

Show Answer

Before we jump into it, what do we expect the field to “look like” from far away? Since it is a finite line segment, from far away, it should look like a point charge. We will check the expression we get to see if it meets this expectation.

The electric field for a line charge is given by the general expression

\vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{line} \frac{λ d l}{r^{2}} \hat{r} .

The symmetry of the situation (our choice of the two identical differential pieces of charge) implies the horizontal (x)-components of the field cancel, so that the net field points in the z-direction. Let’s check this formally.

The total field $\vec{E} (P)$ is the vector sum of the fields from each of the two charge elements (call them ${\vec{E}}_{1}$ and ${\vec{E}}_{2}$ , for now):

\vec{E} (P) = {\vec{E}}_{1} + {\vec{E}}_{2} = E_{1 x} \hat{i} + E_{1 z} \hat{k} + E_{2 x} (− \hat{i}) + E_{2 z} \hat{k} .

Because the two charge elements are identical and are the same distance away from the point P where we want to calculate the field, $E_{1 x} = E_{2 x},$ so those components cancel. This leaves

\vec{E} (P) = E_{1 z} \hat{k} + E_{2 z} \hat{k} = E_{1} cos θ \hat{k} + E_{2} cos θ \hat{k} .

These components are also equal, so we have

\begin{array}{cc} \vec{E} (P) & = \frac{1}{4 π ϵ_{0}} \int \frac{λ d l}{r^{2}} cos θ \hat{k} + \frac{1}{4 π ϵ_{0}} \int \frac{λ d l}{r^{2}} cos θ \hat{k} \\ = \frac{1}{4 π ϵ_{0}} \int_{0}^{L / 2} \frac{2 λ d x}{r^{2}} cos θ \hat{k} \end{array}

where our differential line element dl is dx, in this example, since we are integrating along a line of charge that lies on the x-axis. (The limits of integration are 0 to $\frac{L}{2}$ , not $- \frac{L}{2}$ to $+ \frac{L}{2}$ , because we have constructed the net field from two differential pieces of charge dq. If we integrated along the entire length, we would pick up an erroneous factor of 2.)

In principle, this is complete. However, to actually calculate this integral, we need to eliminate all the variables that are not given. In this case, both r and $θ$ change as we integrate outward to the end of the line charge, so those are the variables to get rid of. We can do that the same way we did for the two point charges: by noticing that

r = {(z^{2} + x^{2})}^{1 / 2}

and

cos θ = \frac{z}{r} = \frac{z}{{(z^{2} + x^{2})}^{1 / 2}} .

Substituting, we obtain

\begin{array}{cc} \vec{E} (P) & = \frac{1}{4 π ϵ_{0}} \int_{0}^{L / 2} \frac{2 λ d x}{(z^{2} + x^{2})} \frac{z}{{(z^{2} + x^{2})}^{1 / 2}} \hat{k} \\ = \frac{1}{4 π ϵ_{0}} \int_{0}^{L / 2} \frac{2 λ z}{{(z^{2} + x^{2})}^{3 / 2}} d x \hat{k} \\ = \frac{2 λ z}{4 π ϵ_{0}} [\frac{x}{z^{2} \sqrt{z^{2} + x^{2}}}] |_{0}^{L / 2} \hat{k} \end{array}

which simplifies to

\vec{E} (z) = \frac{1}{4 π ϵ_{0}} \frac{λ L}{z \sqrt{z^{2} + \frac{L^{2}}{4}}} \hat{k} .

Significance

Notice, once again, the use of symmetry to simplify the problem. This is a very common strategy for calculating electric fields. The fields of nonsymmetrical charge distributions have to be handled with multiple integrals and may need to be calculated numerically by a computer.

Check Your Understanding

How would the strategy used above change to calculate the electric field at a point a distance z above one end of the finite line segment?

Show Solution

We will no longer be able to take advantage of symmetry. Instead, we will need to calculate each of the two components of the electric field with their own integral.

Example

Electric Field of an Infinite Line of Charge

Find the electric field a distance z above the midpoint of an infinite line of charge that carries a uniform line charge density $λ$ .

Strategy

This is exactly like the preceding example, except the limits of integration will be $− \infty$ to $+ \infty$ .

Solution

Show Answer

Again, the horizontal components cancel out, so we wind up with

\vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{− \infty}^{\infty} \frac{λ d x}{r^{2}} cos θ \hat{k}

where our differential line element dl is dx, in this example, since we are integrating along a line of charge that lies on the x-axis. Again,

cos θ = \frac{z}{r} = \frac{z}{{(z^{2} + x^{2})}^{1 / 2}} .

Substituting, we obtain

\begin{array}{cc} \vec{E} (P) & = \frac{1}{4 π ϵ_{0}} \int_{− \infty}^{\infty} \frac{λ d x}{(z^{2} + x^{2})} \frac{z}{{(z^{2} + x^{2})}^{1 / 2}} \hat{k} \\ = \frac{1}{4 π ϵ_{0}} \int_{− \infty}^{\infty} \frac{λ z}{{(z^{2} + x^{2})}^{3 / 2}} d x \hat{k} \\ = \frac{λ z}{4 π ϵ_{0}} {[\frac{x}{z^{2} \sqrt{z^{2} + x^{2}}}] |}_{− \infty}^{\infty} \hat{k}, \end{array}

which simplifies to

\vec{E} (z) = \frac{1}{4 π ϵ_{0}} \frac{2 λ}{z} \hat{k} .

Significance

Our strategy for working with continuous charge distributions also gives useful results for charges with infinite dimension.

In the case of a finite line of charge, note that for $z ≫ L$ , $z^{2}$ dominates the L in the denominator, so that Equation 5.12 simplifies to

\vec{E} \approx \frac{1}{4 π ϵ_{0}} \frac{λ L}{z^{2}} \hat{k} .

If you recall that $λ L = q$ , the total charge on the wire, we have retrieved the expression for the field of a point charge, as expected.

In the limit $L \to \infty$ , on the other hand, we get the field of an infinite straight wire, which is a straight wire whose length is much, much greater than either of its other dimensions, and also much, much greater than the distance at which the field is to be calculated:

\vec{E} (z) = \frac{1}{4 π ϵ_{0}} \frac{2 λ}{z} \hat{k} .

An interesting artifact of this infinite limit is that we have lost the usual $1 / r^{2}$ dependence that we are used to. This will become even more intriguing in the case of an infinite plane.

Example

Electric Field due to a Ring of Charge

A ring has a uniform charge density $λ$ , with units of coulomb per unit meter of arc. Find the electric potential at a point on the axis passing through the center of the ring.

Strategy

We use the same procedure as for the charged wire. The difference here is that the charge is distributed on a circle. We divide the circle into infinitesimal elements shaped as arcs on the circle and use polar coordinates shown in Figure 5.24.

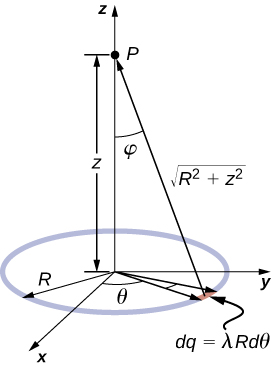
A ring of radius R is shown in the x y plane of an x y z coordinate system. The ring is centered on the origin. A small segment of the ring is shaded. The segment is at an angle of theta from the x axis, subtends an angle of d theta, and contains a charge of d q equal to lambda R d theta. Point P is on the z axis, a distance of z above the center of the ring. The distance from the shaded segment to point P is equal to the square root of R squared plus squared. — **Figure 5.24** The system and variable for calculating the electric field due to a ring of charge.

Solution

Show Answer

The electric field for a line charge is given by the general expression

\vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{line} \frac{λ d l}{r^{2}} \hat{r} .

A general element of the arc between $θ$ and $θ + d θ$ is of length $R d θ$ and therefore contains a charge equal to $λ R d θ .$ The element is at a distance of $r = \sqrt{z^{2} + R^{2}}$ from P, the angle is $cos φ = \frac{z}{\sqrt{z^{2} + R^{2}}}$ , and therefore the electric field is

\begin{array}{cc} \vec{E} (P) & = \frac{1}{4 π ϵ_{0}} \int_{line} \frac{λ d l}{r^{2}} \hat{r} = \frac{1}{4 π ϵ_{0}} \int_{0}^{2 π} \frac{λ R d θ}{z^{2} + R^{2}} \frac{z}{\sqrt{z^{2} + R^{2}}} \hat{z} \\ = \frac{1}{4 π ϵ_{0}} \frac{λ R z}{{(z^{2} + R^{2})}^{3 / 2}} \hat{z} \int_{0}^{2 π} d θ = \frac{1}{4 π ϵ_{0}} \frac{2 π λ R z}{{(z^{2} + R^{2})}^{3 / 2}} \hat{z} \\ = \frac{1}{4 π ϵ_{0}} \frac{q_{tot} z}{{(z^{2} + R^{2})}^{3 / 2}} \hat{z} . \end{array}

Significance

As usual, symmetry simplified this problem, in this particular case resulting in a trivial integral. Also, when we take the limit of $z >> R$ , we find that

\vec{E} \approx \frac{1}{4 π ϵ_{0}} \frac{q_{tot}}{z^{2}} \hat{z},

as we expect.

Example

The Field of a Disk

Find the electric field of a circular thin disk of radius R and uniform charge density at a distance z above the center of the disk (Figure 5.25)

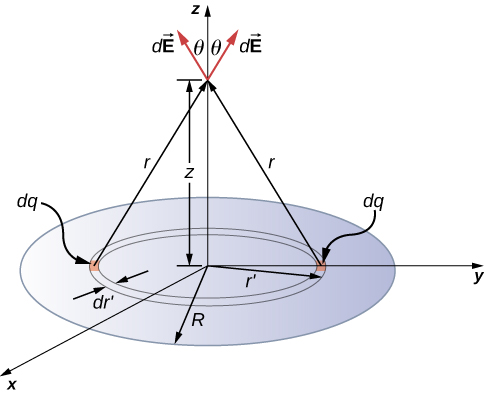
A disk of radius R is shown in the x y plane of an x y z coordinate system. The disk is centered on the origin. A ring, concentric with the disk, of radius r prime and width d r prime is indicated and two small segments on opposite sides of the ring are shaded and labeled as having charge d q. The test point is on the z axis, a distance of z above the center of the disk. The distance from each shaded segment to the test point is r. The electric field contributions, d E, due to the d q charges are shown as arrows in the directions of the associated r vectors. The d E vectors are at an angle of theta to the z axis. — **Figure 5.25** A uniformly charged disk. As in the line charge example, the field above the center of this disk can be calculated by taking advantage of the symmetry of the charge distribution.

Strategy

The electric field for a surface charge is given by

\vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{surface} \frac{σ d A}{r^{2}} \hat{r} .

To solve surface charge problems, we break the surface into symmetrical differential “stripes” that match the shape of the surface; here, we’ll use rings, as shown in the figure. Again, by symmetry, the horizontal components cancel and the field is entirely in the vertical $(\hat{k})$ direction. The vertical component of the electric field is extracted by multiplying by $cos θ$ , so

\vec{E} (P) = \frac{1}{4 π ϵ_{0}} \int_{surface} \frac{σ d A}{r^{2}} cos θ \hat{k} .

As before, we need to rewrite the unknown factors in the integrand in terms of the given quantities. In this case,

\begin{array}{ccc} d A & = & 2 π r^{′} d r^{'} \\ r^{2} & = & {r^{'}}^{2} + z^{2} \\ cos θ & = & \frac{z}{{({r^{'}}^{2} + z^{2})}^{1 / 2}} . \end{array}

(Please take note of the two different “r’s” here; r is the distance from the differential ring of charge to the point P where we wish to determine the field, whereas $r^{'}$ is the distance from the center of the disk to the differential ring of charge.) Also, we already performed the polar angle integral in writing down dA.

Solution

Show Answer

Substituting all this in, we get

\begin{array}{cc} \vec{E} (P) & = \vec{E} (z) = \frac{1}{4 π ϵ_{0}} \int_{0}^{R} \frac{σ (2 π r^{'} d r^{'}) z}{{({r^{'}}^{2} + z^{2})}^{3 / 2}} \hat{k} \\ = \frac{1}{4 π ϵ_{0}} (2 π σ z) (\frac{1}{z} - \frac{1}{\sqrt{R^{2} + z^{2}}}) \hat{k} \end{array}

or, more simply,

\vec{E} (z) = \frac{1}{4 π ϵ_{0}} (2 π σ - \frac{2 π σ z}{\sqrt{R^{2} + z^{2}}}) \hat{k} .

Significance

Again, it can be shown (via a Taylor expansion) that when $z ≫ R$ , this reduces to

\vec{E} (z) \approx \frac{1}{4 π ϵ_{0}} \frac{σ π R^{2}}{z^{2}} \hat{k},

which is the expression for a point charge $Q = σ π R^{2} .$

Check Your Understanding

How would the above limit change with a uniformly charged rectangle instead of a disk?

Show Solution

The point charge would be $Q = σ a b$ where a and b are the sides of the rectangle but otherwise identical.

As $R \to \infty$ , Equation 5.14 reduces to the field of an infinite plane, which is a flat sheet whose area is much, much greater than its thickness, and also much, much greater than the distance at which the field is to be calculated:

\vec{E} = \frac{σ}{2 ϵ_{0}} \hat{k} .

Note that this field is constant. This surprising result is, again, an artifact of our limit, although one that we will make use of repeatedly in the future. To understand why this happens, imagine being placed above an infinite plane of constant charge. Does the plane look any different if you vary your altitude? No—you still see the plane going off to infinity, no matter how far you are from it. It is important to note that Equation 5.15 is because we are above the plane. If we were below, the field would point in the $− \hat{k}$ direction.

Example

The Field of Two Infinite Planes

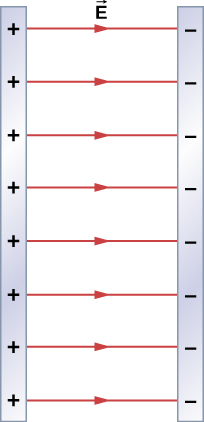
Find the electric field everywhere resulting from two infinite planes with equal but opposite charge densities (Figure 5.26).

The figure shows two vertically oriented parallel plates A and B separated by a distance d. Plate A is positively charged and B is negatively charged. Electric field lines are parallel between the plates and curved outward at the ends of the plates. A charge q is moved from A to B. The work done W equals q times V sub A B, and the electric field intensity E equals V sub A B over d. — **Figure 5.26** Two charged infinite planes. Note the direction of the electric field.

Strategy

We already know the electric field resulting from a single infinite plane, so we may use the principle of superposition to find the field from two.

Solution

Show Answer

The electric field points away from the positively charged plane and toward the negatively charged plane. Since the $σ$ are equal and opposite, this means that in the region outside of the two planes, the electric fields cancel each other out to zero.

However, in the region between the planes, the electric fields add, and we get

\vec{E} = \frac{σ}{ϵ_{0}} \hat{i}

for the electric field. The $\hat{i}$ is because in the figure, the field is pointing in the +x-direction.

Significance

Systems that may be approximated as two infinite planes of this sort provide a useful means of creating uniform electric fields.

Check Your Understanding

What would the electric field look like in a system with two parallel positively charged planes with equal charge densities?

Show Solution

The electric field would be zero in between, and have magnitude $\frac{σ}{ϵ_{0}}$ everywhere else.

Summary

A very large number of charges can be treated as a continuous charge distribution, where the calculation of the field requires integration. Common cases are:
- one-dimensional (like a wire); uses a line charge density $λ$
- two-dimensional (metal plate); uses surface charge density $σ$
- three-dimensional (metal sphere); uses volume charge density $ρ$
The “source charge” is a differential amount of charge dq. Calculating dq depends on the type of source charge distribution:

$d q = λ d l; d q = σ d A; d q = ρ d V .$
Symmetry of the charge distribution is usually key.
Important special cases are the field of an “infinite” wire and the field of an “infinite” plane.

Conceptual Questions

Give a plausible argument as to why the electric field outside an infinite charged sheet is constant.

Show Solution

At infinity, we would expect the field to go to zero, but because the sheet is infinite in extent, this is not the case. Everywhere you are, you see an infinite plane in all directions.

Compare the electric fields of an infinite sheet of charge, an infinite, charged conducting plate, and infinite, oppositely charged parallel plates.

Describe the electric fields of an infinite charged plate and of two infinite, charged parallel plates in terms of the electric field of an infinite sheet of charge.

Show Solution

The infinite charged plate would have $E = \frac{σ}{2 ϵ_{0}}$ everywhere. The field would point toward the plate if it were negatively charged and point away from the plate if it were positively charged. The electric field of the parallel plates would be zero between them if they had the same charge, and E would be $E = \frac{σ}{ϵ_{0}}$ everywhere else. If the charges were opposite, the situation is reversed, zero outside the plates and $E = \frac{σ}{ϵ_{0}}$ between them.

A negative charge is placed at the center of a ring of uniform positive charge. What is the motion (if any) of the charge? What if the charge were placed at a point on the axis of the ring other than the center?

Problems

A thin conducting plate 1.0 m on the side is given a charge of $- 2.0 \times 10^{- 6} C$ . An electron is placed 1.0 cm above the center of the plate. What is the acceleration of the electron?

Calculate the magnitude and direction of the electric field 2.0 m from a long wire that is charged uniformly at $λ = 4.0 \times 10^{- 6} C/m .$

Show Solution

$\vec{E} (z) = 3.6 \times 10^{4} N / C \hat{k}$

Two thin conducting plates, each 25.0 cm on a side, are situated parallel to one another and 5.0 mm apart. If $10^{- 11}$ electrons are moved from one plate to the other, what is the electric field between the plates?

The charge per unit length on the thin rod shown below is $λ$ . What is the electric field at the point P? (Hint: Solve this problem by first considering the electric field $d \vec{E}$ at P due to a small segment dx of the rod, which contains charge $d q = λ d x$ . Then find the net field by integrating $d \vec{E}$ over the length of the rod.)

A horizontal rod of length L is shown. The rod has total charge q. Point P is a distance a to the right of the right end of the rod.

Show Solution

$d E = \frac{1}{4 π ϵ_{0}} \frac{λ d x}{{(x + a)}^{2}}, E = \frac{λ}{4 π ϵ_{0}} [\frac{1}{l + a} - \frac{1}{a}]$

The charge per unit length on the thin semicircular wire shown below is $λ$ . What is the electric field at the point P?

A semicircular arc of radius r is shown. The arc has total charge q. Point P is at the center of the circle of which the arc is a part.

Two thin parallel conducting plates are placed 2.0 cm apart. Each plate is 2.0 cm on a side; one plate carries a net charge of $8.0 μ C,$ and the other plate carries a net charge of $- 8.0 μ C .$ What is the charge density on the inside surface of each plate? What is the electric field between the plates?

Show Solution

$σ = 0.02 C / m^{2} E = 2.26 \times 10^{9} N / C$

A thin conducing plate 2.0 m on a side is given a total charge of $- 10.0 μ C$ . (a) What is the electric field $1.0 cm$ above the plate? (b) What is the force on an electron at this point? (c) Repeat these calculations for a point 2.0 cm above the plate. (d) When the electron moves from 1.0 to 2,0 cm above the plate, how much work is done on it by the electric field?

A total charge q is distributed uniformly along a thin, straight rod of length L (see below). What is the electric field at $P_{1} ? At P_{2} ?$

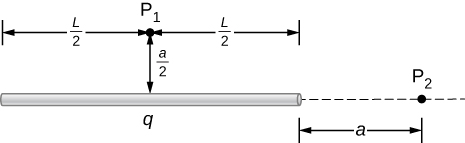
A horizontal rod of length L is shown. The rod has total charge q. Point P 1 is a distance a over 2 above the midpoint of the rod, so that the horizontal distance from P 1 to each end of the rod is L over 2. Point P 2 is a distance a to the right of the right end of the rod.

Show Solution

At $P_{1}$ : $\vec{E} (y) = \frac{1}{4 π ϵ_{0}} \frac{λ L}{y \sqrt{y^{2} + \frac{L^{2}}{4}}} \hat{j} \Rightarrow \frac{1}{4 π ϵ_{0}} \frac{q}{\frac{a}{2} \sqrt{{(\frac{a}{2})}^{2} + \frac{L^{2}}{4}}} \hat{j} = \frac{1}{π ϵ_{0}} \frac{q}{a \sqrt{a^{2} + L^{2}}} \hat{j}$
At $P_{2} :$ Put the origin at the end of L.
$d E = \frac{1}{4 π ϵ_{0}} \frac{λ d x}{{(x + a)}^{2}}, \vec{E} = - \frac{q}{4 π ϵ_{0} l} [\frac{1}{l + a} - \frac{1}{a}] \hat{i}$

Charge is distributed along the entire x-axis with uniform density $λ .$ How much work does the electric field of this charge distribution do on an electron that moves along the y-axis from $y = a to y = b ?$

Charge is distributed along the entire x-axis with uniform density $λ_{x}$ and along the entire y-axis with uniform density $λ_{y} .$ Calculate the resulting electric field at (a) $\vec{r} = a \hat{i} + b \hat{j}$ and (b) $\vec{r} = c \hat{k} .$

Show Solution

a. $\vec{E} (\vec{r}) = \frac{1}{4 π ϵ_{0}} \frac{2 λ_{x}}{b} \hat{i} + \frac{1}{4 π ϵ_{0}} \frac{2 λ_{y}}{a} \hat{j}$ ; b. $\frac{1}{4 π ϵ_{0}} \frac{2 (λ_{x} + λ_{y})}{c} \hat{k}$

A rod bent into the arc of a circle subtends an angle $2 θ$ at the center P of the circle (see below). If the rod is charged uniformly with a total charge Q, what is the electric field at P?

An arc that is part of a circle of radius R and with center P is shown. The arc extends from an angle theta to the left of vertical to an angle theta to the right of vertical.

A proton moves in the electric field $\vec{E} = 200 \hat{i} N/C .$ (a) What are the force on and the acceleration of the proton? (b) Do the same calculation for an electron moving in this field.

Show Solution

a. $\vec{F} = 3.2 \times 10^{- 17} N \hat{i}$ ,
$\vec{a} = 1.92 \times 10^{10} m / s^{2} \hat{i}$ ;
b. $\vec{F} = - 3.2 \times 10^{- 17} N \hat{i}$ ,
$\vec{a} = - 3.51 \times 10^{13} m / s^{2} \hat{i}$

An electron and a proton, each starting from rest, are accelerated by the same uniform electric field of 200 N/C. Determine the distance and time for each particle to acquire a kinetic energy of $3.2 \times 10^{- 16} J .$

A spherical water droplet of radius $25 μ m$ carries an excess 250 electrons. What vertical electric field is needed to balance the gravitational force on the droplet at the surface of the earth?

Show Solution

$m = 6.5 \times 10^{- 11} kg$ ,
$E = 1.6 \times 10^{7} N / C$

A proton enters the uniform electric field produced by the two charged plates shown below. The magnitude of the electric field is $4.0 \times 10^{5} N/C,$ and the speed of the proton when it enters is $1.5 \times 10^{7} m/s .$ What distance d has the proton been deflected downward when it leaves the plates?

Two oppositely charged horizontal plates are parallel to each other. The upper plate is positive and the lower is negative. The plates are 12.0 centimeters long. The path of a positive proton is shown passing from left to right between the plates. It enters moving horizontally and deflects down toward the negative plate, emerging a distance d below the straight line trajectory.

Shown below is a small sphere of mass 0.25 g that carries a charge of $9.0 \times 10^{- 10} C .$ The sphere is attached to one end of a very thin silk string 5.0 cm long. The other end of the string is attached to a large vertical conducting plate that has a charge density of $30 \times 10^{- 6} {C/m}^{2} .$ What is the angle that the string makes with the vertical?

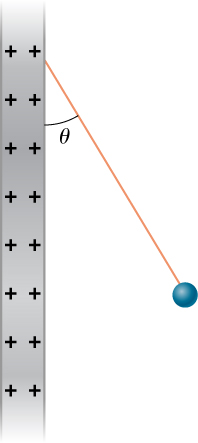
A small sphere is attached to the lower end of a string. The other end of the string is attached to a large vertical conducting plate that has a uniform positive charge density. The string makes an angle of theta with the vertical.

Show Solution

$E = 1.70 \times 10^{6} N / C$ ,
$F = 1.53 \times 10^{- 3} N T cos θ = m g T sin θ = q E$ ,
$tan θ = 0.62 \Rightarrow θ = 32.0 °$ ,
This is independent of the length of the string.

Two infinite rods, each carrying a uniform charge density $λ,$ are parallel to one another and perpendicular to the plane of the page. (See below.) What is the electrical field at $P_{1} ? At P_{2} ?$

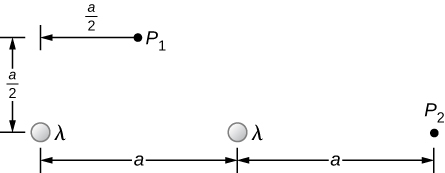
An end view of the arrangement in the problem is shown. Two rods are parallel to one another and perpendicular to the plane of the page. They are separated by a horizontal distance of a. Pint P 1 is a distance of a over 2 above the midpoint between the rods, and so also a distance of a over 2 horizontally from each rod. Point P 2 is a distance of a to the right of the rightmost rod.

Positive charge is distributed with a uniform density $λ$ along the positive x-axis from $r to \infty,$ along the positive y-axis from $r to \infty,$ and along a $90 °$ arc of a circle of radius r, as shown below. What is the electric field at O?

A uniform distribution of positive charges is shown on an x y coordinate system. The charges are distributed along a 90 degree arc of a circle of radius r in the first quadrant, centered on the origin. The distribution continues along the positive x and y axes from r to infinity.

Show Solution

circular arc $d E_{x} (− \hat{i}) = \frac{1}{4 π ϵ_{0}} \frac{λ d s}{r^{2}} cos θ (− \hat{i})$ ,
${\vec{E}}_{x} = \frac{λ}{4 π ϵ_{0} r} (− \hat{i})$ ,
$d E_{y} (− \hat{i}) = \frac{1}{4 π ϵ_{0}} \frac{λ d s}{r^{2}} sin θ (− \hat{j})$ ,
${\vec{E}}_{y} = \frac{λ}{4 π ϵ_{0} r} (− \hat{j})$ ;
y-axis: ${\vec{E}}_{x} = \frac{λ}{4 π ϵ_{0} r} (− \hat{i})$ ;
x-axis: ${\vec{E}}_{y} = \frac{λ}{4 π ϵ_{0} r} (− \hat{j})$ ,
$\vec{E} = \frac{λ}{2 π ϵ_{0} r} (− \hat{i}) + \frac{λ}{2 π ϵ_{0} r} (− \hat{j})$

From a distance of 10 cm, a proton is projected with a speed of $v = 4.0 \times 10^{6} m/s$ directly at a large, positively charged plate whose charge density is $σ = 2.0 \times 10^{- 5} {C/m}^{2} .$ (See below.) (a) Does the proton reach the plate? (b) If not, how far from the plate does it turn around?

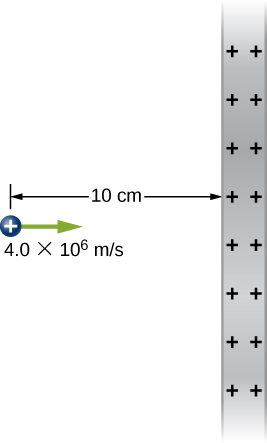
A positive charge is shown at a distance of 10 centimeters and moving to the right with a speed of 4.0 times 10 to the 6 meters per second, directly toward a large, positively and uniformly charged vertical plate.

A particle of mass m and charge $− q$ moves along a straight line away from a fixed particle of charge Q. When the distance between the two particles is $r_{0}, − q$ is moving with a speed $v_{0} .$ (a) Use the work-energy theorem to calculate the maximum separation of the charges. (b) What do you have to assume about $v_{0}$ to make this calculation? (c) What is the minimum value of $v_{0}$ such that $− q$ escapes from Q?

Show Solution

a. $W = \frac{1}{2} m (v^{2} - v_{0}^{2})$ , $\frac{Q q}{4 π ϵ_{0}} (\frac{1}{r} - \frac{1}{r_{0}}) = \frac{1}{2} m (v^{2} - v_{0}^{2}) \Rightarrow r_{0} - r = \frac{4 π ϵ_{0}}{Q q} \frac{1}{2} r r_{0} m (v^{2} - v_{0}^{2})$ ; b. $r_{0} - r$ is negative; therefore, $v_{0} > v$ , $r \to \infty, and v \to 0 : \frac{Q q}{4 π ϵ_{0}} (- \frac{1}{r_{0}}) = - \frac{1}{2} m v_{0}^{2} \Rightarrow v_{0} = \sqrt{\frac{Q q}{2 π ϵ_{0} m r_{0}}}$

Glossary

continuous charge distribution: total source charge composed of so large a number of elementary charges that it must be treated as continuous, rather than discrete

infinite plane: flat sheet in which the dimensions making up the area are much, much greater than its thickness, and also much, much greater than the distance at which the field is to be calculated; its field is constant

infinite straight wire: straight wire whose length is much, much greater than either of its other dimensions, and also much, much greater than the distance at which the field is to be calculated

linear charge density: amount of charge in an element of a charge distribution that is essentially one-dimensional (the width and height are much, much smaller than its length); its units are C/m

surface charge density: amount of charge in an element of a two-dimensional charge distribution (the thickness is small); its units are $C / m^{2}$

volume charge density: amount of charge in an element of a three-dimensional charge distribution; its units are $C / m^{3}$

Licenses and Attributions

Calculating Electric Fields of Charge Distributions. Authored by: OpenStax College. Located at: https://openstax.org/books/university-physics-volume-2/pages/5-5-calculating-electric-fields-of-charge-distributions. License: CC BY: Attribution. License Terms: Download for free at https://openstax.org/books/university-physics-volume-2/pages/1-introduction

License

Icon for the Creative Commons Attribution 4.0 International License

Learning Objectives

Example

Electric Field of a Line Segment

Strategy

Solution

Significance

Check Your Understanding

Example

Electric Field of an Infinite Line of Charge

Strategy

Solution

Significance

Example

Electric Field due to a Ring of Charge

Strategy

Solution

Significance

Example

The Field of a Disk

Strategy

Solution

Significance

Check Your Understanding

Example

The Field of Two Infinite Planes

Strategy

Solution

Significance

Check Your Understanding

Summary

Conceptual Questions

Problems

Glossary

License

Share This Book