Chapter 11. Magnetic Forces and Fields
11.7 Applications of Magnetic Forces and Fields
Learning Objectives
By the end of this section, you will be able to:
- Explain how a mass spectrometer works to separate charges
- Explain how a cyclotron works
Being able to manipulate and sort charged particles allows deeper experimentation to understand what matter is made of. We first look at a mass spectrometer to see how we can separate ions by their charge-to-mass ratio. Then we discuss cyclotrons as a method to accelerate charges to very high energies.
Mass Spectrometer
The mass spectrometer is a device that separates ions according to their charge-to-mass ratios. One particular version, the Bainbridge mass spectrometer, is illustrated in Figure 11.19. Ions produced at a source are first sent through a velocity selector, where the magnetic force is equally balanced with the electric force. These ions all emerge with the same speed [latex]v=E\text{/}B[/latex] since any ion with a different velocity is deflected preferentially by either the electric or magnetic force, and ultimately blocked from the next stage. They then enter a uniform magnetic field [latex]{B}_{0}[/latex] where they travel in a circular path whose radius R is given by Equation 11.3. The radius is measured by a particle detector located as shown in the figure.
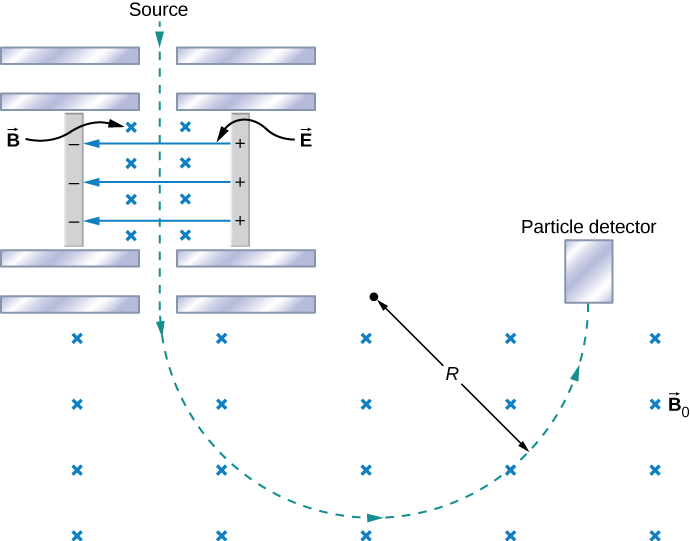
The relationship between the charge-to-mass ratio q/m and the radius R is determined by combining Equation 11.3 and Equation 11.25:
Since most ions are singly charged [latex]\left(q=1.6\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-19}\phantom{\rule{0.2em}{0ex}}\text{C}\right),[/latex] measured values of R can be used with this equation to determine the mass of ions. With modern instruments, masses can be determined to one part in [latex]{10}^{8}.[/latex]
An interesting use of a spectrometer is as part of a system for detecting very small leaks in a research apparatus. In low-temperature physics laboratories, a device known as a dilution refrigerator uses a mixture of He-3, He-4, and other cryogens to reach temperatures well below 1 K. The performance of the refrigerator is severely hampered if even a minute leak between its various components occurs. Consequently, before it is cooled down to the desired temperature, the refrigerator is subjected to a leak test. A small quantity of gaseous helium is injected into one of its compartments, while an adjacent, but supposedly isolated, compartment is connected to a high-vacuum pump to which a mass spectrometer is attached. A heated filament ionizes any helium atoms evacuated by the pump. The detection of these ions by the spectrometer then indicates a leak between the two compartments of the dilution refrigerator.
In conjunction with gas chromatography, mass spectrometers are used widely to identify unknown substances. While the gas chromatography portion breaks down the substance, the mass spectrometer separates the resulting ionized molecules. This technique is used with fire debris to ascertain the cause, in law enforcement to identify illegal drugs, in security to identify explosives, and in many medicinal applications.
Cyclotron
The cyclotron was developed by E.O. Lawrence to accelerate charged particles (usually protons, deuterons, or alpha-particles) to large kinetic energies. These particles are then used for nuclear-collision experiments to produce radioactive isotopes. A cyclotron is illustrated in Figure 11.20. The particles move between two flat, semi-cylindrical metallic containers D1 and D2, called dees. The dees are enclosed in a larger metal container, and the apparatus is placed between the poles of an electromagnet that provides a uniform magnetic field. Air is removed from the large container so that the particles neither lose energy nor are deflected because of collisions with air molecules. The dees are connected to a high-frequency voltage source that provides an alternating electric field in the small region between them. Because the dees are made of metal, their interiors are shielded from the electric field.
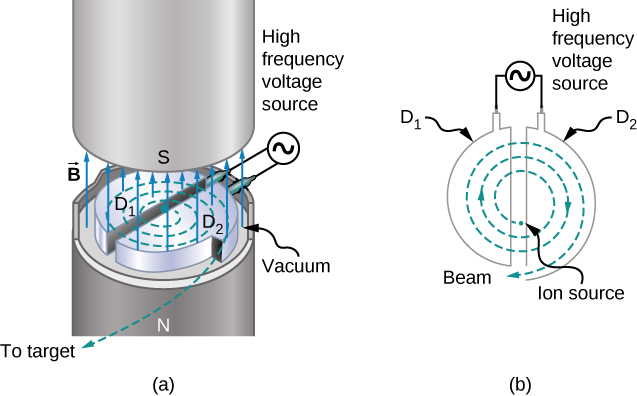
Suppose a positively charged particle is injected into the gap between the dees when D2 is at a positive potential relative to D1. The particle is then accelerated across the gap and enters D1 after gaining kinetic energy qV, where V is the average potential difference the particle experiences between the dees. When the particle is inside D1, only the uniform magnetic field [latex]\stackrel{\to }{\textbf{B}}[/latex] of the electromagnet acts on it, so the particle moves in a circle of radius
with a period of
The period of the alternating voltage course is set at T, so while the particle is inside D1, moving along its semicircular orbit in a time T/2, the polarity of the dees is reversed. When the particle reenters the gap, D1 is positive with respect to D2, and the particle is again accelerated across the gap, thereby gaining a kinetic energy qV. The particle then enters D2, circulates in a slightly larger circle, and emerges from D2 after spending a time T/2 in this dee. This process repeats until the orbit of the particle reaches the boundary of the dees. At that point, the particle (actually, a beam of particles) is extracted from the cyclotron and used for some experimental purpose.
The operation of the cyclotron depends on the fact that, in a uniform magnetic field, a particle’s orbital period is independent of its radius and its kinetic energy. Consequently, the period of the alternating voltage source need only be set at the one value given by Equation 11.33. With that setting, the electric field accelerates particles every time they are between the dees.
If the maximum orbital radius in the cyclotron is R, then from Equation 11.32, the maximum speed of a circulating particle of mass m and charge q is
Thus, its kinetic energy when ejected from the cyclotron is
The maximum kinetic energy attainable with this type of cyclotron is approximately 30 MeV. Above this energy, relativistic effects become important, which causes the orbital period to increase with the radius. Up to energies of several hundred MeV, the relativistic effects can be compensated for by making the magnetic field gradually increase with the radius of the orbit. However, for higher energies, much more elaborate methods must be used to accelerate particles.
Particles are accelerated to very high energies with either linear accelerators or synchrotrons. The linear accelerator accelerates particles continuously with the electric field of an electromagnetic wave that travels down a long evacuated tube. The Stanford Linear Accelerator (SLAC) is about 3.3 km long and accelerates electrons and positrons (positively charged electrons) to energies of 50 GeV. The synchrotron is constructed so that its bending magnetic field increases with particle speed in such a way that the particles stay in an orbit of fixed radius. The world’s highest-energy synchrotron is located at CERN, which is on the Swiss-French border near Geneva. CERN has been of recent interest with the verified discovery of the Higgs Boson (see Particle Physics and Cosmology). This synchrotron can accelerate beams of approximately [latex]{10}^{13}[/latex] protons to energies of about [latex]{10}^{3}[/latex] GeV.
Example
Accelerating Alpha-Particles in a Cyclotron
A cyclotron used to accelerate alpha-particles ([latex]m=6.64\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-27}\text{kg},q=3.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-19}\text{C}[/latex]) has a radius of 0.50 m and a magnetic field of 1.8 T. (a) What is the period of revolution of the alpha-particles? (b) What is their maximum kinetic energy?
Strategy
- The period of revolution is approximately the distance traveled in a circle divided by the speed. Identifying that the magnetic force applied is the centripetal force, we can derive the period formula.
- The kinetic energy can be found from the maximum speed of the beam, corresponding to the maximum radius within the cyclotron.
Solution
Show Answer
- By identifying the mass, charge, and magnetic field in the problem, we can calculate the period:
- By identifying the charge, magnetic field, radius of path, and the mass, we can calculate the maximum kinetic energy:
[latex]\frac{1}{2}m{v}_{\text{max}}{}^{2}=\frac{{q}^{2}{B}^{2}{R}^{2}}{2m}=\frac{{\left(3.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-19}\text{C}\right)}^{2}{\left(1.8\text{T}\right)}^{2}{\left(0.50\text{m}\right)}^{2}}{2\left(6.65\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-27}\text{kg}\right)}=6.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-12}\text{J}=39\text{MeV.}[/latex]
Check Your Understanding
A cyclotron is to be designed to accelerate protons to kinetic energies of 20 MeV using a magnetic field of 2.0 T. What is the required radius of the cyclotron?
Show Solution
0.32 m
Summary
- A mass spectrometer is a device that separates ions according to their charge-to-mass ratios by first sending them through a velocity selector, then a uniform magnetic field.
- Cyclotrons are used to accelerate charged particles to large kinetic energies through applied electric and magnetic fields.
Key Equations
| Force on a charge in a magnetic field | [latex]\stackrel{\to }{\textbf{F}}=q\stackrel{\to }{\textbf{v}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\stackrel{\to }{\textbf{B}}[/latex] |
| Magnitude of magnetic force | [latex]F=qvB\phantom{\rule{0.1em}{0ex}}\text{sin}\phantom{\rule{0.1em}{0ex}}\theta[/latex] |
| Radius of a particle’s path in a magnetic field | [latex]r=\frac{mv}{qB}[/latex] |
| Period of a particle’s motion in a magnetic field | [latex]T=\frac{2\pi m}{qB}[/latex] |
| Force on a current-carrying wire in a uniform magnetic field | [latex]\stackrel{\to }{\textbf{F}}=I\stackrel{\to }{\textbf{l}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\stackrel{\to }{\textbf{B}}[/latex] |
| Magnetic dipole moment | [latex]\stackrel{\to }{\pmb{\mu }}=NIA\hat{\textbf{n}}[/latex] |
| Torque on a current loop | [latex]\stackrel{\to }{\pmb{\tau }}=\stackrel{\to }{\pmb{\mu }}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\stackrel{\to }{\textbf{B}}[/latex] |
| Energy of a magnetic dipole | [latex]U=\text{−}\stackrel{\to }{\pmb{\mu }}·\stackrel{\to }{\textbf{B}}[/latex] |
| Drift velocity in crossed electric and magnetic fields | [latex]{v}_{d}=\frac{E}{B}[/latex] |
| Hall potential | [latex]V=\frac{IBl}{neA}[/latex] |
| Hall potential in terms of drift velocity | [latex]V=Bl{v}_{d}[/latex] |
| Charge-to-mass ratio in a mass spectrometer | [latex]\frac{q}{m}=\frac{E}{B{B}_{0}R}[/latex] |
| Maximum speed of a particle in a cyclotron | [latex]{v}_{\text{max}}=\frac{qBR}{m}[/latex] |
Conceptual Questions
Describe the primary function of the electric field and the magnetic field in a cyclotron.
Problems
A physicist is designing a cyclotron to accelerate protons to one-tenth the speed of light. The magnetic field will have a strength of 1.5 T. Determine (a) the rotational period of the circulating protons and (b) the maximum radius of the protons’ orbit.
Show Solution
a. [latex]4.4\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-8}\text{s};[/latex] b. 0.21 m
The strengths of the fields in the velocity selector of a Bainbridge mass spectrometer are B = 0.500 T and E = [latex]1.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\phantom{\rule{0.2em}{0ex}}\text{V/m},[/latex] and the strength of the magnetic field that separates the ions is [latex]{B}_{o}=0.750\phantom{\rule{0.2em}{0ex}}\text{T}.[/latex] A stream of singly charged Li ions is found to bend in a circular arc of radius 2.32 cm. What is the mass of the Li ions?
The magnetic field in a cyclotron is 1.25 T, and the maximum orbital radius of the circulating protons is 0.40 m. (a) What is the kinetic energy of the protons when they are ejected from the cyclotron? (b) What is this energy in MeV? (c) Through what potential difference would a proton have to be accelerated to acquire this kinetic energy? (d) What is the period of the voltage source used to accelerate the protons? (e) Repeat the calculations for alpha-particles.
Show Solution
a. [latex]1.92\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-12}\text{J};[/latex] b. 12 MeV; c. 12 MV; d. [latex]5.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-8}\text{s};[/latex] e. [latex]1.92\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-12}\text{J},[/latex] 12 MeV, 12 V, [latex]10.4\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-8}\text{s}[/latex]
A mass spectrometer is being used to separate common oxygen-16 from the much rarer oxygen-18, taken from a sample of old glacial ice. (The relative abundance of these oxygen isotopes is related to climatic temperature at the time the ice was deposited.) The ratio of the masses of these two ions is 16 to 18, the mass of oxygen-16 is [latex]2.66\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-26}\text{kg},[/latex] and they are singly charged and travel at [latex]5.00\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] in a 1.20-T magnetic field. What is the separation between their paths when they hit a target after traversing a semicircle?
(a) Triply charged uranium-235 and uranium-238 ions are being separated in a mass spectrometer. (The much rarer uranium-235 is used as reactor fuel.) The masses of the ions are $latex $[latex]3.90\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-25}\text{kg}[/latex] and [latex]3.95\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-25}\text{kg},[/latex] respectively, and they travel at [latex]3.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\text{m/s}[/latex] in a 0.250-T field. What is the separation between their paths when they hit a target after traversing a semicircle? (b) Discuss whether this distance between their paths seems to be big enough to be practical in the separation of uranium-235 from uranium-238.
Show Solution
a. [latex]2.50\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-2}\text{m};[/latex] b. Yes, this distance between their paths is clearly big enough to separate the U-235 from the U-238, since it is a distance of 2.5 cm.
Additional Problems
Calculate the magnetic force on a hypothetical particle of charge [latex]1.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-19}\text{C}[/latex] moving with a velocity of [latex]6.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{4}\hat{\textbf{i}}\text{m/s}[/latex] in a magnetic field of [latex]1.2\hat{\textbf{k}}\text{T}.[/latex]
Repeat the previous problem with a new magnetic field of [latex]\left(0.4\hat{\textbf{i}}+1.2\hat{\textbf{k}}\right)\text{T}.[/latex]
Show Solution
[latex]-7.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-15}\text{N}\hat{\textbf{j}}[/latex]
An electron is projected into a uniform magnetic field [latex]\left(0.5\hat{\textbf{i}}+0.8\hat{\textbf{k}}\right)\text{T}[/latex] with a velocity of [latex]\left(3.0\hat{\textbf{i}}+4.0\hat{\textbf{j}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\phantom{\rule{0.2em}{0ex}}\text{m/s}.[/latex] What is the magnetic force on the electron?
The mass and charge of a water droplet are [latex]1.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-4}\text{g}[/latex] and [latex]2.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-8}\text{C},[/latex] respectively. If the droplet is given an initial horizontal velocity of [latex]5.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\hat{\textbf{i}}\text{m/s},[/latex] what magnetic field will keep it moving in this direction? Why must gravity be considered here?
Show Solution
[latex]9.8\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}\hat{\textbf{j}}\text{T};[/latex] the magnetic and gravitational forces must balance to maintain dynamic equilibrium
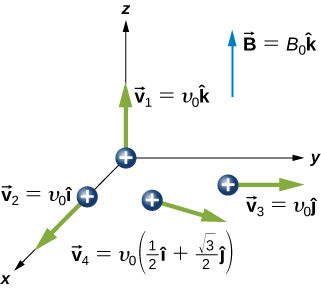
Four different proton velocities are given. For each case, determine the magnetic force on the proton in terms of e, [latex]{v}_{0},[/latex] and [latex]{B}_{0}.[/latex]
An electron of kinetic energy 2000 eV passes between parallel plates that are 1.0 cm apart and kept at a potential difference of 300 V. What is the strength of the uniform magnetic field B that will allow the electron to travel undeflected through the plates? Assume E and B are perpendicular.
Show Solution
[latex]1.13\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-3}\text{T}[/latex]
An alpha-particle ([latex]m=6.64\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-27}\text{kg},[/latex] [latex]q=3.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-19}\text{C}[/latex]) moving with a velocity [latex]\stackrel{\to }{\textbf{v}}=\left(2.0\hat{\textbf{i}}-4.0\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] enters a region where [latex]\stackrel{\to }{\textbf{E}}=\left(5.0\hat{\textbf{i}}-2.0\hat{\textbf{j}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{4}\phantom{\rule{0.2em}{0ex}}\text{V/m}[/latex] and [latex]\stackrel{\to }{\textbf{B}}=\left(1.0\hat{\textbf{i}}+4.0\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-2}\text{T}.[/latex] What is the initial force on it?
An electron moving with a velocity [latex]\stackrel{\to }{\textbf{v}}=\left(4.0\hat{\textbf{i}}+3.0\hat{\textbf{j}}+2.0\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] enters a region where there is a uniform electric field and a uniform magnetic field. The magnetic field is given by [latex]\stackrel{\to }{\textbf{B}}=\left(1.0\hat{\textbf{i}}-2.0\hat{\textbf{j}}+4.0\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-2}\text{T}.[/latex] If the electron travels through a region without being deflected, what is the electric field?
Show Solution
[latex]\left(1.6\hat{\textbf{i}}-1.4\hat{\textbf{j}}-1.1\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\phantom{\rule{0.2em}{0ex}}\text{V/m}[/latex]
At a particular instant, an electron is traveling west to east with a kinetic energy of 10 keV. Earth’s magnetic field has a horizontal component of [latex]1.8\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}\text{T}[/latex] north and a vertical component of [latex]5.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}\text{T}[/latex] down. (a) What is the path of the electron? (b) What is the radius of curvature of the path?
What is the (a) path of a proton and (b) the magnetic force on the proton that is traveling west to east with a kinetic energy of 10 keV in Earth’s magnetic field that has a horizontal component of 1.8 x 10–5 T north and a vertical component of 5.0 x 10–5 T down?
Show Solution
a. circular motion in a north, down plane; b. [latex]\left(1.61\hat{\textbf{j}}-0.58\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-14}\text{N}[/latex]
What magnetic field is required in order to confine a proton moving with a speed of [latex]4.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] to a circular orbit of radius 10 cm?
An electron and a proton move with the same speed in a plane perpendicular to a uniform magnetic field. Compare the radii and periods of their orbits.
Show Solution
The proton has more mass than the electron; therefore, its radius and period will be larger.
A proton and an alpha-particle have the same kinetic energy and both move in a plane perpendicular to a uniform magnetic field. Compare the periods of their orbits.
A singly charged ion takes [latex]2.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-3}\text{s}[/latex] to complete eight revolutions in a uniform magnetic field of magnitude [latex]2.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-2}\text{T}.[/latex] What is the mass of the ion?
Show Solution
[latex]1.3\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-25}\text{kg}[/latex]
A particle moving downward at a speed of [latex]6.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] enters a uniform magnetic field that is horizontal and directed from east to west. (a) If the particle is deflected initially to the north in a circular arc, is its charge positive or negative? (b) If B = 0.25 T and the charge-to-mass ratio (q/m) of the particle is [latex]4.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{7}\text{C/kg},[/latex] what is the radius of the path? (c) What is the speed of the particle after it has moved in the field for [latex]1.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}\text{s}?[/latex] for 2.0 s?
A proton, deuteron, and an alpha-particle are all accelerated from rest through the same potential difference. They then enter the same magnetic field, moving perpendicular to it. Compute the ratios of the radii of their circular paths. Assume that [latex]{m}_{d}=2{m}_{p}[/latex] and [latex]{m}_{\alpha }=4{m}_{p}.[/latex]
Show Solution
1:0.707:1
A singly charged ion is moving in a uniform magnetic field of [latex]7.5\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-2}\text{T}[/latex] completes 10 revolutions in [latex]3.47\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-4}\text{s}.[/latex] Identify the ion.
Two particles have the same linear momentum, but particle A has four times the charge of particle B. If both particles move in a plane perpendicular to a uniform magnetic field, what is the ratio [latex]{R}_{A}\text{/}{R}_{B}[/latex] of the radii of their circular orbits?
Show Solution
1/4
A uniform magnetic field of magnitude [latex]B[/latex] is directed parallel to the z-axis. A proton enters the field with a velocity [latex]\stackrel{\to }{\textbf{v}}=\left(4\hat{\textbf{j}}+3\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] and travels in a helical path with a radius of 5.0 cm. (a) What is the value of [latex]B[/latex]? (b) What is the time required for one trip around the helix? (c) Where is the proton [latex]5.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-7}\text{s}[/latex] after entering the field?
An electron moving along the +x -axis at [latex]5.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] enters a magnetic field that makes a [latex]{75}^{\text{o}}[/latex] angle with the x-axis of magnitude 0.20 T. Calculate the (a) pitch and (b) radius of the trajectory.
Show Solution
a. [latex]2.3\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-4}\text{m};[/latex] b. [latex]1.37\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-4}\text{m}[/latex]
(a) A 0.750-m-long section of cable carrying current to a car starter motor makes an angle of 60º with Earth’s [latex]5.5\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}\text{T}[/latex] field. What is the current when the wire experiences a force of [latex]7.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-3}\text{N}?[/latex] (b) If you run the wire between the poles of a strong horseshoe magnet, subjecting 5.00 cm of it to a 1.75-T field, what force is exerted on this segment of wire?
(a) What is the angle between a wire carrying an 8.00-A current and the 1.20-T field it is in if 50.0 cm of the wire experiences a magnetic force of 2.40 N? (b) What is the force on the wire if it is rotated to make an angle of 90º with the field?
Show Solution
a. [latex]30.0\text{°};[/latex] b. 4.80 N
A 1.0-m-long segment of wire lies along the x-axis and carries a current of 2.0 A in the positive x-direction. Around the wire is the magnetic field of [latex]\left(3.0\hat{\textbf{i}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}4.0\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-3}\text{T}.[/latex] Find the magnetic force on this segment.
A 5.0-m section of a long, straight wire carries a current of 10 A while in a uniform magnetic field of magnitude [latex]8.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-3}\text{T}.[/latex] Calculate the magnitude of the force on the section if the angle between the field and the direction of the current is (a) 45°; (b) 90°; (c) 0°; or (d) 180°.
Show Solution
a. 0.283 N; b. 0.4 N; c. 0 N; d. 0 N
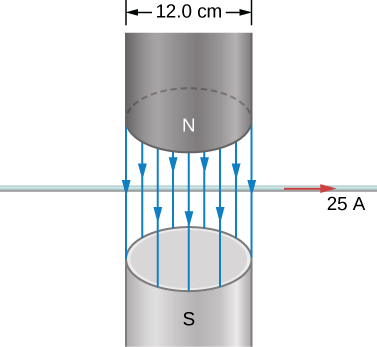
An electromagnet produces a magnetic field of magnitude 1.5 T throughout a cylindrical region of radius 6.0 cm. A straight wire carrying a current of 25 A passes through the field as shown in the accompanying figure. What is the magnetic force on the wire?
The current loop shown in the accompanying figure lies in the plane of the page, as does the magnetic field. Determine the net force and the net torque on the loop if I = 10 A and B = 1.5 T.
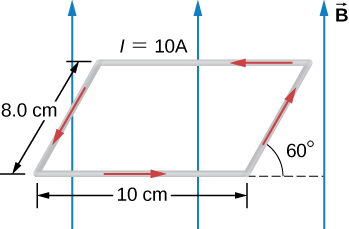
Show Solution
0 N and 0.012 Nm
A circular coil of radius 5.0 cm is wound with five turns and carries a current of 5.0 A. If the coil is placed in a uniform magnetic field of strength 5.0 T, what is the maximum torque on it?
A circular coil of wire of radius 5.0 cm has 20 turns and carries a current of 2.0 A. The coil lies in a magnetic field of magnitude 0.50 T that is directed parallel to the plane of the coil. (a) What is the magnetic dipole moment of the coil? (b) What is the torque on the coil?
Show Solution
a. [latex]0.31\phantom{\rule{0.2em}{0ex}}{\text{Am}}^{2};[/latex] b. 0.16 Nm
A current-carrying coil in a magnetic field experiences a torque that is 75% of the maximum possible torque. What is the angle between the magnetic field and the normal to the plane of the coil?
A 4.0-cm by 6.0-cm rectangular current loop carries a current of 10 A. What is the magnetic dipole moment of the loop?
Show Solution
[latex]0.024\phantom{\rule{0.2em}{0ex}}{\text{Am}}^{2}[/latex]
A circular coil with 200 turns has a radius of 2.0 cm. (a) What current through the coil results in a magnetic dipole moment of 3.0 Am2? (b) What is the maximum torque that the coil will experience in a uniform field of strength [latex]5.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-2}\text{T}?[/latex] (c) If the angle between μ and B is 45°, what is the magnitude of the torque on the coil? (d) What is the magnetic potential energy of coil for this orientation?
The current through a circular wire loop of radius 10 cm is 5.0 A. (a) Calculate the magnetic dipole moment of the loop. (b) What is the torque on the loop if it is in a uniform 0.20-T magnetic field such that [latex]\mu[/latex] and B are directed at [latex]30\text{°}[/latex] to each other? (c) For this position, what is the potential energy of the dipole?
Show Solution
a. [latex]0.16\phantom{\rule{0.2em}{0ex}}{\text{Am}}^{2};[/latex] b. 0.016 Nm; c. 0.028 J
A wire of length 1.0 m is wound into a single-turn planar loop. The loop carries a current of 5.0 A, and it is placed in a uniform magnetic field of strength 0.25 T. (a) What is the maximum torque that the loop will experience if it is square? (b) If it is circular? (c) At what angle relative to B would the normal to the circular coil have to be oriented so that the torque on it would be the same as the maximum torque on the square coil?
Consider an electron rotating in a circular orbit of radius r. Show that the magnitudes of the magnetic dipole moment μ and the angular momentum L of the electron are related by:
Show Solution
(Proof)
The Hall effect is to be used to find the sign of charge carriers in a semiconductor sample. The probe is placed between the poles of a magnet so that magnetic field is pointed up. A current is passed through a rectangular sample placed horizontally. As current is passed through the sample in the east direction, the north side of the sample is found to be at a higher potential than the south side. Decide if the number density of charge carriers is positively or negatively charged.
The density of charge carriers for copper is [latex]8.47\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{28}[/latex] electrons per cubic meter. What will be the Hall voltage reading from a probe made up of [latex]3\phantom{\rule{0.2em}{0ex}}\text{cm}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{2 cm}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{1 cm}\phantom{\rule{0.2em}{0ex}}\left(\text{L}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{W}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{T}\right)[/latex] copper plate when a current of 1.5 A is passed through it in a magnetic field of 2.5 T perpendicular to the [latex]3\phantom{\rule{0.2em}{0ex}}\text{cm}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\text{2 cm}.[/latex]
Show Solution
[latex]4.65\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-7}\text{V}[/latex]
The Hall effect is to be used to find the density of charge carriers in an unknown material. A Hall voltage 40 [latex]\text{μV}[/latex] for 3-A current is observed in a 3-T magnetic field for a rectangular sample with length 2 cm, width 1.5 cm, and height 0.4 cm. Determine the density of the charge carriers.
Show that the Hall voltage across wires made of the same material, carrying identical currents, and subjected to the same magnetic field is inversely proportional to their diameters. (Hint: Consider how drift velocity depends on wire diameter.)
Show Solution
Since [latex]E=Blv,[/latex] where the width is twice the radius, [latex]I=2r,[/latex] [latex]I=nqA{v}_{d},[/latex]
[latex]{v}_{\text{d}}=\frac{I}{nqA}=\frac{I}{nq\pi {r}^{2}}[/latex] so [latex]E=B\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}2r\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\frac{\text{I}}{nq\pi {r}^{2}}=\frac{2IB}{nq\pi r}\propto \frac{1}{r}\propto \frac{1}{d}.[/latex]
The Hall voltage is inversely proportional to the diameter of the wire.
A velocity selector in a mass spectrometer uses a 0.100-T magnetic field. (a) What electric field strength is needed to select a speed of [latex]4.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}?[/latex] (b) What is the voltage between the plates if they are separated by 1.00 cm?
Find the radius of curvature of the path of a 25.0-MeV proton moving perpendicularly to the 1.20-T field of a cyclotron.
Show Solution
[latex]6.92\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{7}\phantom{\rule{0.2em}{0ex}}\text{m/s};[/latex] 0.602 m
Unreasonable results To construct a non-mechanical water meter, a 0.500-T magnetic field is placed across the supply water pipe to a home and the Hall voltage is recorded. (a) Find the flow rate through a 3.00-cm-diameter pipe if the Hall voltage is 60.0 mV. (b) What would the Hall voltage be for the same flow rate through a 10.0-cm-diameter pipe with the same field applied?
Unreasonable results A charged particle having mass [latex]6.64\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-27}\text{kg}[/latex] (that of a helium atom) moving at [latex]8.70\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\text{m/s}[/latex] perpendicular to a 1.50-T magnetic field travels in a circular path of radius 16.0 mm. (a) What is the charge of the particle? (b) What is unreasonable about this result? (c) Which assumptions are responsible?
Show Solution
a. [latex]2.4\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-19}\text{C};[/latex] b. not an integer multiple of e; c. need to assume all charges have multiples of e, could be other forces not accounted for
Unreasonable results An inventor wants to generate 120-V power by moving a 1.00-m-long wire perpendicular to Earth’s [latex]5.00\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}\phantom{\rule{0.2em}{0ex}}\text{T}[/latex] field. (a) Find the speed with which the wire must move. (b) What is unreasonable about this result? (c) Which assumption is responsible?
Unreasonable results Frustrated by the small Hall voltage obtained in blood flow measurements, a medical physicist decides to increase the applied magnetic field strength to get a 0.500-V output for blood moving at 30.0 cm/s in a 1.50-cm-diameter vessel. (a) What magnetic field strength is needed? (b) What is unreasonable about this result? (c) Which premise is responsible?
Show Solution
a. B = 5 T; b. very large magnet; c. applying such a large voltage
Challenge Problems
A particle of charge +q and mass m moves with velocity [latex]{\stackrel{\to }{\textbf{v}}}_{0}[/latex] pointed in the +y-direction as it crosses the x-axis at x = R at a particular time. There is a negative charge –Q fixed at the origin, and there exists a uniform magnetic field [latex]{\stackrel{\to }{\textbf{B}}}_{0}[/latex] pointed in the +z-direction. It is found that the particle describes a circle of radius R about –Q. Find [latex]{\stackrel{\to }{\textbf{B}}}_{0}[/latex] in terms of the given quantities.
A proton of speed v = [latex]6\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\text{m/s}[/latex] enters a region of uniform magnetic field of B = 0.5 T at an angle of [latex]q=30\text{°}[/latex] to the magnetic field. In the region of magnetic field proton describes a helical path with radius R and pitch p (distance between loops). Find R and p.
Show Solution
[latex]R=\left(mv\phantom{\rule{0.1em}{0ex}}\text{sin}\phantom{\rule{0.1em}{0ex}}\theta \right)\text{/}qB;[/latex][latex]p=\left(\frac{2\pi m}{eB}\right)v\text{cos}\phantom{\rule{0.1em}{0ex}}\theta[/latex]
A particle’s path is bent when it passes through a region of non-zero magnetic field although its speed remains unchanged. This is very useful for “beam steering” in particle accelerators. Consider a proton of speed [latex]4\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{6}\text{m/s}[/latex] entering a region of uniform magnetic field 0.2 T over a 5-cm-wide region. Magnetic field is perpendicular to the velocity of the particle. By how much angle will the path of the proton be bent? (Hint: The particle comes out tangent to a circle.)
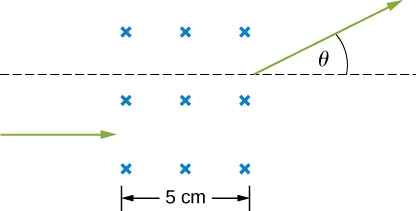
In a region a non-uniform magnetic field exists such that [latex]{B}_{x}=0,{B}_{y}=0,\phantom{\rule{0.2em}{0ex}}and\phantom{\rule{0.2em}{0ex}}{B}_{z}=ax,[/latex] where a is a constant. At some time t, a wire of length L is carrying a current I is located along the x-axis from origin to x = L. Find the magnetic force on the wire at this instant in time.
Show Solution
[latex]Ia{L}^{2}\text{/}2[/latex]
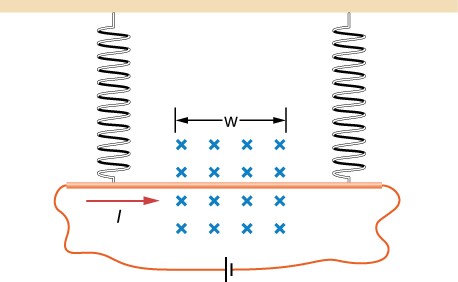
A copper rod of mass m and length L is hung from the ceiling using two springs of spring constant k. A uniform magnetic field of magnitude [latex]{B}_{0}[/latex] pointing perpendicular to the rod and spring (coming out of the page in the figure) exists in a region of space covering a length w of the copper rod. The ends of the rod are then connected by flexible copper wire across the terminals of a battery of voltage V. Determine the change in the length of the springs when a current I runs through the copper rod in the direction shown in figure. (Ignore any force by the flexible wire.)
The accompanied figure shows an arrangement for measuring mass of ions by an instrument called the mass spectrometer. An ion of mass m and charge +q is produced essentially at rest in source S, a chamber in which a gas discharge is taking place. The ion is accelerated by a potential difference [latex]{V}_{\text{acc}}[/latex] and allowed to enter a region of constant magnetic field [latex]{\stackrel{\to }{\textbf{B}}}_{0}.[/latex] In the uniform magnetic field region, the ion moves in a semicircular path striking a photographic plate at a distance x from the entry point. Derive a formula for mass m in terms of [latex]{B}_{0},[/latex] q, [latex]{V}_{\text{acc}},[/latex] and x.
Show Solution
[latex]m=\frac{q{B}_{0}{}^{2}}{8{V}_{\text{acc}}}{x}^{2}[/latex]
A wire is made into a circular shape of radius R and pivoted along a central support. The two ends of the wire are touching a brush that is connected to a dc power source. The structure is between the poles of a magnet such that we can assume there is a uniform magnetic field on the wire. In terms of a coordinate system with origin at the center of the ring, magnetic field is [latex]{B}_{x}={B}_{0},{B}_{y}={B}_{z}=0,[/latex] and the ring rotates about the z-axis. Find the torque on the ring when it is not in the xz-plane.
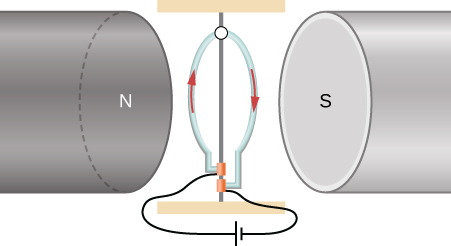
A long-rigid wire lies along the x-axis and carries a current of 2.5 A in the positive x-direction. Around the wire is the magnetic field [latex]\stackrel{\to }{\textbf{B}}=2.0\hat{\textbf{i}}+5.0{x}^{2}\hat{\textbf{j}},[/latex] with x in meters and B in millitesla. Calculate the magnetic force on the segment of wire between x = 2.0 m and x = 4.0 m.
Show Solution
0.23 N
A circular loop of wire of area 10 cm2 carries a current of 25 A. At a particular instant, the loop lies in the xy-plane and is subjected to a magnetic field [latex]\stackrel{\to }{\textbf{B}}=\left(2.0\hat{\textbf{i}}+6.0\hat{\textbf{j}}+8.0\hat{\textbf{k}}\right)\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-3}\phantom{\rule{0.2em}{0ex}}\text{T}.[/latex] As viewed from above the xy-plane, the current is circulating clockwise. (a) What is the magnetic dipole moment of the current loop? (b) At this instant, what is the magnetic torque on the loop?
Glossary
- cyclotron
- device used to accelerate charged particles to large kinetic energies
- dees
- large metal containers used in cyclotrons that serve contain a stream of charged particles as their speed is increased
- mass spectrometer
- device that separates ions according to their charge-to-mass ratios
Licenses and Attributions
Applications of Magnetic Forces and Fields. Authored by: OpenStax College. Located at: https://openstax.org/books/university-physics-volume-2/pages/11-7-applications-of-magnetic-forces-and-fields. License: CC BY: Attribution. License Terms: Download for free at https://openstax.org/books/university-physics-volume-2/pages/1-introduction
