3.6 Adiabatic Processes for an Ideal Gas

Samuel J. Ling; William Moebs; Jeff Sanny

Chapter 3. The First Law of Thermodynamics

3.6 Adiabatic Processes for an Ideal Gas

Learning Objectives

By the end of this section, you will be able to:

Define adiabatic expansion of an ideal gas
Demonstrate the qualitative difference between adiabatic and isothermal expansions

When an ideal gas is compressed adiabatically $(Q = 0),$ work is done on it and its temperature increases; in an adiabatic expansion, the gas does work and its temperature drops. Adiabatic compressions actually occur in the cylinders of a car, where the compressions of the gas-air mixture take place so quickly that there is no time for the mixture to exchange heat with its environment. Nevertheless, because work is done on the mixture during the compression, its temperature does rise significantly. In fact, the temperature increases can be so large that the mixture can explode without the addition of a spark. Such explosions, since they are not timed, make a car run poorly—it usually “knocks.” Because ignition temperature rises with the octane of gasoline, one way to overcome this problem is to use a higher-octane gasoline.

Another interesting adiabatic process is the free expansion of a gas. Figure 3.13 shows a gas confined by a membrane to one side of a two-compartment, thermally insulated container. When the membrane is punctured, gas rushes into the empty side of the container, thereby expanding freely. Because the gas expands “against a vacuum” $(p = 0)$ , it does no work, and because the vessel is thermally insulated, the expansion is adiabatic. With $Q = 0$ and $W = 0$ in the first law, $Δ E_{int} = 0,$ so $E_{int}_{i} = E_{int}_{f}$ for the free expansion.

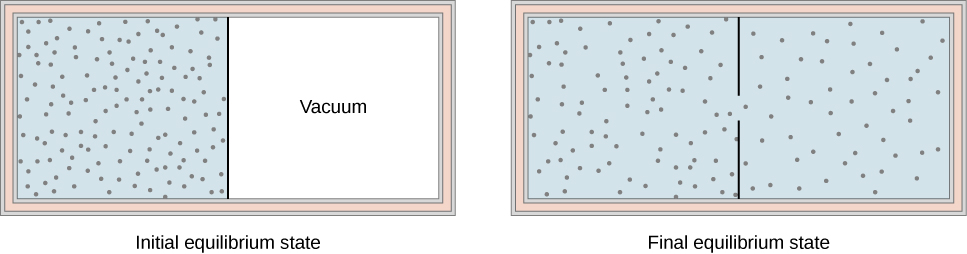
The figure on the left is an illustration of the initial equilibrium state of a container with a partition in the middle dividing it into two chambers. The outer walls are insulated. The chamber on the left is full of gas, indicated by blue shading and many small dots representing the gas molecules. The right chamber is empty. The figure on the right is an illustration of the final equilibrium state of the container. The partition has a hole in it. The entire container, on both sides of the partition, is full of gas, indicated by blue shading and many small dots representing the gas molecules. The dots in the second, final equilibrium state, illustration are less dense than in the first, initial state illustration. — **Figure 3.13** The gas in the left chamber expands freely into the right chamber when the membrane is punctured.

If the gas is ideal, the internal energy depends only on the temperature. Therefore, when an ideal gas expands freely, its temperature does not change.

A quasi-static, adiabatic expansion of an ideal gas is represented in Figure 3.14, which shows an insulated cylinder that contains 1 mol of an ideal gas. The gas is made to expand quasi-statically by removing one grain of sand at a time from the top of the piston. When the gas expands by dV, the change in its temperature is dT. The work done by the gas in the expansion is $d W = p d V; d Q = 0$ because the cylinder is insulated; and the change in the internal energy of the gas is, from Equation 3.9, $d E_{int} = C_{V} d T .$ Therefore, from the first law,

C_{V} d T = 0 - p d V = − p d V,

so

d T = - \frac{p d V}{C_{V}} .

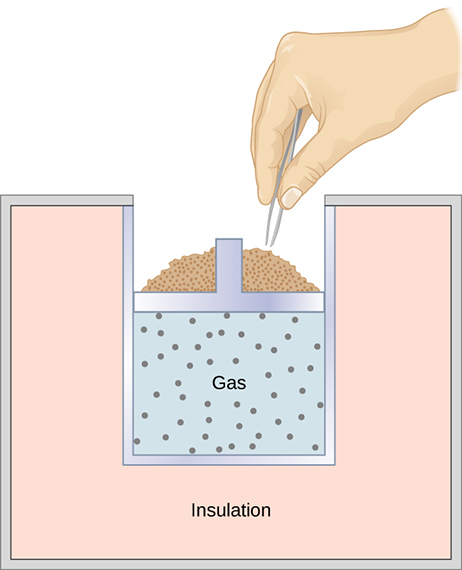
The figure is an illustration of a container. The walls and bottom are filled with a thick layer of insulation. The chamber of the container is closed from above by a piston. Inside the chamber is a gas. There is a pile of sand on top of the piston, and a hand with tweezers is removing grains from the pile. — **Figure 3.14** When sand is removed from the piston one grain at a time, the gas expands adiabatically and quasi-statically in the insulated vessel.

Also, for 1 mol of an ideal gas,

d (p V) = d (R T),

so

p d V + V d p = R d T

and

d T = \frac{p d V + V d p}{R} .

We now have two equations for dT. Upon equating them, we find that

C_{V} V d p + (C_{V} + R) p d V = 0.

Now, we divide this equation by pV and use $C_{p} = C_{V} + R$ . We are then left with

C_{V} \frac{d p}{p} + C_{p} \frac{d V}{V} = 0,

which becomes

\frac{d p}{p} + γ \frac{d V}{V} = 0,

where we define $γ$ as the ratio of the molar heat capacities:

γ = \frac{C_{p}}{C_{V}} .

Thus,

\int \frac{d p}{p} + γ \int \frac{d V}{V} = 0

and

ln p + γ ln V = constant .

Finally, using $ln (A^{x}) = x ln A and ln A B = ln A + ln B$ , we can write this in the form

p V^{γ} = constant .

This equation is the condition that must be obeyed by an ideal gas in a quasi-static adiabatic process. For example, if an ideal gas makes a quasi-static adiabatic transition from a state with pressure and volume $p_{1}$ and $V_{1}$ to a state with $p_{2}$ and $V_{2},$ then it must be true that $p_{1} V_{1}^{γ} = p_{2} V_{2}^{γ} .$

The adiabatic condition of Figure 3.12 can be written in terms of other pairs of thermodynamic variables by combining it with the ideal gas law. In doing this, we find that

p^{1 - γ} T^{γ} = constant

and

T V^{γ - 1} = constant .

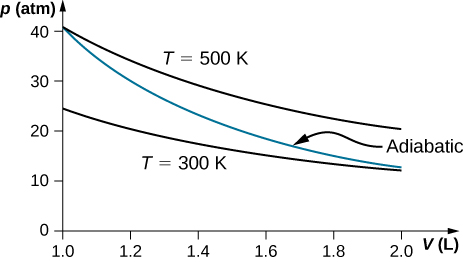
A reversible adiabatic expansion of an ideal gas is represented on the pV diagram of Figure 3.15. The slope of the curve at any point is

\frac{d p}{d V} = \frac{d}{d V} (\frac{constant}{V^{γ}}) = − γ \frac{p}{V} .

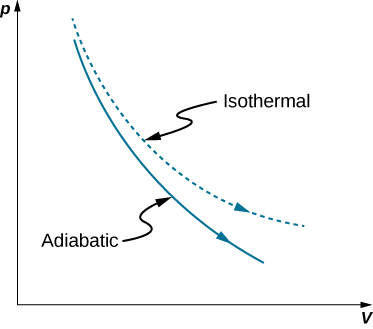
The figure is a plot of pressure, p on the vertical axis as a function of volume, V on the horizontal axis. Two curves are plotted. Both are monotonically decreasing and concave up. One is slightly higher and has a greater curvature. This curve is labeled “isothermal.” The second curve is below the isothermal curve and has a slightly smaller curvature. This curve is labeled “adiabatic.” — **Figure 3.15** Quasi-static adiabatic and isothermal expansions of an ideal gas.

The dashed curve shown on this pV diagram represents an isothermal expansion where T (and therefore pV) is constant. The slope of this curve is useful when we consider the second law of thermodynamics in the next chapter. This slope is

\frac{d p}{d V} = \frac{d}{d V} \frac{n R T}{V} = - \frac{p}{V} .

Because $γ > 1,$ the isothermal curve is not as steep as that for the adiabatic expansion.

Example

Compression of an Ideal Gas in an Automobile Engine

Gasoline vapor is injected into the cylinder of an automobile engine when the piston is in its expanded position. The temperature, pressure, and volume of the resulting gas-air mixture are $20 ° C$ , $1.00 \times 10^{5} {N/m}^{2},$ and $240 {cm}^{3}$ , respectively. The mixture is then compressed adiabatically to a volume of $40 {cm}^{3}$ . Note that in the actual operation of an automobile engine, the compression is not quasi-static, although we are making that assumption here. (a) What are the pressure and temperature of the mixture after the compression? (b) How much work is done by the mixture during the compression?

Strategy

Because we are modeling the process as a quasi-static adiabatic compression of an ideal gas, we have $p V^{γ} = constant$ and $p V = n R T$ . The work needed can then be evaluated with $W = \int_{V_{1}}^{V_{2}} p d V$ .

Solution

Show Answer

For an adiabatic compression we have

$p_{2} = p_{1} {(\frac{V_{1}}{V_{2}})}^{γ},$

so after the compression, the pressure of the mixture is

$p_{2} = (1.00 \times 10^{5} {N/m}^{2}) {(\frac{240 \times 10^{- 6} m^{3}}{40 \times 10^{- 6} m^{3}})}^{1.40} = 1.23 \times 10^{6} {N/m}^{2} .$

From the ideal gas law, the temperature of the mixture after the compression is

$\begin{array}{cc} T_{2} & = (\frac{p_{2} V_{2}}{p_{1} V_{1}}) T_{1} \\ = \frac{(1.23 \times 10^{6} {N/m}^{2}) (40 \times 10^{- 6} m^{3})}{(1.00 \times 10^{5} {N/m}^{2}) (240 \times 10^{- 6} m^{3})} \cdot 293 K \\ = 600 K = 328 ° C . \end{array}$
The work done by the mixture during the compression is

$W = \int_{V_{1}}^{V_{2}} p d V .$

With the adiabatic condition of Equation 3.12, we may write p as $K / V^{γ},$ where $K = p_{1} V_{1}^{γ} = p_{2} V_{2}^{γ} .$ The work is therefore

$\begin{array}{cc} W & = \int_{V_{1}}^{V_{2}} \frac{K}{V^{γ}} d V \\ = \frac{K}{1 - γ} (\frac{1}{V_{2}^{γ - 1}} - \frac{1}{V_{1}^{γ - 1}}) \\ = \frac{1}{1 - γ} (\frac{p_{2} V_{2}^{γ}}{V_{2}^{γ - 1}} - \frac{p_{1} V_{1}^{γ}}{V_{1}^{γ - 1}}) \\ = \frac{1}{1 - γ} (p_{2} V_{2} - p_{1} V_{1}) \\ = \frac{1}{1 - 1.40} [(1.23 \times 10^{6} {N/m}^{2}) (40 \times 10^{- 6} m^{3}) - (1.00 \times 10^{5} {N/m}^{2}) (240 \times 10^{- 6} m^{3})] \\ = - 63 J . \end{array}$

Significance

The negative sign on the work done indicates that the piston does work on the gas-air mixture. The engine would not work if the gas-air mixture did work on the piston.

Summary

A quasi-static adiabatic expansion of an ideal gas produces a steeper pV curve than that of the corresponding isotherm.
A realistic expansion can be adiabatic but rarely quasi-static.

Key Equations

Equation of state for a closed system

f (p, V, T) = 0

Net work for a finite change in volume

W = \int_{V_{1}}^{V_{2}} p d V

Internal energy of a system (average total energy)

E_{int} = \sum_{i} ({\overset{―}{K}}_{i} + {\overset{―}{U}}_{i}),

Internal energy of a monatomic ideal gas

E_{int} = n N_{A} (\frac{3}{2} k_{B} T) = \frac{3}{2} n R T

First law of thermodynamics

Δ E_{int} = Q - W

Molar heat capacity at constant pressure

C_{p} = C_{V} + R

Ratio of molar heat capacities

γ = C_{p} / C_{V}

Condition for an ideal gas in a quasi-static adiabatic process

p V^{γ} = constant

Conceptual Questions

Is it possible for $γ$ to be smaller than unity?

Show Solution

No, it is always greater than 1.

Would you expect $γ$ to be larger for a gas or a solid? Explain.

There is no change in the internal energy of an ideal gas undergoing an isothermal process since the internal energy depends only on the temperature. Is it therefore correct to say that an isothermal process is the same as an adiabatic process for an ideal gas? Explain your answer.

Show Solution

An adiabatic process has a change in temperature but no heat flow. The isothermal process has no change in temperature but has heat flow.

Does a gas do any work when it expands adiabatically? If so, what is the source of the energy needed to do this work?

Problems

A monatomic ideal gas undergoes a quasi-static adiabatic expansion in which its volume is doubled. How is the pressure of the gas changed?

Show Solution

pressure decreased by 0.31 times the original pressure

An ideal gas has a pressure of 0.50 atm and a volume of 10 L. It is compressed adiabatically and quasi-statically until its pressure is 3.0 atm and its volume is 2.8 L. Is the gas monatomic, diatomic, or polyatomic?

Pressure and volume measurements of a dilute gas undergoing a quasi-static adiabatic expansion are shown below. Plot ln p vs. V and determine $γ$ for this gas from your graph.

P (atm)	V (L)
20.0	1.0
17.0	1.1
14.0	1.3
11.0	1.5
8.0	2.0
5.0	2.6
2.0	5.2
1.0	8.4

Show Solution

;
$γ = 0.713$

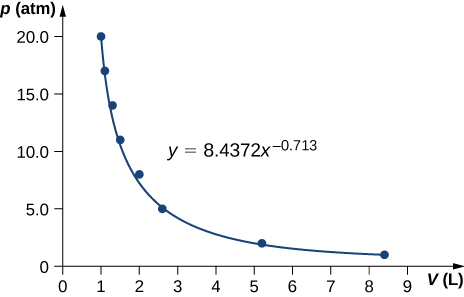
An ideal monatomic gas at 300 K expands adiabatically and reversibly to twice its volume. What is its final temperature?

An ideal diatomic gas at 80 K is slowly expanded adiabatically and reversibly to twice its volume. What is its final temperature?

Show Solution

84 K

An ideal diatomic gas at 80 K is slowly compressed adiabatically to one-third its original volume. What is its final temperature?

Compare the charge in internal energy of an ideal gas for a quasi-static adiabatic expansion with that for a quasi-static isothermal expansion. What happens to the temperature of an ideal gas in an adiabatic expansion?

Show Solution

An adiabatic expansion has less work done and no heat flow, thereby a lower internal energy comparing to an isothermal expansion which has both heat flow and work done. Temperature decreases during adiabatic expansion.

The temperature of n moles of an ideal gas changes from $T_{1}$ to $T_{2}$ in a quasi-static adiabatic transition. Show that the work done by the gas is given by

$W = \frac{n R}{γ - 1} (T_{1} - T_{2}) .$

A dilute gas expands quasi-statically to three times its initial volume. Is the final gas pressure greater for an isothermal or an adiabatic expansion? Does your answer depend on whether the gas is monatomic, diatomic, or polyatomic?

Show Solution

Isothermal has a greater final pressure and does not depend on the type of gas.

(a) An ideal gas expands adiabatically from a volume of $2.0 \times 10^{- 3} m^{3}$ to $2.5 \times 10^{- 3} m^{3}$ . If the initial pressure and temperature were $5.0 \times 10^{5} Pa$ and 300 K, respectively, what are the final pressure and temperature of the gas? Use $γ = 5 / 3$ for the gas. (b) In an isothermal process, an ideal gas expands from a volume of $2.0 \times 10^{- 3} m^{3}$ to $2.5 \times 10^{- 3} m^{3}$ . If the initial pressure and temperature were $5.0 \times 10^{5} Pa$ and 300 K, respectively, what are the final pressure and temperature of the gas?

On an adiabatic process of an ideal gas pressure, volume and temperature change such that $p V^{γ}$ is constant with $γ = 5 / 3$ for monatomic gas such as helium and $γ = 7 / 5$ for diatomic gas such as hydrogen at room temperature. Use numerical values to plot two isotherms of 1 mol of helium gas using ideal gas law and two adiabatic processes mediating between them. Use $T_{1} = 500 K, V_{1} = 1 L, and T_{2} = 300 K$ for your plot.

Show Solution

Two moles of a monatomic ideal gas such as helium is compressed adiabatically and reversibly from a state (3 atm, 5 L) to a state with pressure 4 atm. (a) Find the volume and temperature of the final state. (b) Find the temperature of the initial state of the gas. (c) Find the work done by the gas in the process. (d) Find the change in internal energy of the gas in the process.

Additional Problems

Consider the process shown below. During steps AB and BC, 3600 J and 2400 J of heat, respectively, are added to the system. (a) Find the work done in each of the processes AB, BC, AD, and DC. (b) Find the internal energy change in processes AB and BC. (c) Find the internal energy difference between states C and A. (d) Find the total heat added in the ADC process. (e) From the information given, can you find the heat added in process AD? Why or why not?

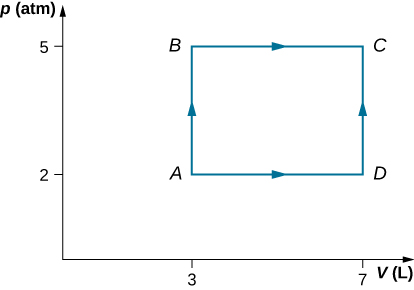
Show Solution

a. $W_{A B} = 0, W_{B C} = 2026 J, W_{A D} = 810.4 J, W_{D C} = 0;$ b. $Δ E_{A B} = 3600 J, Δ E_{B C} = 374 J;$ c. $Δ E_{A C} = 3974 J;$ d. $Q_{A D C} = 4784 J;$ e. No, because heat was added for both parts AD and DC. There is not enough information to figure out how much is from each segment of the path.

A car tire contains $0.0380 m^{3}$ of air at a pressure of $2.20 \times 10^{5} Pa$ (about 32 psi). How much more internal energy does this gas have than the same volume has at zero gauge pressure (which is equivalent to normal atmospheric pressure)?

A helium-filled toy balloon has a gauge pressure of 0.200 atm and a volume of 10.0 L. How much greater is the internal energy of the helium in the balloon than it would be at zero gauge pressure?

Show Solution

300 J

Steam to drive an old-fashioned steam locomotive is supplied at a constant gauge pressure of $1.75 \times 10^{6} {N/m}^{2}$ (about 250 psi) to a piston with a 0.200-m radius. (a) By calculating $p Δ V$ , find the work done by the steam when the piston moves 0.800 m. Note that this is the net work output, since gauge pressure is used. (b) Now find the amount of work by calculating the force exerted times the distance traveled. Is the answer the same as in part (a)?

A hand-driven tire pump has a piston with a 2.50-cm diameter and a maximum stroke of 30.0 cm. (a) How much work do you do in one stroke if the average gauge pressure is $2.4 \times 10^{5} {N/m}^{2}$ (about 35 psi)? (b) What average force do you exert on the piston, neglecting friction and gravitational force?

Show Solution

a. 59.5 J; b. 170 N

Calculate the net work output of a heat engine following path ABCDA as shown below.

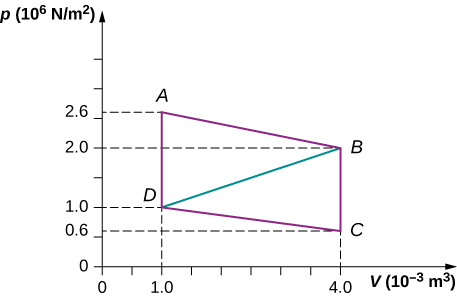
What is the net work output of a heat engine that follows path ABDA in the preceding problem with a straight line from B to D? Why is the work output less than for path ABCDA?

Show Solution

$2.4 \times 10^{3} J$

Five moles of a monatomic ideal gas in a cylinder at $27 ° C$ is expanded isothermally from a volume of 5 L to 10 L. (a) What is the change in internal energy? (b) How much work was done on the gas in the process? (c) How much heat was transferred to the gas?

Four moles of a monatomic ideal gas in a cylinder at $27 ° C$ is expanded at constant pressure equal to 1 atm until its volume doubles. (a) What is the change in internal energy? (b) How much work was done by the gas in the process? (c) How much heat was transferred to the gas?

Show Solution

a. 15,000 J; b. 10,000 J; c. 25,000 J

Helium gas is cooled from $20 ° C$ to $10 ° C$ by expanding from 40 atm to 1 atm. If there is 1.4 mol of helium, (a) What is the final volume of helium? (b) What is the change in internal energy?

In an adiabatic process, oxygen gas in a container is compressed along a path that can be described by the following pressure in atm as a function of volume V, with $V_{0} = 1 L$ : $p = (3.0 atm) {(V / V_{0})}^{- 1.2}$ . The initial and final volumes during the process were 2 L and 1.5 L, respectively. Find the amount of work done on the gas.

Show Solution

78 J

A cylinder containing three moles of a monatomic ideal gas is heated at a constant pressure of 2 atm. The temperature of the gas changes from 300 K to 350 K as a result of the expansion. Find work done (a) on the gas; and (b) by the gas.

A cylinder containing three moles of nitrogen gas is heated at a constant pressure of 2 atm. The temperature of the gas changes from 300 K to 350 K as a result of the expansion. Find work done (a) on the gas, and (b) by the gas by using van der Waals equation of state instead of ideal gas law.

Show Solution

A cylinder containing three moles of nitrogen gas is heated at a constant pressure of 2 atm. a. −1220 J; b. +1220 J

Two moles of a monatomic ideal gas such as oxygen is compressed adiabatically and reversibly from a state (3 atm, 5 L) to a state with a pressure of 4 atm. (a) Find the volume and temperature of the final state. (b) Find the temperature of the initial state. (c) Find work done by the gas in the process. (d) Find the change in internal energy in the process. Assume $C_{V} = 5 R$ and $C_{p} = C_{V} + R$ for the diatomic ideal gas in the conditions given.

An insulated vessel contains 1.5 moles of argon at 2 atm. The gas initially occupies a volume of 5 L. As a result of the adiabatic expansion the pressure of the gas is reduced to 1 atm. (a) Find the volume and temperature of the final state. (b) Find the temperature of the gas in the initial state. (c) Find the work done by the gas in the process. (d) Find the change in the internal energy of the gas in the process.

Show Solution

a. 7.6 L, 61.6 K; b. 81.3 K; c. $3.63 L \cdot atm = 367 J$ ; d. −367 J

Challenge Problems

One mole of an ideal monatomic gas occupies a volume of $1.0 \times 10^{- 2} m^{3}$ at a pressure of $2.0 \times 10^{5} {N/m}^{2} .$ (a) What is the temperature of the gas? (b) The gas undergoes a quasi-static adiabatic compression until its volume is decreased to $5.0 \times 10^{- 3} m^{3} .$ What is the new gas temperature? (c) How much work is done on the gas during the compression? (d) What is the change in the internal energy of the gas?

One mole of an ideal gas is initially in a chamber of volume $1.0 \times 10^{- 2} m^{3}$ and at a temperature of $27 ° C$ . (a) How much heat is absorbed by the gas when it slowly expands isothermally to twice its initial volume? (b) Suppose the gas is slowly transformed to the same final state by first decreasing the pressure at constant volume and then expanding it isobarically. What is the heat transferred for this case? (c) Calculate the heat transferred when the gas is transformed quasi-statically to the same final state by expanding it isobarically, then decreasing its pressure at constant volume.

Show Solution

a. 1700 J; b. 1200 J; c. 2400 J

A bullet of mass 10 g is traveling horizontally at 200 m/s when it strikes and embeds in a pendulum bob of mass 2.0 kg. (a) How much mechanical energy is dissipated in the collision? (b) Assuming that $C_{v}$ for the bob plus bullet is 3R, calculate the temperature increase of the system due to the collision. Take the molecular mass of the system to be 200 g/mol.

The insulated cylinder shown below is closed at both ends and contains an insulating piston that is free to move on frictionless bearings. The piston divides the chamber into two compartments containing gases A and B. Originally, each compartment has a volume of $5.0 \times 10^{- 2} m^{3}$ and contains a monatomic ideal gas at a temperature of $0 ° C$ and a pressure of 1.0 atm. (a) How many moles of gas are in each compartment? (b) Heat Q is slowly added to A so that it expands and B is compressed until the pressure of both gases is 3.0 atm. Use the fact that the compression of B is adiabatic to determine the final volume of both gases. (c) What are their final temperatures? (d) What is the value of Q?

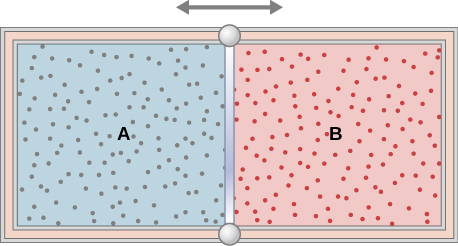
Show Solution

a. 2.2 mol; b. $V_{A} = 2.6 \times 10^{- 2} m^{3}$ , $V_{B} = 7.4 \times 10^{- 2} m^{3}$ ; c. $T_{A} = 1220 K, T_{B} = 430 K$ ; d. 30,500 J

In a diesel engine, the fuel is ignited without a spark plug. Instead, air in a cylinder is compressed adiabatically to a temperature above the ignition temperature of the fuel; at the point of maximum compression, the fuel is injected into the cylinder. Suppose that air at $20 ° C$ is taken into the cylinder at a volume $V_{1}$ and then compressed adiabatically and quasi-statically to a temperature of $600 ° C$ and a volume $V_{2} .$ If $γ = 1.4,$ what is the ratio $V_{1} / V_{2} ?$ (Note: In an operating diesel engine, the compression is not quasi-static.)

Licenses and Attributions

Adiabatic Processes for an Ideal Gas. Authored by: OpenStax College. Located at: https://openstax.org/books/university-physics-volume-2/pages/3-6-adiabatic-processes-for-an-ideal-gas. License: CC BY: Attribution. License Terms: Download for free at https://openstax.org/books/university-physics-volume-2/pages/1-introduction

License

Icon for the Creative Commons Attribution 4.0 International License

Learning Objectives

Example

Compression of an Ideal Gas in an Automobile Engine

Strategy

Solution

Significance

Summary

Key Equations

Conceptual Questions

Problems

Additional Problems

Challenge Problems

License

Share This Book