15.3 RLC Series Circuits with AC

Samuel J. Ling; William Moebs; Jeff Sanny

Chapter 15. Alternating-Current Circuits

15.3 RLC Series Circuits with AC

Learning Objectives

By the end of the section, you will be able to:

Describe how the current varies in a resistor, a capacitor, and an inductor while in series with an ac power source
Use phasors to understand the phase angle of a resistor, capacitor, and inductor ac circuit and to understand what that phase angle means
Calculate the impedance of a circuit

The ac circuit shown in Figure 15.11, called an RLC series circuit, is a series combination of a resistor, capacitor, and inductor connected across an ac source. It produces an emf of

v (t) = V_{0} sin ω t .

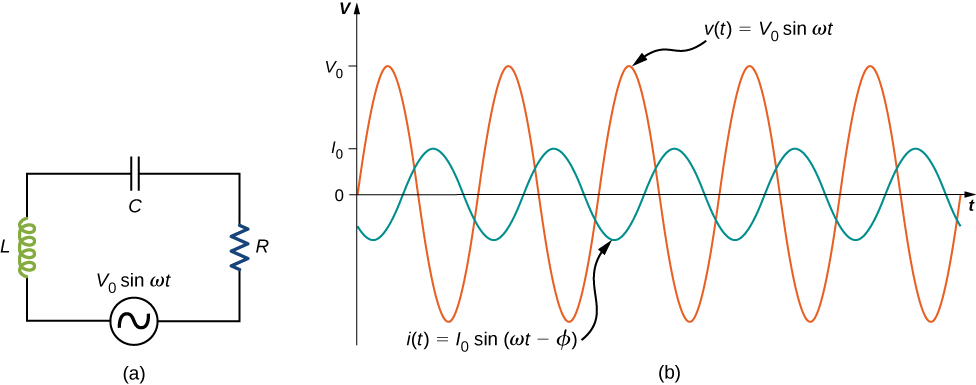
Figure a shows a circuit with an AC voltage source connected to a resistor, a capacitor and an inductor in series. The source is labeled V0 sine omega t. Figure b shows sine waves of AC voltage and current on the same graph. Voltage has a greater amplitude than current and its maximum value is marked V0 on the y axis. The maximum value of current is marked I0. The two curves have the same wavelength but are out of phase. The voltage curve is labeled V parentheses t parentheses equal to V0 sine omega t. The current curve is labeled I parentheses t parentheses equal to I0 sine parentheses omega t minus phi parentheses. — **Figure 15.11** (a) An RLC series circuit. (b) A comparison of the generator output voltage and the current. The value of the phase difference $φ$ depends on the values of R, C, and L.

Since the elements are in series, the same current flows through each element at all points in time. The relative phase between the current and the emf is not obvious when all three elements are present. Consequently, we represent the current by the general expression

i (t) = I_{0} sin (ω t - φ),

where $I_{0}$ is the current amplitude and $φ$ is the phase angle between the current and the applied voltage. The phase angle is thus the amount by which the voltage and current are out of phase with each other in a circuit. Our task is to find $I_{0} and φ .$

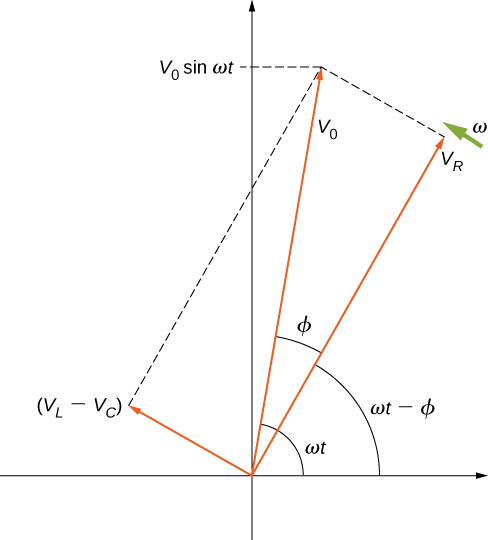
A phasor diagram involving $i (t), v_{R} (t), v_{C} (t), and v_{L} (t)$ is helpful for analyzing the circuit. As shown in Figure 15.12, the phasor representing $v_{R} (t)$ points in the same direction as the phasor for $i (t);$ its amplitude is $V_{R} = I_{0} R .$ The $v_{C} (t)$ phasor lags the i(t) phasor by $π / 2$ rad and has the amplitude $V_{C} = I_{0} X_{C} .$ The phasor for $v_{L} (t)$ leads the i(t) phasor by $π / 2$ rad and has the amplitude $V_{L} = I_{0} X_{L} .$

Figure shows the coordinate axes, with four arrows starting from the origin. Arrow V subscripts R points up and right, making an angle omega t minus phi with the x axis. Its y intercept is V subscript R parentheses t parentheses. Arrow I0 is along arrow V subscript R, but shorter than it. Arrow V subscript L points up and left and is perpendicular to V subscript R. It makes a y intercept V subscript L parentheses t parentheses. Arrow V subscript C points down and right. It is perpendicular to V subscript R. It makes a y intercept V subscript C parentheses t parentheses. Three arrows labeled omega are each perpendicular to V subscript R, V subscript L and V subscript C, shown near their tips. — **Figure 15.12** The phasor diagram for the RLC series circuit of Figure 15.11.

At any instant, the voltage across the RLC combination is $v_{R} (t) + v_{L} (t) + v_{C} (t) = v (t),$ the emf of the source. Since a component of a sum of vectors is the sum of the components of the individual vectors—for example, ${(A + B)}_{y} = A_{y} + B_{y}$ —the projection of the vector sum of phasors onto the vertical axis is the sum of the vertical projections of the individual phasors. Hence, if we add vectorially the phasors representing $v_{R} (t), v_{L} (t), and v_{C} (t)$ and then find the projection of the resultant onto the vertical axis, we obtain

v_{R} (t) + v_{L} (t) + v_{C} (t) = v (t) = V_{0} sin ω t .

The vector sum of the phasors is shown in Figure 15.13. The resultant phasor has an amplitude $V_{0}$ and is directed at an angle $φ$ with respect to the $v_{R} (t),$ or i(t), phasor. The projection of this resultant phasor onto the vertical axis is $v (t) = V_{0} sin ω t .$ We can easily determine the unknown quantities $I_{0}$ and $φ$ from the geometry of the phasor diagram. For the phase angle,

φ = {tan}^{- 1} \frac{V_{L} - V_{C}}{V_{R}} = {tan}^{- 1} \frac{I_{0} X_{L} - I_{0} X_{C}}{I_{0} R},

and after cancellation of $I_{0},$ this becomes

φ = {tan}^{- 1} \frac{X_{L} - X_{C}}{R} .

Furthermore, from the Pythagorean theorem,

V_{0} = \sqrt{V_{R}^{2} + {(V_{L} - V_{C})}^{2}} = \sqrt{{(I_{0} R)}^{2} + {(I_{0} X_{L} - I_{0} X_{C})}^{2}} = I_{0} \sqrt{R^{2} + {(X_{L} - X_{C})}^{2}} .

Three arrows start from the origin on the coordinate axis. Arrow V subscript R points up and right, making an angle omega t minus phi with the x axis. Arrow V0 points up and right, making an angle omega t with the x axis. It makes an angle phi with the arrow V subscript R. It makes a y intercept labeled V0 sine omega t. The third arrow is labeled V subscript L minus V subscript C. It points up and left and is perpendicular to arrow V subscript R. Dotted lines indicate that the rectangle formed with its longer side being V subscript R and shorter side being V subscript L minus V subscript C, would have the arrow V0 as a diagonal. An arrow labeled omega is shown near the tip of V subscript R, perpendicular to it. — **Figure 15.13** The resultant of the phasors for $v_{L} (t)$ , $v_{C} (t)$ , and $v_{R} (t)$ is equal to the phasor for $v (t) = V_{0} sin ω t .$ The i(t) phasor (not shown) is aligned with the $v_{R} (t)$ phasor.

The current amplitude is therefore the ac version of Ohm’s law:

I_{0} = \frac{V_{0}}{\sqrt{R^{2} + {(X_{L} - X_{C})}^{2}}} = \frac{V_{0}}{Z},

where

Z = \sqrt{R^{2} + {(X_{L} - X_{C})}^{2}}

is known as the impedance of the circuit. Its unit is the ohm, and it is the ac analog to resistance in a dc circuit, which measures the combined effect of resistance, capacitive reactance, and inductive reactance (Figure 15.14).

Photograph of power capacitors at a power station. — **Figure 15.14** Power capacitors are used to balance the impedance of the effective inductance in transmission lines.

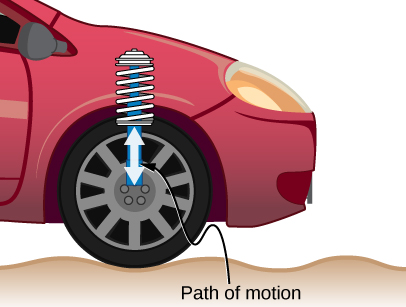
The RLC circuit is analogous to the wheel of a car driven over a corrugated road (Figure 15.15). The regularly spaced bumps in the road drive the wheel up and down; in the same way, a voltage source increases and decreases. The shock absorber acts like the resistance of the RLC circuit, damping and limiting the amplitude of the oscillation. Energy within the wheel system goes back and forth between kinetic and potential energy stored in the car spring, analogous to the shift between a maximum current, with energy stored in an inductor, and no current, with energy stored in the electric field of a capacitor. The amplitude of the wheel’s motion is at a maximum if the bumps in the road are hit at the resonant frequency, which we describe in more detail in Resonance in an AC Circuit.

Figure shows one wheel of a car. Arrows show the up-down motion of its shock absorber spring. — **Figure 15.15** On a car, the shock absorber damps motion and dissipates energy. This is much like the resistance in an RLC circuit. The mass and spring determine the resonant frequency.

Problem-Solving Strategy: AC Circuits

To analyze an ac circuit containing resistors, capacitors, and inductors, it is helpful to think of each device’s reactance and find the equivalent reactance using the rules we used for equivalent resistance in the past. Phasors are a great method to determine whether the emf of the circuit has positive or negative phase (namely, leads or lags other values). A mnemonic device of “ELI the ICE man” is sometimes used to remember that the emf (E) leads the current (I) in an inductor (L) and the current (I) leads the emf (E) in a capacitor (C).

Use the following steps to determine the emf of the circuit by phasors:

Draw the phasors for voltage across each device: resistor, capacitor, and inductor, including the phase angle in the circuit.
If there is both a capacitor and an inductor, find the net voltage from these two phasors, since they are antiparallel.
Find the equivalent phasor from the phasor in step 2 and the resistor’s phasor using trigonometry or components of the phasors. The equivalent phasor found is the emf of the circuit.

Example

An RLC Series Circuit

The output of an ac generator connected to an RLC series combination has a frequency of 200 Hz and an amplitude of 0.100 V. If $R = 4.00 Ω,$ $L = 3.00 \times 10^{- 3} H,$ and $C = 8.00 \times 10^{- 4} F,$ what are (a) the capacitive reactance, (b) the inductive reactance, (c) the impedance, (d) the current amplitude, and (e) the phase difference between the current and the emf of the generator?

Strategy

The reactances and impedance in (a)–(c) are found by substitutions into Equation 15.3, Equation 15.8, and Equation 15.11, respectively. The current amplitude is calculated from the peak voltage and the impedance. The phase difference between the current and the emf is calculated by the inverse tangent of the difference between the reactances divided by the resistance.

Solution

Show Answer

From Equation 15.3, the capacitive reactance is

$X_{C} = \frac{1}{ω C} = \frac{1}{2 π (200 Hz) (8.00 \times 10^{- 4} F)} = 0.995 Ω .$
From Equation 15.8, the inductive reactance is

$X_{L} = ω L = 2 π (200 Hz) (3.00 \times 10^{- 3} H) = 3.77 Ω .$
Substituting the values of R, $X_{C}$ , and $X_{L}$ into Equation 15.11, we obtain for the impedance

$Z = \sqrt{{(4.00 Ω)}^{2} + {(3.77 Ω - 0.995 Ω)}^{2}} = 4.87 Ω .$
The current amplitude is

$I_{0} = \frac{V_{0}}{Z} = \frac{0.100 V}{4.87 Ω} = 2.05 \times 10^{- 2} A .$
From Equation 15.9, the phase difference between the current and the emf is

$φ = {tan}^{- 1} \frac{X_{L} - X_{C}}{R} = {tan}^{- 1} \frac{2.77 Ω}{4.00 Ω} = 0.607 rad .$

Significance

The phase angle is positive because the reactance of the inductor is larger than the reactance of the capacitor.

Check Your Understanding

Find the voltages across the resistor, the capacitor, and the inductor in the circuit of Figure 15.11 using $v (t) = V_{0} sin ω t$ as the output of the ac generator.

Show Solution

$\begin{matrix} v_{R} = (V_{0} R / Z) sin (ω t - φ); v_{C} = (V_{0} X_{C} / Z) sin (ω t - φ + π / 2) = − (V_{0} X_{C} / Z) cos (ω t - φ); \\ v_{L} = (V_{0} X_{L} / Z) sin (ω t - φ + π / 2) = (V_{0} X_{L} / Z) cos (ω t - φ) \end{matrix}$

Summary

An RLC series circuit is a resistor, capacitor, and inductor series combination across an ac source.
The same current flows through each element of an RLC series circuit at all points in time.
The counterpart of resistance in a dc circuit is impedance, which measures the combined effect of resistors, capacitors, and inductors. The maximum current is defined by the ac version of Ohm’s law.
Impedance has units of ohms and is found using the resistance, the capacitive reactance, and the inductive reactance.

Conceptual Questions

In an RLC series circuit, can the voltage measured across the capacitor be greater than the voltage of the source? Answer the same question for the voltage across the inductor.

Show Solution

yes for both

Problems

What is the impedance of a series combination of a $50 -Ω$ resistor, a $5.0 - μ F$ capacitor, and a $10 - μ F$ capacitor at a frequency of 2.0 kHz?

A resistor and capacitor are connected in series across an ac generator. The emf of the generator is given by $v (t) = V_{0} cos ω t,$ where $V_{0} = 120 V,$ $ω = 120 π rad/s,$ $R = 400 Ω$ , and $C = 4.0 μ F$ . (a) What is the impedance of the circuit? (b) What is the amplitude of the current through the resistor? (c) Write an expression for the current through the resistor. (d) Write expressions representing the voltages across the resistor and across the capacitor.

Show Solution

a. $770 Ω$ ; b. 0.16 A; c. $I = (0.16 A) cos (120 π t)$ ; d. $v_{R} = 62 cos (120 π t)$ ; $v_{C} = 103 cos (120 π t - π / 2)$

A resistor and inductor are connected in series across an ac generator. The emf of the generator is given by $v (t) = V_{0} cos ω t,$ where $V_{0} = 120 V$ and $ω = 120 π rad/s;$ also, $R = 400 Ω$ and $L = 1.5 H .$ (a) What is the impedance of the circuit? (b) What is the amplitude of the current through the resistor? (c) Write an expression for the current through the resistor. (d) Write expressions representing the voltages across the resistor and across the inductor.

In an RLC series circuit, the voltage amplitude and frequency of the source are 100 V and 500 Hz, respectively, an $R = 500 Ω,$ $L = 0.20 H,$ and $C = 2.0 μ F .$ (a) What is the impedance of the circuit? (b) What is the amplitude of the current from the source? (c) If the emf of the source is given by $v (t) = (100 V) sin 1000 π t$ , how does the current vary with time? (d) Repeat the calculations with C changed to $0.20 μ F .$

Show Solution

a. $690 Ω$ ; b. 0.15 A; c. $I = (0.15 A) sin (1000 π t - 0.753)$ ; d. $1100 Ω$ , 0.092 A, $I = (0.092 A) sin (1000 π t + 1.09)$

An RLC series circuit with $R = 600 Ω$ , $L = 30 mH,$ and $C = 0.050 μ F$ is driven by an ac source whose frequency and voltage amplitude are 500 Hz and 50 V, respectively. (a) What is the impedance of the circuit? (b) What is the amplitude of the current in the circuit? (c) What is the phase angle between the emf of the source and the current?

For the circuit shown below, what are (a) the total impedance and (b) the phase angle between the current and the emf? (c) Write an expression for $i (t) .$

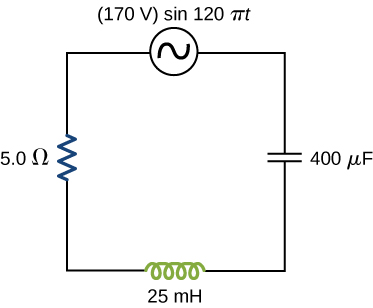
Figure shows a circuit with a voltage source 170 V, sine 120 pi t, a resistor of 5 ohm, a capacitor of 400 microfarad and an inductor of 25 milihenry all connected in series.

Show Solution

a. $5.7 Ω$ ; b. $29 °$ ; c. $I = (30. A) cos (120 π t + 0.51)$

Glossary

impedance: ac analog to resistance in a dc circuit, which measures the combined effect of resistance, capacitive reactance, and inductive reactance

phase angle: amount by which the voltage and current are out of phase with each other in a circuit

Licenses and Attributions

RLC Series Circuits with AC. Authored by: OpenStax College. Located at: https://openstax.org/books/university-physics-volume-2/pages/15-3-rlc-series-circuits-with-ac. License: CC BY: Attribution. License Terms: Download for free at https://openstax.org/books/university-physics-volume-2/pages/1-introduction

License

Icon for the Creative Commons Attribution 4.0 International License

Learning Objectives

Problem-Solving Strategy: AC Circuits

Example

An RLC Series Circuit

Strategy

Solution

Significance

Check Your Understanding

Summary

Conceptual Questions

Problems

Glossary

License

Share This Book