Chapter 6. Gauss’s Law
6.2 Explaining Gauss’s Law
Learning Objectives
By the end of this section, you will be able to:
- State Gauss’s law
- Explain the conditions under which Gauss’s law may be used
- Apply Gauss’s law in appropriate systems
We can now determine the electric flux through an arbitrary closed surface due to an arbitrary charge distribution. We found that if a closed surface does not have any charge inside where an electric field line can terminate, then any electric field line entering the surface at one point must necessarily exit at some other point of the surface. Therefore, if a closed surface does not have any charges inside the enclosed volume, then the electric flux through the surface is zero. Now, what happens to the electric flux if there are some charges inside the enclosed volume? Gauss’s law gives a quantitative answer to this question.
To get a feel for what to expect, let’s calculate the electric flux through a spherical surface around a positive point charge q, since we already know the electric field in such a situation. Recall that when we place the point charge at the origin of a coordinate system, the electric field at a point P that is at a distance r from the charge at the origin is given by
where

Then we apply
We now find the net flux by integrating this flux over the surface of the sphere:
where the total surface area of the spherical surface is
A remarkable fact about this equation is that the flux is independent of the size of the spherical surface. This can be directly attributed to the fact that the electric field of a point charge decreases as
Electric Field Lines Picture
An alternative way to see why the flux through a closed spherical surface is independent of the radius of the surface is to look at the electric field lines. Note that every field line from q that pierces the surface at radius
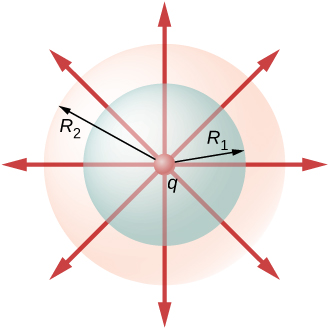
Therefore, the net number of electric field lines passing through the two surfaces from the inside to outside direction is equal. This net number of electric field lines, which is obtained by subtracting the number of lines in the direction from outside to inside from the number of lines in the direction from inside to outside gives a visual measure of the electric flux through the surfaces.
You can see that if no charges are included within a closed surface, then the electric flux through it must be zero. A typical field line enters the surface at
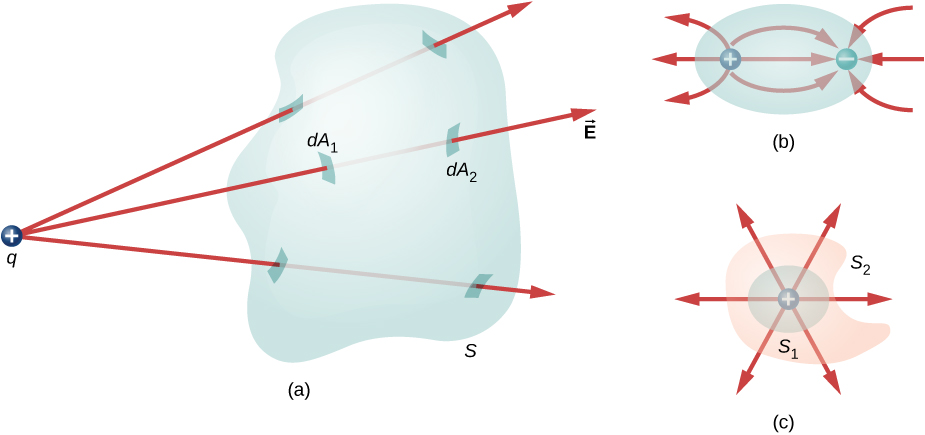
Statement of Gauss’s Law
Gauss’s law generalizes this result to the case of any number of charges and any location of the charges in the space inside the closed surface. According to Gauss’s law, the flux of the electric field
This equation holds for charges of either sign, because we define the area vector of a closed surface to point outward. If the enclosed charge is negative (see Figure 6.16(b)), then the flux through either

The Gaussian surface does not need to correspond to a real, physical object; indeed, it rarely will. It is a mathematical construct that may be of any shape, provided that it is closed. However, since our goal is to integrate the flux over it, we tend to choose shapes that are highly symmetrical.
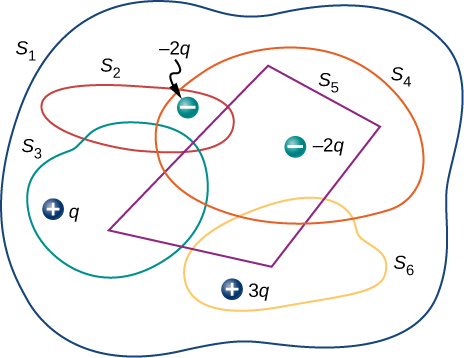
If the charges are discrete point charges, then we just add them. If the charge is described by a continuous distribution, then we need to integrate appropriately to find the total charge that resides inside the enclosed volume. For example, the flux through the Gaussian surface S of Figure 6.17 is
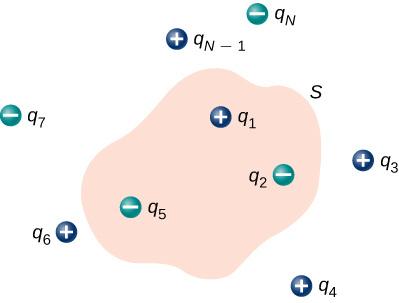
Recall that the principle of superposition holds for the electric field. Therefore, the total electric field at any point, including those on the chosen Gaussian surface, is the sum of all the electric fields present at this point. This allows us to write Gauss’s law in terms of the total electric field.
Gauss’s Law
The flux
To use Gauss’s law effectively, you must have a clear understanding of what each term in the equation represents. The field
Example
Electric Flux through Gaussian Surfaces
Calculate the electric flux through each Gaussian surface shown in Figure 6.19.
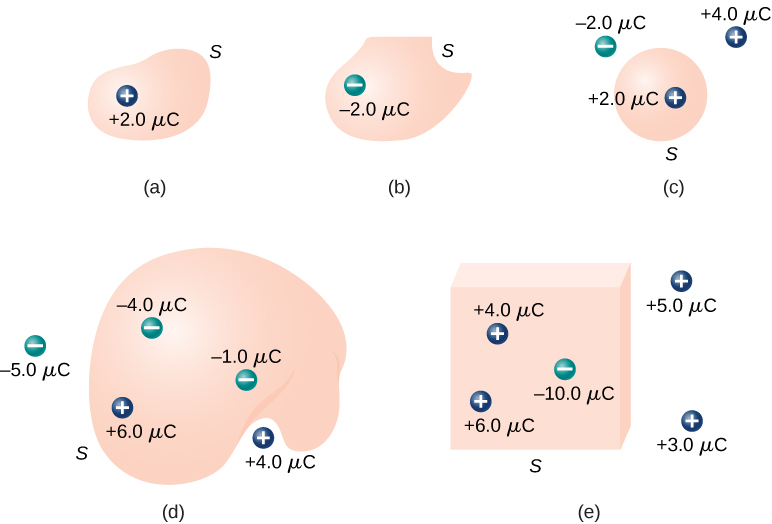
Strategy
From Gauss’s law, the flux through each surface is given by
Solution
Show Answer
For the surfaces and charges shown, we find
Significance
In the special case of a closed surface, the flux calculations become a sum of charges. In the next section, this will allow us to work with more complex systems.
Check Your Understanding
Calculate the electric flux through the closed cubical surface for each charge distribution shown in Figure 6.20.

Show Solution
a.
Use this simulation to adjust the magnitude of the charge and the radius of the Gaussian surface around it. See how this affects the total flux and the magnitude of the electric field at the Gaussian surface.
Summary
- Gauss’s law relates the electric flux through a closed surface to the net charge within that surface,
where - All surfaces that include the same amount of charge have the same number of field lines crossing it, regardless of the shape or size of the surface, as long as the surfaces enclose the same amount of charge.
Conceptual Questions
Two concentric spherical surfaces enclose a point charge q. The radius of the outer sphere is twice that of the inner one. Compare the electric fluxes crossing the two surfaces.
Show Solution
Since the electric field vector has a
Compare the electric flux through the surface of a cube of side length a that has a charge q at its center to the flux through a spherical surface of radius a with a charge q at its center.
(a) If the electric flux through a closed surface is zero, is the electric field necessarily zero at all points on the surface? (b) What is the net charge inside the surface?
Show Solution
a. no; b. zero
Discuss how Gauss’s law would be affected if the electric field of a point charge did not vary as
Discuss the similarities and differences between the gravitational field of a point mass m and the electric field of a point charge q.
Show Solution
Both fields vary as
Discuss whether Gauss’s law can be applied to other forces, and if so, which ones.
Is the term
Show Solution
No, it is produced by all charges both inside and outside the Gaussian surface.
Reformulate Gauss’s law by choosing the unit normal of the Gaussian surface to be the one directed inward.
Problems
Determine the electric flux through each surface whose cross-section is shown below.
Find the electric flux through the closed surface whose cross-sections are shown below.
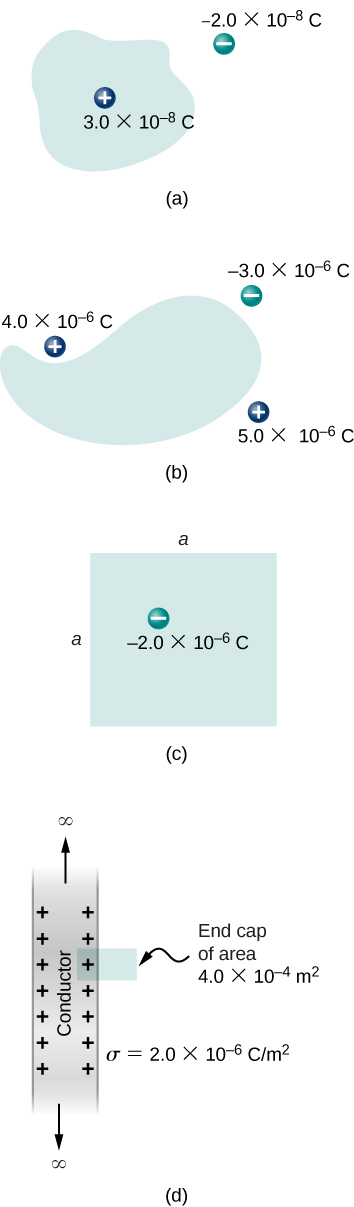
Show Solution
a.
c.
d.
A point charge q is located at the center of a cube whose sides are of length a. If there are no other charges in this system, what is the electric flux through one face of the cube?
A point charge of
Show Solution
A net flux of
A charge q is placed at one of the corners of a cube of side a, as shown below. Find the magnitude of the electric flux through the shaded face due to q. Assume
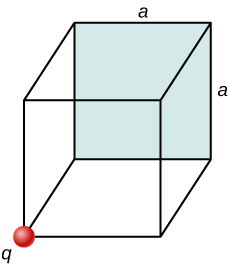
Show Solution
Make a cube with q at the center, using the cube of side a. This would take four cubes of side a to make one side of the large cube. The shaded side of the small cube would be 1/24th of the total area of the large cube; therefore, the flux through the shaded area would be
The electric flux through a cubical box 8.0 cm on a side is
The electric flux through a spherical surface is
Show Solution
A cube whose sides are of length d is placed in a uniform electric field of magnitude
Repeat the previous problem, assuming that the electric field is directed along a body diagonal of the cube.
Show Solution
zero, also because flux in equals flux out
A total charge
Glossary
- Gaussian surface
- any enclosed (usually imaginary) surface
Licenses and Attributions
Explaining Gauss’s Law. Authored by: OpenStax College. Located at: https://openstax.org/books/university-physics-volume-2/pages/6-2-explaining-gausss-law. License: CC BY: Attribution. License Terms: Download for free at https://openstax.org/books/university-physics-volume-2/pages/1-introduction

