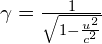Relativity
Relativistic Energy
Samuel J. Ling; Jeff Sanny; and William Moebs
Learning Objectives
By the end of this section, you will be able to:
- Explain how the work-energy theorem leads to an expression for the relativistic kinetic energy of an object
- Show how the relativistic energy relates to the classical kinetic energy, and sets a limit on the speed of any object with mass
- Describe how the total energy of a particle is related to its mass and velocity
- Explain how relativity relates to energy-mass equivalence, and some of the practical implications of energy-mass equivalence
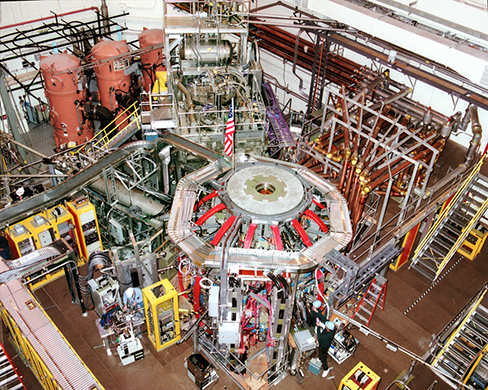
The tokamak in (Figure) is a form of experimental fusion reactor, which can change mass to energy. Nuclear reactors are proof of the relationship between energy and matter.
Conservation of energy is one of the most important laws in physics. Not only does energy have many important forms, but each form can be converted to any other. We know that classically, the total amount of energy in a system remains constant. Relativistically, energy is still conserved, but energy-mass equivalence must now be taken into account, for example, in the reactions that occur within a nuclear reactor. Relativistic energy is intentionally defined so that it is conserved in all inertial frames, just as is the case for relativistic momentum. As a consequence, several fundamental quantities are related in ways not known in classical physics. All of these relationships have been verified by experimental results and have fundamental consequences. The altered definition of energy contains some of the most fundamental and spectacular new insights into nature in recent history.
Kinetic Energy and the Ultimate Speed Limit
The first postulate of relativity states that the laws of physics are the same in all inertial frames. Einstein showed that the law of conservation of energy of a particle is valid relativistically, but for energy expressed in terms of velocity and mass in a way consistent with relativity.
Consider first the relativistic expression for the kinetic energy. We again use u for velocity to distinguish it from relative velocity v between observers. Classically, kinetic energy is related to mass and speed by the familiar expression ![]() The corresponding relativistic expression for kinetic energy can be obtained from the work-energy theorem. This theorem states that the net work on a system goes into kinetic energy. Specifically, if a force, expressed as
The corresponding relativistic expression for kinetic energy can be obtained from the work-energy theorem. This theorem states that the net work on a system goes into kinetic energy. Specifically, if a force, expressed as ![]() accelerates a particle from rest to its final velocity, the work done on the particle should be equal to its final kinetic energy. In mathematical form, for one-dimensional motion:
accelerates a particle from rest to its final velocity, the work done on the particle should be equal to its final kinetic energy. In mathematical form, for one-dimensional motion:
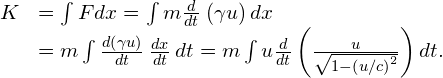
Integrate this by parts to obtain
![Rendered by QuickLaTeX.com \begin{array}{cc}\hfill K& =\frac{m{u}^{2}}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}{|}_{0u}^{}-m\int \frac{u}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}\phantom{\rule{0.2em}{0ex}}\frac{du}{dt}\phantom{\rule{0.2em}{0ex}}dt\hfill \\ & =\frac{m{u}^{2}}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}-m\int \frac{u}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}\phantom{\rule{0.2em}{0ex}}du\hfill \\ & =\frac{m{u}^{2}}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}-m{c}^{2}\left(\sqrt{1-{\left(u\text{/}c\right)}^{2}}\right){|}_{0}^{u}\hfill \\ & =\frac{m{u}^{2}}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}+\frac{m{c}^{2}}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}-m{c}^{2}\hfill \\ & =m{c}^{2}\left[\frac{\left({u}^{2}\text{/}{c}^{2}\right)+1-\left({u}^{2}\text{/}{c}^{2}\right)}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}\right]-m{c}^{2}\hfill \\ \hfill K& =\frac{m{c}^{2}}{\sqrt{1-{\left(u\text{/}c\right)}^{2}}}-m{c}^{2}.\hfill \end{array}](https://pressbooks.online.ucf.edu/app/uploads/quicklatex/quicklatex.com-283142ec533bb478babd605d3b019590_l3.png)
Relativistic kinetic energy of any particle of mass m is
When an object is motionless, its speed is ![]() and
and
so that ![]() at rest, as expected. But the expression for relativistic kinetic energy (such as total energy and rest energy) does not look much like the classical
at rest, as expected. But the expression for relativistic kinetic energy (such as total energy and rest energy) does not look much like the classical ![]() To show that the expression for
To show that the expression for ![]() reduces to the classical expression for kinetic energy at low speeds, we use the binomial expansion to obtain an approximation for
reduces to the classical expression for kinetic energy at low speeds, we use the binomial expansion to obtain an approximation for ![]() valid for small
valid for small ![]() :
:
by neglecting the very small terms in ![]() and higher powers of
and higher powers of ![]() Choosing
Choosing ![]() and
and ![]() leads to the conclusion that γ at nonrelativistic speeds, where
leads to the conclusion that γ at nonrelativistic speeds, where ![]() is small, satisfies
is small, satisfies
A binomial expansion is a way of expressing an algebraic quantity as a sum of an infinite series of terms. In some cases, as in the limit of small speed here, most terms are very small. Thus, the expression derived here for ![]() is not exact, but it is a very accurate approximation. Therefore, at low speed:
is not exact, but it is a very accurate approximation. Therefore, at low speed:
Entering this into the expression for relativistic kinetic energy gives
That is, relativistic kinetic energy becomes the same as classical kinetic energy when ![]()
It is even more interesting to investigate what happens to kinetic energy when the speed of an object approaches the speed of light. We know that ![]() becomes infinite as u approaches c, so that
becomes infinite as u approaches c, so that ![]() also becomes infinite as the velocity approaches the speed of light ((Figure)). The increase in
also becomes infinite as the velocity approaches the speed of light ((Figure)). The increase in ![]() is far larger than in
is far larger than in ![]() as v approaches c. An infinite amount of work (and, hence, an infinite amount of energy input) is required to accelerate a mass to the speed of light.
as v approaches c. An infinite amount of work (and, hence, an infinite amount of energy input) is required to accelerate a mass to the speed of light.
No object with mass can attain the speed of light.
The speed of light is the ultimate speed limit for any particle having mass. All of this is consistent with the fact that velocities less than c always add to less than c. Both the relativistic form for kinetic energy and the ultimate speed limit being c have been confirmed in detail in numerous experiments. No matter how much energy is put into accelerating a mass, its velocity can only approach—not reach—the speed of light.
c.”> c.”>
c.”>
Comparing Kinetic Energy An electron has a velocity ![]() (a) Calculate the kinetic energy in MeV of the electron. (b) Compare this with the classical value for kinetic energy at this velocity. (The mass of an electron is
(a) Calculate the kinetic energy in MeV of the electron. (b) Compare this with the classical value for kinetic energy at this velocity. (The mass of an electron is ![]() )
)
Strategy The expression for relativistic kinetic energy is always correct, but for (a), it must be used because the velocity is highly relativistic (close to c). First, we calculate the relativistic factor ![]() and then use it to determine the relativistic kinetic energy. For (b), we calculate the classical kinetic energy (which would be close to the relativistic value if v were less than a few percent of c) and see that it is not the same.
and then use it to determine the relativistic kinetic energy. For (b), we calculate the classical kinetic energy (which would be close to the relativistic value if v were less than a few percent of c) and see that it is not the same.
Solution for (a) For part (a):
- Identify the knowns:
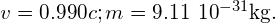
- Identify the unknown:
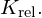
- Express the answer as an equation:
 with
with 
- Do the calculation. First calculate
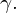 Keep extra digits because this is an intermediate calculation:
Keep extra digits because this is an intermediate calculation:

Now use this value to calculate the kinetic energy:
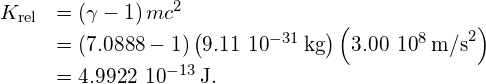
- Convert units:
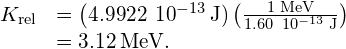
Solution for (b) For part (b):
- List the knowns:

- List the unknown:
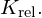
- Express the answer as an equation:

- Do the calculation:
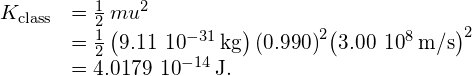
- Convert units:
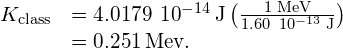
Significance As might be expected, because the velocity is 99.0% of the speed of light, the classical kinetic energy differs significantly from the correct relativistic value. Note also that the classical value is much smaller than the relativistic value. In fact, ![]() in this case. This illustrates how difficult it is to get a mass moving close to the speed of light. Much more energy is needed than predicted classically. Ever-increasing amounts of energy are needed to get the velocity of a mass a little closer to that of light. An energy of 3 MeV is a very small amount for an electron, and it can be achieved with present-day particle accelerators. SLAC, for example, can accelerate electrons to over
in this case. This illustrates how difficult it is to get a mass moving close to the speed of light. Much more energy is needed than predicted classically. Ever-increasing amounts of energy are needed to get the velocity of a mass a little closer to that of light. An energy of 3 MeV is a very small amount for an electron, and it can be achieved with present-day particle accelerators. SLAC, for example, can accelerate electrons to over ![]()
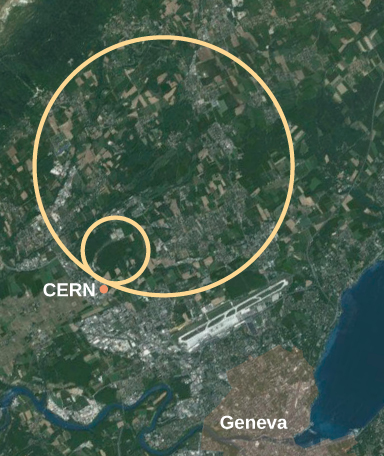
Is there any point in getting v a little closer to c than 99.0% or 99.9%? The answer is yes. We learn a great deal by doing this. The energy that goes into a high-velocity mass can be converted into any other form, including into entirely new particles. In the Large Hadron Collider in (Figure), charged particles are accelerated before entering the ring-like structure. There, two beams of particles are accelerated to their final speed of about 99.7% the speed of light in opposite directions, and made to collide, producing totally new species of particles. Most of what we know about the substructure of matter and the collection of exotic short-lived particles in nature has been learned this way. Patterns in the characteristics of these previously unknown particles hint at a basic substructure for all matter. These particles and some of their characteristics will be discussed in a later chapter on particle physics.
Total Relativistic Energy
The expression for kinetic energy can be rearranged to:
Einstein argued in a separate article, also later published in 1905, that if the energy of a particle changes by ![]() its mass changes by
its mass changes by ![]() Abundant experimental evidence since then confirms that
Abundant experimental evidence since then confirms that ![]() corresponds to the energy that the particle of mass m has when at rest. For example, when a neutral pion of mass m at rest decays into two photons, the photons have zero mass but are observed to have total energy corresponding to
corresponds to the energy that the particle of mass m has when at rest. For example, when a neutral pion of mass m at rest decays into two photons, the photons have zero mass but are observed to have total energy corresponding to ![]() for the pion. Similarly, when a particle of mass m decays into two or more particles with smaller total mass, the observed kinetic energy imparted to the products of the decay corresponds to the decrease in mass. Thus, E is the total relativistic energy of the particle, and
for the pion. Similarly, when a particle of mass m decays into two or more particles with smaller total mass, the observed kinetic energy imparted to the products of the decay corresponds to the decrease in mass. Thus, E is the total relativistic energy of the particle, and ![]() is its rest energy.
is its rest energy.
Total energyE of a particle is
where m is mass, c is the speed of light, ![]() and u is the velocity of the mass relative to an observer.
and u is the velocity of the mass relative to an observer.
Rest energy of an object is
This is the correct form of Einstein’s most famous equation, which for the first time showed that energy is related to the mass of an object at rest. For example, if energy is stored in the object, its rest mass increases. This also implies that mass can be destroyed to release energy. The implications of these first two equations regarding relativistic energy are so broad that they were not completely recognized for some years after Einstein published them in 1905, nor was the experimental proof that they are correct widely recognized at first. Einstein, it should be noted, did understand and describe the meanings and implications of his theory.
Calculating Rest Energy Calculate the rest energy of a 1.00-g mass.
Strategy One gram is a small mass—less than one-half the mass of a penny. We can multiply this mass, in SI units, by the speed of light squared to find the equivalent rest energy.
Solution
- Identify the knowns:

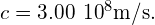
- Identify the unknown:

- Express the answer as an equation:
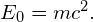
- Do the calculation:
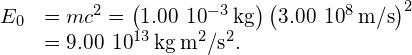
- Convert units. Noting that
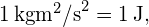 we see the rest energy is:
we see the rest energy is:
Significance This is an enormous amount of energy for a 1.00-g mass. Rest energy is large because the speed of light c is a large number and ![]() is a very large number, so that
is a very large number, so that ![]() is huge for any macroscopic mass. The
is huge for any macroscopic mass. The ![]() rest mass energy for 1.00 g is about twice the energy released by the Hiroshima atomic bomb and about 10,000 times the kinetic energy of a large aircraft carrier.
rest mass energy for 1.00 g is about twice the energy released by the Hiroshima atomic bomb and about 10,000 times the kinetic energy of a large aircraft carrier.
Today, the practical applications of the conversion of mass into another form of energy, such as in nuclear weapons and nuclear power plants, are well known. But examples also existed when Einstein first proposed the correct form of relativistic energy, and he did describe some of them. Nuclear radiation had been discovered in the previous decade, and it had been a mystery as to where its energy originated. The explanation was that, in some nuclear processes, a small amount of mass is destroyed and energy is released and carried by nuclear radiation. But the amount of mass destroyed is so small that it is difficult to detect that any is missing. Although Einstein proposed this as the source of energy in the radioactive salts then being studied, it was many years before there was broad recognition that mass could be and, in fact, commonly is, converted to energy ((Figure)).
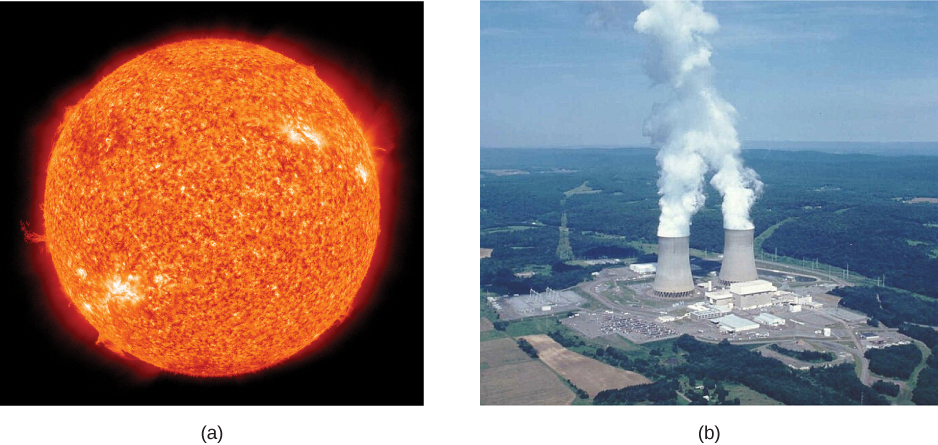
Because of the relationship of rest energy to mass, we now consider mass to be a form of energy rather than something separate. There had not been even a hint of this prior to Einstein’s work. Energy-mass equivalence is now known to be the source of the sun’s energy, the energy of nuclear decay, and even one of the sources of energy keeping Earth’s interior hot.
Stored Energy and Potential Energy
What happens to energy stored in an object at rest, such as the energy put into a battery by charging it, or the energy stored in a toy gun’s compressed spring? The energy input becomes part of the total energy of the object and thus increases its rest mass. All stored and potential energy becomes mass in a system. In seeming contradiction, the principle of conservation of mass (meaning total mass is constant) was one of the great laws verified by nineteenth-century science. Why was it not noticed to be incorrect? The following example helps answer this question.
Calculating Rest Mass A car battery is rated to be able to move 600 ampere-hours ![]() of charge at 12.0 V. (a) Calculate the increase in rest mass of such a battery when it is taken from being fully depleted to being fully charged, assuming none of the chemical reactants enter or leave the battery. (b) What percent increase is this, given that the battery’s mass is 20.0 kg?
of charge at 12.0 V. (a) Calculate the increase in rest mass of such a battery when it is taken from being fully depleted to being fully charged, assuming none of the chemical reactants enter or leave the battery. (b) What percent increase is this, given that the battery’s mass is 20.0 kg?
Strategy In part (a), we first must find the energy stored as chemical energy ![]() in the battery, which equals the electrical energy the battery can provide. Because
in the battery, which equals the electrical energy the battery can provide. Because ![]() we have to calculate the charge q in
we have to calculate the charge q in ![]() which is the product of the current I and the time t. We then multiply the result by 12.0 V. We can then calculate the battery’s increase in mass using
which is the product of the current I and the time t. We then multiply the result by 12.0 V. We can then calculate the battery’s increase in mass using ![]() Part (b) is a simple ratio converted into a percentage.
Part (b) is a simple ratio converted into a percentage.
Solution for (a)
- Identify the knowns:
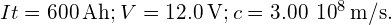
- Identify the unknown:
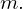
- Express the answer as an equation:
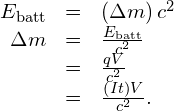
- Do the calculation:
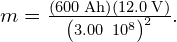
Write amperes A as coulombs per second (C/s), and convert hours into seconds:
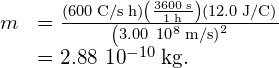
where we have used the conversion
Solution for (b) For part (b):
- Identify the knowns:
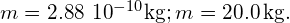
- Identify the unknown: % change.
- Express the answer as an equation:

- Do the calculation:
*** QuickLaTeX cannot compile formula: \begin{array}{cc}\hfill \text{% increase}& =\frac{\text{Δ}m}{m}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}100%\hfill \\ & =\frac{2.88\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-10}\phantom{\rule{0.2em}{0ex}}\text{kg}}{20.0\phantom{\rule{0.2em}{0ex}}\text{kg}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}100%\hfill \\ & =1.44\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-9}%.\hfill \end{array} *** Error message: File ended while scanning use of \text@. Emergency stop.
Significance Both the actual increase in mass and the percent increase are very small, because energy is divided by ![]() a very large number. We would have to be able to measure the mass of the battery to a precision of a billionth of a percent, or 1 part in
a very large number. We would have to be able to measure the mass of the battery to a precision of a billionth of a percent, or 1 part in ![]() to notice this increase. It is no wonder that the mass variation is not readily observed. In fact, this change in mass is so small that we may question how anyone could verify that it is real. The answer is found in nuclear processes in which the percentage of mass destroyed is large enough to be measured accurately. The mass of the fuel of a nuclear reactor, for example, is measurably smaller when its energy has been used. In that case, stored energy has been released (converted mostly into thermal energy to power electric generators) and the rest mass has decreased. A decrease in mass also occurs from using the energy stored in a battery, except that the stored energy is much greater in nuclear processes, making the change in mass measurable in practice as well as in theory.
to notice this increase. It is no wonder that the mass variation is not readily observed. In fact, this change in mass is so small that we may question how anyone could verify that it is real. The answer is found in nuclear processes in which the percentage of mass destroyed is large enough to be measured accurately. The mass of the fuel of a nuclear reactor, for example, is measurably smaller when its energy has been used. In that case, stored energy has been released (converted mostly into thermal energy to power electric generators) and the rest mass has decreased. A decrease in mass also occurs from using the energy stored in a battery, except that the stored energy is much greater in nuclear processes, making the change in mass measurable in practice as well as in theory.
Relativistic Energy and Momentum
We know classically that kinetic energy and momentum are related to each other, because:
Relativistically, we can obtain a relationship between energy and momentum by algebraically manipulating their defining equations. This yields:
where E is the relativistic total energy, ![]() and p is the relativistic momentum. This relationship between relativistic energy and relativistic momentum is more complicated than the classical version, but we can gain some interesting new insights by examining it. First, total energy is related to momentum and rest mass. At rest, momentum is zero, and the equation gives the total energy to be the rest energy
and p is the relativistic momentum. This relationship between relativistic energy and relativistic momentum is more complicated than the classical version, but we can gain some interesting new insights by examining it. First, total energy is related to momentum and rest mass. At rest, momentum is zero, and the equation gives the total energy to be the rest energy ![]() (so this equation is consistent with the discussion of rest energy above). However, as the mass is accelerated, its momentum p increases, thus increasing the total energy. At sufficiently high velocities, the rest energy term
(so this equation is consistent with the discussion of rest energy above). However, as the mass is accelerated, its momentum p increases, thus increasing the total energy. At sufficiently high velocities, the rest energy term ![]() becomes negligible compared with the momentum term
becomes negligible compared with the momentum term ![]() thus,
thus, ![]() at extremely relativistic velocities.
at extremely relativistic velocities.
If we consider momentum p to be distinct from mass, we can determine the implications of the equation ![]() for a particle that has no mass. If we take m to be zero in this equation, then
for a particle that has no mass. If we take m to be zero in this equation, then ![]() Massless particles have this momentum. There are several massless particles found in nature, including photons (which are packets of electromagnetic radiation). Another implication is that a massless particle must travel at speed c and only at speed c. It is beyond the scope of this text to examine the relationship in the equation
Massless particles have this momentum. There are several massless particles found in nature, including photons (which are packets of electromagnetic radiation). Another implication is that a massless particle must travel at speed c and only at speed c. It is beyond the scope of this text to examine the relationship in the equation ![]() in detail, but you can see that the relationship has important implications in special relativity.
in detail, but you can see that the relationship has important implications in special relativity.
Check Your Understanding What is the kinetic energy of an electron if its speed is 0.992c?
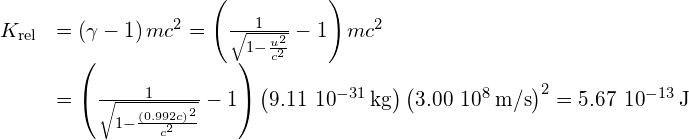
Summary
- The relativistic work-energy theorem is
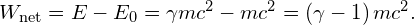
- Relativistically,
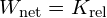 where
where 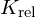 is the relativistic kinetic energy.
is the relativistic kinetic energy. - An object of mass m at velocity u has kinetic energy
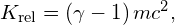 where
where 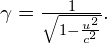
- At low velocities, relativistic kinetic energy reduces to classical kinetic energy.
- No object with mass can attain the speed of light, because an infinite amount of work and an infinite amount of energy input is required to accelerate a mass to the speed of light.
- Relativistic energy is conserved as long as we define it to include the possibility of mass changing to energy.
- The total energy of a particle with mass m traveling at speed u is defined as
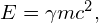 where
where 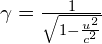 and u denotes the velocity of the particle.
and u denotes the velocity of the particle. - The rest energy of an object of mass m is
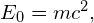 meaning that mass is a form of energy. If energy is stored in an object, its mass increases. Mass can be destroyed to release energy.
meaning that mass is a form of energy. If energy is stored in an object, its mass increases. Mass can be destroyed to release energy. - We do not ordinarily notice the increase or decrease in mass of an object because the change in mass is so small for a large increase in energy. The equation
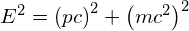 relates the relativistic total energy E and the relativistic momentum p. At extremely high velocities, the rest energy
relates the relativistic total energy E and the relativistic momentum p. At extremely high velocities, the rest energy 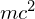 becomes negligible, and
becomes negligible, and 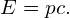
Key Equations
| Time dilation | |
| Lorentz factor | |
| Length contraction | |
| Galilean transformation | |
| Lorentz transformation | |
| Inverse Lorentz transformation | |
| Space-time invariants | |
| Relativistic velocity addition | |
| Relativistic Doppler effect for wavelength | 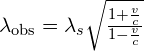 |
| Relativistic Doppler effect for frequency | 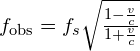 |
| Relativistic momentum | 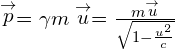 |
| Relativistic total energy | |
| Relativistic kinetic energy |
Conceptual Questions
How are the classical laws of conservation of energy and conservation of mass modified by modern relativity?
What happens to the mass of water in a pot when it cools, assuming no molecules escape or are added? Is this observable in practice? Explain.
Because it loses thermal energy, which is the kinetic energy of the random motion of its constituent particles, its mass decreases by an extremely small amount, as described by energy-mass equivalence.
Consider a thought experiment. You place an expanded balloon of air on weighing scales outside in the early morning. The balloon stays on the scales and you are able to measure changes in its mass. Does the mass of the balloon change as the day progresses? Discuss the difficulties in carrying out this experiment.
The mass of the fuel in a nuclear reactor decreases by an observable amount as it puts out energy. Is the same true for the coal and oxygen combined in a conventional power plant? If so, is this observable in practice for the coal and oxygen? Explain.
Yes, in principle there would be a similar effect on mass for any decrease in energy, but the change would be so small for the energy changes in a chemical reaction that it would be undetectable in practice.
We know that the velocity of an object with mass has an upper limit of c. Is there an upper limit on its momentum? Its energy? Explain.
Given the fact that light travels at c , can it have mass? Explain.
Not according to special relativity. Nothing with mass can attain the speed of light.
If you use an Earth-based telescope to project a laser beam onto the moon, you can move the spot across the moon’s surface at a velocity greater than the speed of light. Does this violate modern relativity? (Note that light is being sent from the Earth to the moon, not across the surface of the moon.)
Problems
What is the rest energy of an electron, given its mass is ![]() Give your answer in joules and MeV.
Give your answer in joules and MeV.
0.512 MeV according to the number of significant figures stated. The exact value is closer to 0.511 MeV.
Find the rest energy in joules and MeV of a proton, given its mass is ![]()
If the rest energies of a proton and a neutron (the two constituents of nuclei) are 938.3 and 939.6 MeV, respectively, what is the difference in their mass in kilograms?
![]() to two digits because the difference in rest mass energies is found to two digits
to two digits because the difference in rest mass energies is found to two digits
The Big Bang that began the universe is estimated to have released ![]() of energy. How many stars could half this energy create, assuming the average star’s mass is
of energy. How many stars could half this energy create, assuming the average star’s mass is ![]() ?
?
A supernova explosion of a ![]() star produces
star produces ![]() of energy. (a) How many kilograms of mass are converted to energy in the explosion? (b) What is the ratio
of energy. (a) How many kilograms of mass are converted to energy in the explosion? (b) What is the ratio ![]() of mass destroyed to the original mass of the star?
of mass destroyed to the original mass of the star?
a. ![]() b.
b. ![]()
(a) Using data from Potential Energy of a System, calculate the mass converted to energy by the fission of 1.00 kg of uranium. (b) What is the ratio of mass destroyed to the original mass, ![]()
(a) Using data from Potential Energy of a System, calculate the amount of mass converted to energy by the fusion of 1.00 kg of hydrogen. (b) What is the ratio of mass destroyed to the original mass, ![]() ? (c) How does this compare with
? (c) How does this compare with ![]() for the fission of 1.00 kg of uranium?
for the fission of 1.00 kg of uranium?
a. ![]() b.
b. ![]() c.
c. ![]() is greater for hydrogen
is greater for hydrogen
There is approximately ![]() of energy available from fusion of hydrogen in the world’s oceans. (a) If
of energy available from fusion of hydrogen in the world’s oceans. (a) If ![]() of this energy were utilized, what would be the decrease in mass of the oceans? (b) How great a volume of water does this correspond to? (c) Comment on whether this is a significant fraction of the total mass of the oceans.
of this energy were utilized, what would be the decrease in mass of the oceans? (b) How great a volume of water does this correspond to? (c) Comment on whether this is a significant fraction of the total mass of the oceans.
A muon has a rest mass energy of 105.7 MeV, and it decays into an electron and a massless particle. (a) If all the lost mass is converted into the electron’s kinetic energy, find ![]() for the electron. (b) What is the electron’s velocity?
for the electron. (b) What is the electron’s velocity?
a. 208; b. 0.999988c; six digits used to show difference from c
A ![]() -meson is a particle that decays into a muon and a massless particle. The
-meson is a particle that decays into a muon and a massless particle. The ![]() -meson has a rest mass energy of 139.6 MeV, and the muon has a rest mass energy of 105.7 MeV. Suppose the
-meson has a rest mass energy of 139.6 MeV, and the muon has a rest mass energy of 105.7 MeV. Suppose the ![]() -meson is at rest and all of the missing mass goes into the muon’s kinetic energy. How fast will the muon move?
-meson is at rest and all of the missing mass goes into the muon’s kinetic energy. How fast will the muon move?
(a) Calculate the relativistic kinetic energy of a 1000-kg car moving at 30.0 m/s if the speed of light were only 45.0 m/s. (b) Find the ratio of the relativistic kinetic energy to classical.
a. ![]() b. 1.54
b. 1.54
Alpha decay is nuclear decay in which a helium nucleus is emitted. If the helium nucleus has a mass of ![]() and is given 5.00 MeV of kinetic energy, what is its velocity?
and is given 5.00 MeV of kinetic energy, what is its velocity?
(a) Beta decay is nuclear decay in which an electron is emitted. If the electron is given 0.750 MeV of kinetic energy, what is its velocity? (b) Comment on how the high velocity is consistent with the kinetic energy as it compares to the rest mass energy of the electron.
a. 0.914c; b. The rest mass energy of an electron is 0.511 MeV, so the kinetic energy is approximately 150% of the rest mass energy. The electron should be traveling close to the speed of light.
Additional Problems
(a) At what relative velocity is ![]() (b) At what relative velocity is
(b) At what relative velocity is ![]()
(a) At what relative velocity is ![]() (b) At what relative velocity is
(b) At what relative velocity is ![]()
a. 0.866c; b. 0.995c
Unreasonable Results (a) Find the value of ![]() required for the following situation. An earthbound observer measures 23.9 h to have passed while signals from a high-velocity space probe indicate that 24.0 h have passed on board. (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?
required for the following situation. An earthbound observer measures 23.9 h to have passed while signals from a high-velocity space probe indicate that 24.0 h have passed on board. (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?
(a) How long does it take the astronaut in (Figure) to travel 4.30 ly at ![]() (as measured by the earthbound observer)? (b) How long does it take according to the astronaut? (c) Verify that these two times are related through time dilation with
(as measured by the earthbound observer)? (b) How long does it take according to the astronaut? (c) Verify that these two times are related through time dilation with ![]() as given.
as given.
a. 4.303 y to four digits to show any effect; b. 0.1434 y; c. ![]()
(a) How fast would an athlete need to be running for a 100-![]() race to look 100 yd long? (b) Is the answer consistent with the fact that relativistic effects are difficult to observe in ordinary circumstances? Explain.
race to look 100 yd long? (b) Is the answer consistent with the fact that relativistic effects are difficult to observe in ordinary circumstances? Explain.
(a) Find the value of ![]() for the following situation. An astronaut measures the length of his spaceship to be 100 m, while an earthbound observer measures it to be 25.0 m. (b) What is the speed of the spaceship relative to Earth?
for the following situation. An astronaut measures the length of his spaceship to be 100 m, while an earthbound observer measures it to be 25.0 m. (b) What is the speed of the spaceship relative to Earth?
a. 4.00; b. ![]()
A clock in a spaceship runs one-tenth the rate at which an identical clock on Earth runs. What is the speed of the spaceship?
An astronaut has a heartbeat rate of 66 beats per minute as measured during his physical exam on Earth. The heartbeat rate of the astronaut is measured when he is in a spaceship traveling at 0.5c with respect to Earth by an observer (A) in the ship and by an observer (B) on Earth. (a) Describe an experimental method by which observer B on Earth will be able to determine the heartbeat rate of the astronaut when the astronaut is in the spaceship. (b) What will be the heartbeat rate(s) of the astronaut reported by observers A and B?
a. A sends a radio pulse at each heartbeat to B, who knows their relative velocity and uses the time dilation formula to calculate the proper time interval between heartbeats from the observed signal. b. ![]()
A spaceship (A) is moving at speed c/2 with respect to another spaceship (B). Observers in A and B set their clocks so that the event at (x, y, z, t) of turning on a laser in spaceship B has coordinates (0, 0, 0, 0) in A and also (0, 0, 0, 0) in B. An observer at the origin of B turns on the laser at ![]() and turns it off at
and turns it off at ![]() in his time. What is the time duration between on and off as seen by an observer in A?
in his time. What is the time duration between on and off as seen by an observer in A?
Same two observers as in the preceding exercise, but now we look at two events occurring in spaceship A. A photon arrives at the origin of A at its time ![]() and another photon arrives at
and another photon arrives at ![]() at
at ![]() in the frame of ship A. (a) Find the coordinates and times of the two events as seen by an observer in frame B. (b) In which frame are the two events simultaneous and in which frame are they are not simultaneous?
in the frame of ship A. (a) Find the coordinates and times of the two events as seen by an observer in frame B. (b) In which frame are the two events simultaneous and in which frame are they are not simultaneous?
a. first photon: ![]() at
at ![]() second photon:
second photon:

b. simultaneous in A, not simultaneous in B
Same two observers as in the preceding exercises. A rod of length 1 m is laid out on the x-axis in the frame of B from origin to ![]() What is the length of the rod observed by an observer in the frame of spaceship A?
What is the length of the rod observed by an observer in the frame of spaceship A?
An observer at origin of inertial frame S sees a flashbulb go off at ![]() and
and ![]() at time
at time ![]() At what time and position in the S
At what time and position in the S![]() system did the flash occur, if S
system did the flash occur, if S![]() is moving along shared x-direction with S at a velocity
is moving along shared x-direction with S at a velocity ![]()
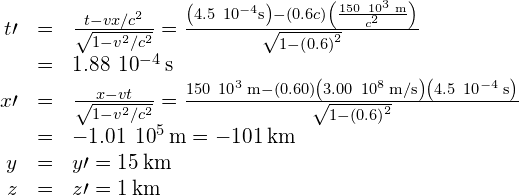
An observer sees two events ![]() apart at a separation of 800 m. How fast must a second observer be moving relative to the first to see the two events occur simultaneously?
apart at a separation of 800 m. How fast must a second observer be moving relative to the first to see the two events occur simultaneously?
An observer standing by the railroad tracks sees two bolts of lightning strike the ends of a 500-m-long train simultaneously at the instant the middle of the train passes him at 50 m/s. Use the Lorentz transformation to find the time between the lightning strikes as measured by a passenger seated in the middle of the train.
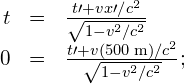
since ![]() we can ignore the term
we can ignore the term ![]() and find
and find
![]()
The breakdown of Newtonian simultaneity is negligibly small, but not exactly zero, at realistic train speeds of 50 m/s.
Two astronomical events are observed from Earth to occur at a time of 1 s apart and a distance separation of ![]() from each other. (a) Determine whether separation of the two events is space like or time like. (b) State what this implies about whether it is consistent with special relativity for one event to have caused the other?
from each other. (a) Determine whether separation of the two events is space like or time like. (b) State what this implies about whether it is consistent with special relativity for one event to have caused the other?
Two astronomical events are observed from Earth to occur at a time of 0.30 s apart and a distance separation of ![]() from each other. How fast must a spacecraft travel from the site of one event toward the other to make the events occur at the same time when measured in the frame of reference of the spacecraft?
from each other. How fast must a spacecraft travel from the site of one event toward the other to make the events occur at the same time when measured in the frame of reference of the spacecraft?
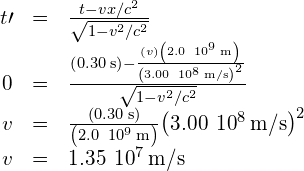
A spacecraft starts from being at rest at the origin and accelerates at a constant rate g, as seen from Earth, taken to be an inertial frame, until it reaches a speed of c/2. (a) Show that the increment of proper time is related to the elapsed time in Earth’s frame by:
(b) Find an expression for the elapsed time to reach speed c/2 as seen in Earth’s frame. (c) Use the relationship in (a) to obtain a similar expression for the elapsed proper time to reach c/2 as seen in the spacecraft, and determine the ratio of the time seen from Earth with that on the spacecraft to reach the final speed.
(a) All but the closest galaxies are receding from our own Milky Way Galaxy. If a galaxy ![]() away is receding from us at 0.900c, at what velocity relative to us must we send an exploratory probe to approach the other galaxy at 0.990c as measured from that galaxy? (b) How long will it take the probe to reach the other galaxy as measured from Earth? You may assume that the velocity of the other galaxy remains constant. (c) How long will it then take for a radio signal to be beamed back? (All of this is possible in principle, but not practical.)
away is receding from us at 0.900c, at what velocity relative to us must we send an exploratory probe to approach the other galaxy at 0.990c as measured from that galaxy? (b) How long will it take the probe to reach the other galaxy as measured from Earth? You may assume that the velocity of the other galaxy remains constant. (c) How long will it then take for a radio signal to be beamed back? (All of this is possible in principle, but not practical.)
Note that all answers to this problem are reported to five significant figures, to distinguish the results. a. 0.99947c; b.![]() c.
c.![]()
Suppose a spaceship heading straight toward the Earth at 0.750c can shoot a canister at 0.500c relative to the ship. (a) What is the velocity of the canister relative to Earth, if it is shot directly at Earth? (b) If it is shot directly away from Earth?
Repeat the preceding problem with the ship heading directly away from Earth.
a. –0.400c; b. –0.909c
If a spaceship is approaching the Earth at 0.100c and a message capsule is sent toward it at 0.100c relative to Earth, what is the speed of the capsule relative to the ship?
(a) Suppose the speed of light were only 3000 m/s. A jet fighter moving toward a target on the ground at 800 m/s shoots bullets, each having a muzzle velocity of 1000 m/s. What are the bullets’ velocity relative to the target? (b) If the speed of light was this small, would you observe relativistic effects in everyday life? Discuss.
a. 1.65 km/s; b. Yes, if the speed of light were this small, speeds that we can achieve in everyday life would be larger than 1% of the speed of light and we could observe relativistic effects much more often.
If a galaxy moving away from the Earth has a speed of 1000 km/s and emits 656 nm light characteristic of hydrogen (the most common element in the universe). (a) What wavelength would we observe on Earth? (b) What type of electromagnetic radiation is this? (c) Why is the speed of Earth in its orbit negligible here?
A space probe speeding towards the nearest star moves at ![]() and sends radio information at a broadcast frequency of 1.00 GHz. What frequency is received on Earth?
and sends radio information at a broadcast frequency of 1.00 GHz. What frequency is received on Earth?
775 MHz
Near the center of our galaxy, hydrogen gas is moving directly away from us in its orbit about a black hole. We receive 1900 nm electromagnetic radiation and know that it was 1875 nm when emitted by the hydrogen gas. What is the speed of the gas?
(a) Calculate the speed of a ![]() particle of dust that has the same momentum as a proton moving at 0.999c. (b) What does the small speed tell us about the mass of a proton compared to even a tiny amount of macroscopic matter?
particle of dust that has the same momentum as a proton moving at 0.999c. (b) What does the small speed tell us about the mass of a proton compared to even a tiny amount of macroscopic matter?
a. ![]() b. The small speed tells us that the mass of a protein is substantially smaller than that of even a tiny amount of macroscopic matter.
b. The small speed tells us that the mass of a protein is substantially smaller than that of even a tiny amount of macroscopic matter.
(a) Calculate ![]() for a proton that has a momentum of
for a proton that has a momentum of ![]() (b) What is its speed? Such protons form a rare component of cosmic radiation with uncertain origins.
(b) What is its speed? Such protons form a rare component of cosmic radiation with uncertain origins.
Show that the relativistic form of Newton’s second law is (a) ![]() (b) Find the force needed to accelerate a mass of 1 kg by 1
(b) Find the force needed to accelerate a mass of 1 kg by 1 ![]() when it is traveling at a velocity of c/2.
when it is traveling at a velocity of c/2.
a.
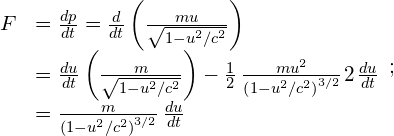
b.
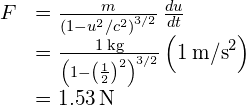
A positron is an antimatter version of the electron, having exactly the same mass. When a positron and an electron meet, they annihilate, converting all of their mass into energy. (a) Find the energy released, assuming negligible kinetic energy before the annihilation. (b) If this energy is given to a proton in the form of kinetic energy, what is its velocity? (c) If this energy is given to another electron in the form of kinetic energy, what is its velocity?
What is the kinetic energy in MeV of a π-meson that lives ![]() as measured in the laboratory, and
as measured in the laboratory, and ![]() when at rest relative to an observer, given that its rest energy is 135 MeV?
when at rest relative to an observer, given that its rest energy is 135 MeV?
90.0 MeV
Find the kinetic energy in MeV of a neutron with a measured life span of 2065 s, given its rest energy is 939.6 MeV, and rest life span is 900s.
(a) Show that ![]() This means that at large velocities
This means that at large velocities ![]() (b) Is
(b) Is ![]() when
when ![]() as for the astronaut discussed in the twin paradox?
as for the astronaut discussed in the twin paradox?
a. ![]() b. yes
b. yes
One cosmic ray neutron has a velocity of ![]() relative to the Earth. (a) What is the neutron’s total energy in MeV? (b) Find its momentum. (c) Is
relative to the Earth. (a) What is the neutron’s total energy in MeV? (b) Find its momentum. (c) Is ![]() in this situation? Discuss in terms of the equation given in part (a) of the previous problem.
in this situation? Discuss in terms of the equation given in part (a) of the previous problem.
What is ![]() for a proton having a mass energy of 938.3 MeV accelerated through an effective potential of 1.0 TV (teravolt)?
for a proton having a mass energy of 938.3 MeV accelerated through an effective potential of 1.0 TV (teravolt)?
![]()
(a) What is the effective accelerating potential for electrons at the Stanford Linear Accelerator, if ![]() for them? (b) What is their total energy (nearly the same as kinetic in this case) in GeV?
for them? (b) What is their total energy (nearly the same as kinetic in this case) in GeV?
(a) Using data from Potential Energy of a System, find the mass destroyed when the energy in a barrel of crude oil is released. (b) Given these barrels contain 200 liters and assuming the density of crude oil is ![]() what is the ratio of mass destroyed to original mass,
what is the ratio of mass destroyed to original mass, ![]()
a. ![]() b.
b. ![]() therefore,
therefore, ![]()
(a) Calculate the energy released by the destruction of 1.00 kg of mass. (b) How many kilograms could be lifted to a 10.0 km height by this amount of energy?
A Van de Graaff accelerator utilizes a 50.0 MV potential difference to accelerate charged particles such as protons. (a) What is the velocity of a proton accelerated by such a potential? (b) An electron?
a. 0.314c; b. 0.99995c (Five digits used to show difference from c)
Suppose you use an average of ![]() of electric energy per month in your home. (a) How long would 1.00 g of mass converted to electric energy with an efficiency of 38.0% last you? (b) How many homes could be supplied at the
of electric energy per month in your home. (a) How long would 1.00 g of mass converted to electric energy with an efficiency of 38.0% last you? (b) How many homes could be supplied at the ![]() per month rate for one year by the energy from the described mass conversion?
per month rate for one year by the energy from the described mass conversion?
(a) A nuclear power plant converts energy from nuclear fission into electricity with an efficiency of 35.0%. How much mass is destroyed in one year to produce a continuous 1000 MW of electric power? (b) Do you think it would be possible to observe this mass loss if the total mass of the fuel is ![]()
a. 1.00 kg; b. This much mass would be measurable, but probably not observable just by looking because it is 0.01% of the total mass.
Nuclear-powered rockets were researched for some years before safety concerns became paramount. (a) What fraction of a rocket’s mass would have to be destroyed to get it into a low Earth orbit, neglecting the decrease in gravity? (Assume an orbital altitude of 250 km, and calculate both the kinetic energy (classical) and the gravitational potential energy needed.) (b) If the ship has a mass of ![]() (100 tons), what total yield nuclear explosion in tons of TNT is needed?
(100 tons), what total yield nuclear explosion in tons of TNT is needed?
The sun produces energy at a rate of ![]() W by the fusion of hydrogen. About 0.7% of each kilogram of hydrogen goes into the energy generated by the Sun. (a) How many kilograms of hydrogen undergo fusion each second? (b) If the sun is 90.0% hydrogen and half of this can undergo fusion before the sun changes character, how long could it produce energy at its current rate? (c) How many kilograms of mass is the sun losing per second? (d) What fraction of its mass will it have lost in the time found in part (b)?
W by the fusion of hydrogen. About 0.7% of each kilogram of hydrogen goes into the energy generated by the Sun. (a) How many kilograms of hydrogen undergo fusion each second? (b) If the sun is 90.0% hydrogen and half of this can undergo fusion before the sun changes character, how long could it produce energy at its current rate? (c) How many kilograms of mass is the sun losing per second? (d) What fraction of its mass will it have lost in the time found in part (b)?
a. ![]() b.
b. ![]() c.
c. ![]() d. 0.32%
d. 0.32%
Show that ![]() for a particle is invariant under Lorentz transformations.
for a particle is invariant under Lorentz transformations.
Glossary
- relativistic kinetic energy
- kinetic energy of an object moving at relativistic speeds
- rest energy
- energy stored in an object at rest:

- speed of light
- ultimate speed limit for any particle having mass
- total energy
- sum of all energies for a particle, including rest energy and kinetic energy, given for a particle of mass m and speed u by
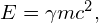 where
where