Radioactive Decay

Samuel J. Ling; Jeff Sanny; William Moebs

Nuclear Physics

Radioactive Decay

Samuel J. Ling; Jeff Sanny; and William Moebs

Learning Objectives

By the end of this section, you will be able to:

Describe the decay of a radioactive substance in terms of its decay constant and half-life
Use the radioactive decay law to estimate the age of a substance
Explain the natural processes that allow the dating of living tissue using ${}^{14}\text{C}$

In 1896, Antoine Becquerel discovered that a uranium-rich rock emits invisible rays that can darken a photographic plate in an enclosed container. Scientists offer three arguments for the nuclear origin of these rays. First, the effects of the radiation do not vary with chemical state; that is, whether the emitting material is in the form of an element or compound. Second, the radiation does not vary with changes in temperature or pressure—both factors that in sufficient degree can affect electrons in an atom. Third, the very large energy of the invisible rays (up to hundreds of eV) is not consistent with atomic electron transitions (only a few eV). Today, this radiation is explained by the conversion of mass into energy deep within the nucleus of an atom. The spontaneous emission of radiation from nuclei is called nuclear radioactivity ((Figure)).

The international ionizing radiation symbol is universally recognized as the warning symbol for nuclear radiation.

A yellow triangle with a black outline, enclosing a fan shaped structure is shown. The “fan” is black and has three blades.

Radioactive Decay Law

When an individual nucleus transforms into another with the emission of radiation, the nucleus is said to decay. Radioactive decay occurs for all nuclei with $Z>82,$ and also for some unstable isotopes with $Z<83.$ The decay rate is proportional to the number of original (undecayed) nuclei N in a substance. The number of nuclei lost to decay, $\text{−}dN$ in time interval dt, is written

$-\frac{dN}{dt}=\lambda N$

where $\lambda$ is called the decay constant. (The minus sign indicates the number of original nuclei decreases over time.) In other words, the more nuclei available to decay, the more that do decay (in time dt). This equation can be rewritten as

$\frac{dN}{N}=\text{−}\lambda dt.$

Integrating both sides of the equation, and defining ${N}_{0}$ to be the number of nuclei at $t=0$ , we obtain

${\int }_{{N}_{0}}^{N}\frac{d{N}^{\prime }}{N}=\text{−}{\int }_{0}^{t}\lambda d{t}^{\prime }.$

This gives us

$\text{ln}\phantom{\rule{0.2em}{0ex}}\frac{N}{{N}_{0}}=\text{−}\lambda t.$

Taking the left and right sides of the equation as a power of e, we have the radioactive decay law.

Radioactive Decay Law

The total number N of radioactive nuclei remaining after time t is

$N={N}_{0}{e}^{\text{−}\lambda t}$

where $\lambda$ is the decay constant for the particular nucleus.

The total number of nuclei drops very rapidly at first, and then more slowly ((Figure)).

A plot of the radioactive decay law demonstrates that the number of nuclei remaining in a decay sample drops dramatically during the first moments of decay.

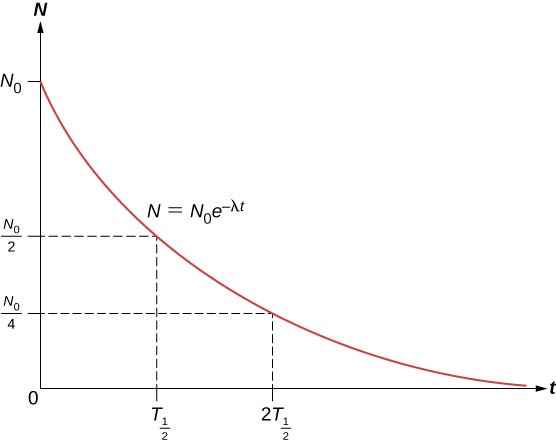
A graph of N versus t is shown. It is labeled N equal to N subscript 0 e to the power minus lambda t. The value of N is maximum, N subscript 0, at t =0 and it reduces with time till it reaches 0. At t = T subscript half, N = N subscript 0 by 2 and at t = 2T subscript half, N = N subscript 0 by 4.

The half-life $\left({T}_{1\text{/}2}\right)$ of a radioactive substance is defined as the time for half of the original nuclei to decay (or the time at which half of the original nuclei remain). The half-lives of unstable isotopes are shown in the chart of nuclides in (Figure). The number of radioactive nuclei remaining after an integer (n) number of half-lives is therefore

$N=\frac{{N}_{0}}{{2}^{n}}$

If the decay constant ( $\lambda$ ) is large, the half-life is small, and vice versa. To determine the relationship between these quantities, note that when $t={T}_{1\text{/}2}$ , then $N={N}_{0}\text{/}2$ . Thus, (Figure) can be rewritten as

$\frac{{N}_{0}}{2}={N}_{0}{e}^{\text{−}\lambda {T}_{1\text{/}2}}.$

Dividing both sides by ${N}_{0}$ and taking the natural logarithm yields

$\text{ln}\phantom{\rule{0.2em}{0ex}}\frac{1}{2}=\text{ln}\phantom{\rule{0.2em}{0ex}}{e}^{\text{−}\lambda {T}_{1\text{/}2}}$

which reduces to

$\lambda =\frac{0.693}{{T}_{1\text{/}2}}.$

Thus, if we know the half-life T_1/2 of a radioactive substance, we can find its decay constant. The lifetime $\stackrel{-}{T}$ of a radioactive substance is defined as the average amount of time that a nucleus exists before decaying. The lifetime of a substance is just the reciprocal of the decay constant, written as

$\stackrel{-}{T}=\frac{1}{\text{λ}}.$

The activity A is defined as the magnitude of the decay rate, or

$A=-\frac{dN}{dt}=\lambda N=\lambda {N}_{0}{e}^{\text{−}\lambda t}.$

The infinitesimal change dN in the time interval dt is negative because the number of parent (undecayed) particles is decreasing, so the activity (A) is positive. Defining the initial activity as ${A}_{0}=\lambda {N}_{0}$ , we have

$A={A}_{0}{e}^{\text{−}\lambda t}.$

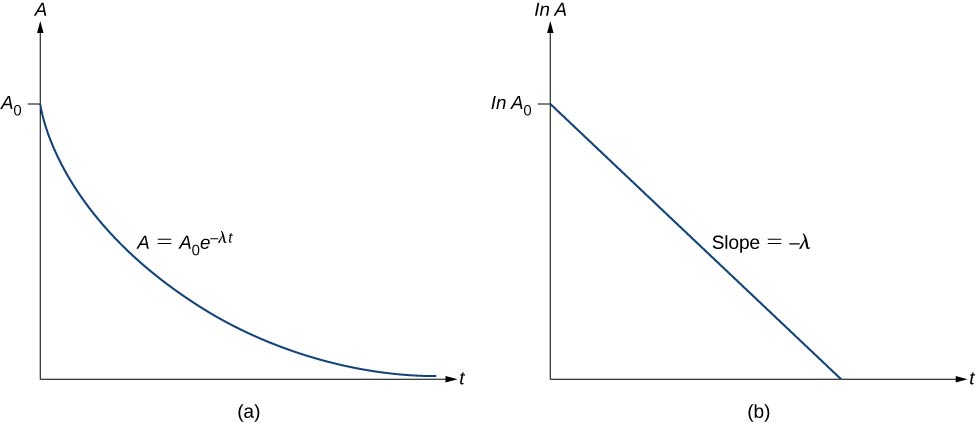
Thus, the activity A of a radioactive substance decreases exponentially with time ((Figure)).

(a) A plot of the activity as a function of time (b) If we measure the activity at different times, we can plot ln A versus t, and obtain a straight line.

Decay Constant and Activity of Strontium-90 The half-life of strontium-90, ${}_{38}^{90}\text{Sr}$ , is 28.8 y. Find (a) its decay constant and (b) the initial activity of 1.00 g of the material.

Strategy We can find the decay constant directly from (Figure). To determine the activity, we first need to find the number of nuclei present.

Solution

The decay constant is found to be

$\lambda =\frac{0.693}{{T}_{1\text{/}2}}=\left(\frac{0.693}{{T}_{1\text{/}2}}\right)\left(\frac{1\phantom{\rule{0.2em}{0ex}}\text{yr}}{3.16\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{7}\phantom{\rule{0.2em}{0ex}}\text{s}}\right)=7.61\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-10}\phantom{\rule{0.2em}{0ex}}{\text{s}}^{\text{−}1}.$
The atomic mass of ${}_{38}^{90}\text{S}\text{r}$ is 89.91 g. Using Avogadro’s number ${N}_{A}=6.022\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{23}$ atoms/mol, we find the initial number of nuclei in 1.00 g of the material:

${N}_{0}=\frac{1.00\phantom{\rule{0.2em}{0ex}}\text{g}}{89.91\phantom{\rule{0.2em}{0ex}}\text{g}}\left({N}_{A}\right)=6.70\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{21}\phantom{\rule{0.2em}{0ex}}\text{nuclei}.$

From this, we find that the activity ${A}_{0}$ at $t=0$ for 1.00 g of strontium-90 is

$\begin{array}{cc}\hfill {A}_{0}& =\lambda {N}_{0}\hfill \\ & =\left(7.61\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-10}\phantom{\rule{0.2em}{0ex}}{\text{s}}^{-1}\right)\left(6.70\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{21}\text{nuclei}\right)\hfill \\ & =5.10\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{12}\phantom{\rule{0.2em}{0ex}}\text{decays/s}.\hfill \end{array}$

Expressing $\lambda$ in terms of the half-life of the substance, we get

$A={A}_{0}{e}^{\text{−}\left(0.693\text{/}{T}_{1\text{/}2}\right){T}_{1\text{/}2}}={A}_{0}{e}^{-0.693}={A}_{0}\text{/}2.$

Therefore, the activity is halved after one half-life. We can determine the decay constant $\lambda$ by measuring the activity as a function of time. Taking the natural logarithm of the left and right sides of (Figure), we get

$\text{ln}\phantom{\rule{0.2em}{0ex}}A=\text{−}\lambda t+\text{ln}\phantom{\rule{0.2em}{0ex}}{A}_{0}.$

This equation follows the linear form $y=mx+b$ . If we plot ln A versus t, we expect a straight line with slope $\text{−}\lambda$ and y-intercept $\text{ln}\phantom{\rule{0.2em}{0ex}}{A}_{0}$ ((Figure)(b)). Activity A is expressed in units of becquerels (Bq), where one $1\phantom{\rule{0.2em}{0ex}}\text{Bq}=1\phantom{\rule{0.2em}{0ex}}\text{decay per second}$ . This quantity can also be expressed in decays per minute or decays per year. One of the most common units for activity is the curie (Ci), defined to be the activity of 1 g of ${}^{226}\text{Ra}$ . The relationship between the Bq and Ci is

$1\phantom{\rule{0.2em}{0ex}}\text{Ci}=3.70\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{10}\text{Bq}.$

What is ${}^{14}\text{C}$ Activity in Living Tissue? Approximately

*** QuickLaTeX cannot compile formula:
20\text{%}

*** Error message:
File ended while scanning use of \text@.
Emergency stop.

of the human body by mass is carbon. Calculate the activity due to ${}^{14}\text{C}$ in 1.00 kg of carbon found in a living organism. Express the activity in units of Bq and Ci.

Strategy The activity of ${}^{14}\text{C}$ is determined using the equation ${A}_{0}=\lambda {N}_{0}$ , where λ is the decay constant and ${N}_{0}$ is the number of radioactive nuclei. The number of ${}^{14}\text{C}$ nuclei in a 1.00-kg sample is determined in two steps. First, we determine the number of ${}^{12}\text{C}$ nuclei using the concept of a mole. Second, we multiply this value by $1.3\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-12}$ (the known abundance of ${}^{14}\text{C}$ in a carbon sample from a living organism) to determine the number of ${}^{14}\text{C}$ nuclei in a living organism. The decay constant is determined from the known half-life of ${}^{14}\text{C}$ (available from (Figure)).

Solution One mole of carbon has a mass of 12.0 g, since it is nearly pure ${}^{12}\text{C}$ . Thus, the number of carbon nuclei in a kilogram is

$N\left({}^{12}\text{C}\right)=\frac{6.02\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{23}\phantom{\rule{0.2em}{0ex}}{\text{mol}}^{-1}}{12.0\phantom{\rule{0.2em}{0ex}}\text{g/mol}}\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}\left(1000\phantom{\rule{0.2em}{0ex}}\text{g}\right)=5.02\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{25}.$

The number of ${}^{14}\text{C}$ nuclei in 1 kg of carbon is therefore

$N\left({}^{14}\text{C}\right)=\left(5.02\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{25}\right)\left(1.3\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-12}\right)=6.52\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{13}.$

Now we can find the activity A by using the equation $A=\frac{0.693\phantom{\rule{0.2em}{0ex}}N}{{t}_{1\text{/}2}}.$ Entering known values gives us

$A=\frac{0.693\left(6.52\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{13}\right)}{5730\phantom{\rule{0.2em}{0ex}}\text{y}}=7.89\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{9}\phantom{\rule{0.2em}{0ex}}{\text{y}}^{\text{−}1}$

or $7.89\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{9}$ decays per year. To convert this to the unit Bq, we simply convert years to seconds. Thus,

$A=\left(7.89\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{9}\phantom{\rule{0.2em}{0ex}}{\text{y}}^{\text{−}1}\right)\frac{1.00\phantom{\rule{0.2em}{0ex}}\text{y}}{3.16\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{7}\phantom{\rule{0.2em}{0ex}}\text{s}}=250\phantom{\rule{0.2em}{0ex}}\text{Bq},$

or 250 decays per second. To express A in curies, we use the definition of a curie,

$A=\frac{250\phantom{\rule{0.2em}{0ex}}\text{Bq}}{3.7\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{10}\phantom{\rule{0.2em}{0ex}}\text{Bq/Ci}}\phantom{\rule{0.2em}{0ex}}=6.76\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-9}\phantom{\rule{0.2em}{0ex}}\text{Ci}.$

Thus,

$A=6.76\phantom{\rule{0.2em}{0ex}}\text{nCi}.$

Significance Approximately

*** QuickLaTeX cannot compile formula:
20\text{%}

*** Error message:
File ended while scanning use of \text@.
Emergency stop.

of the human body by weight is carbon. Hundreds of ${}^{14}\text{C}$ decays take place in the human body every second. Carbon-14 and other naturally occurring radioactive substances in the body compose a person’s background exposure to nuclear radiation. As we will see later in this chapter, this activity level is well below the maximum recommended dosages.

Radioactive Dating

Radioactive dating is a technique that uses naturally occurring radioactivity to determine the age of a material, such as a rock or an ancient artifact. The basic approach is to estimate the original number of nuclei in a material and the present number of nuclei in the material (after decay), and then use the known value of the decay constant $\lambda$ and (Figure) to calculate the total time of the decay, t.

An important method of radioactive dating is carbon-14 dating. Carbon-14 nuclei are produced when high-energy solar radiation strikes ${}^{14}\text{N}$ nuclei in the upper atmosphere and subsequently decay with a half-life of 5730 years. Radioactive carbon has the same chemistry as stable carbon, so it combines with the ecosphere and eventually becomes part of every living organism. Carbon-14 has an abundance of 1.3 parts per trillion of normal carbon. Therefore, if you know the number of carbon nuclei in an object, you multiply that number by $1.3\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-12}$ to find the number of ${}^{14}\text{C}$ nuclei in that object. When an organism dies, carbon exchange with the environment ceases, and ${}^{14}\text{C}$ is not replenished as it decays.

By comparing the abundance of ${}^{14}\text{C}$ in an artifact, such as mummy wrappings, with the normal abundance in living tissue, it is possible to determine the mummy’s age (or the time since the person’s death). Carbon-14 dating can be used for biological tissues as old as 50,000 years, but is generally most accurate for younger samples, since the abundance of ${}^{14}\text{C}$ nuclei in them is greater. Very old biological materials contain no ${}^{14}\text{C}$ at all. The validity of carbon dating can be checked by other means, such as by historical knowledge or by tree-ring counting.

An Ancient Burial Cave In an ancient burial cave, your team of archaeologists discovers ancient wood furniture. Only

*** QuickLaTeX cannot compile formula:
80\text{%}

*** Error message:
File ended while scanning use of \text@.
Emergency stop.

of the original ${}^{14}\text{C}$ remains in the wood. How old is the furniture?

Strategy The problem statement implies that $N\text{/}{N}_{0}=0.80.$ Therefore, the equation $N={N}_{0}{e}^{\text{−}\lambda t}$ can be used to find the product, $\lambda t$ . We know the half-life of ${}^{14}\text{C}$ is 5730 y, so we also know the decay constant, and therefore the total decay time t.

Solution Solving the equation $N={N}_{0}{e}^{\text{−}\lambda t}$ for $N\text{/}{N}_{0}$ gives us

$\frac{N}{{N}_{0}}={e}^{\text{−}\lambda t}.$

Thus,

$0.80={e}^{\text{−}\lambda t}.$

Taking the natural logarithm of both sides of the equation yields

$\text{ln}\phantom{\rule{0.2em}{0ex}}0.80=-\lambda t,$

so that

$-0.223=\text{−}\lambda t.$

Rearranging the equation to isolate t gives us

$t=\frac{0.223}{\lambda },$

where

$\lambda =\frac{0.693}{{t}_{1\text{/}2}}=\frac{0.693}{5730\phantom{\rule{0.2em}{0ex}}\text{y}}.$

Combining this information yields

$t=\frac{0.223}{\left(\frac{0.693}{5730\phantom{\rule{0.2em}{0ex}}\text{y}}\right)}=1844\phantom{\rule{0.2em}{0ex}}\text{y}.$

Significance The furniture is almost 2000 years old—an impressive discovery. The typical uncertainty on carbon-14 dating is about

*** QuickLaTeX cannot compile formula:
5\text{%}

*** Error message:
File ended while scanning use of \text@.
Emergency stop.

, so the furniture is anywhere between 1750 and 1950 years old. This date range must be confirmed by other evidence, such as historical records.

Check Your Understanding A radioactive nuclide has a high decay rate. What does this mean for its half-life and activity?

Half-life is inversely related to decay rate, so the half-life is short. Activity depends on both the number of decaying particles and the decay rate, so the activity can be great or small.

Visit the Radioactive Dating Game to learn about the types of radiometric dating and try your hand at dating some ancient objects.

Summary

In the decay of a radioactive substance, if the decay constant ( $\lambda$ ) is large, the half-life is small, and vice versa.
The radioactive decay law, $N={N}_{0}{e}^{\text{−}\lambda t},$ uses the properties of radioactive substances to estimate the age of a substance.
Radioactive carbon has the same chemistry as stable carbon, so it mixes into the ecosphere and eventually becomes part of every living organism. By comparing the abundance of ${}^{14}\text{C}$ in an artifact with the normal abundance in living tissue, it is possible to determine the artifact’s age.

Conceptual Questions

How is the initial activity rate of a radioactive substance related to its half-life?

For the carbon dating described in this chapter, what important assumption is made about the time variation in the intensity of cosmic rays?

That it is constant.

Problems

A sample of radioactive material is obtained from a very old rock. A plot lnA verses t yields a slope value of $\text{−}{10}^{-9}{\text{s}}^{-1}$ (see (Figure)(b)). What is the half-life of this material?

The decay constant is equal to the negative value of the slope or ${10}^{-9}{\text{s}}^{-1}.$ The half-life of the nuclei, and thus the material, is ${T}_{1\text{/}2}=693\phantom{\rule{0.2em}{0ex}}\text{million years}.$

Show that: $\stackrel{-}{T}=\frac{1}{\text{λ}}$ .

The half-life of strontium-91, ${}_{38}^{91}\text{S}\text{r}$ is 9.70 h. Find (a) its decay constant and (b) for an initial 1.00-g sample, the activity after 15 hours.

a. The decay constant is $\lambda =1.99\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}\phantom{\rule{0.2em}{0ex}}{\text{s}}^{\text{−}1}$ . b. Since strontium-91 has an atomic mass of 90.90 g, the number of nuclei in a 1.00-g sample is initially
${N}_{0}=6.63\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{21}\phantom{\rule{0.2em}{0ex}}\text{nuclei}.$
The initial activity for strontium-91 is
$\begin{array}{cc}\hfill {A}_{0}& =\lambda {N}_{0}\hfill \\ & =1.32\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{17}\phantom{\rule{0.2em}{0ex}}\text{decays/s}\hfill \end{array}$
The activity at $t=15.0\phantom{\rule{0.2em}{0ex}}\text{h}=5.40\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{4}\phantom{\rule{0.2em}{0ex}}\text{s}$ is
$A=4.51\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{16}\phantom{\rule{0.2em}{0ex}}\text{decays/s}.$

A sample of pure carbon-14 $\left({T}_{1\text{/}2}{}_{}=5730\phantom{\rule{0.2em}{0ex}}\text{y}\right)$ has an activity of $1.0\phantom{\rule{0.2em}{0ex}}\mu \phantom{\rule{0.2em}{0ex}}\text{Ci}.$ What is the mass of the sample?

A radioactive sample initially contains $2.40\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{\text{−}2}$ mol of a radioactive material whose half-life is 6.00 h. How many moles of the radioactive material remain after 6.00 h? After 12.0 h? After 36.0 h?

$1.20\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{\text{−}2}\phantom{\rule{0.2em}{0ex}}\text{mol}$ ; $6.00\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{\text{−}3}\text{mol}$ ; $3.75\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{\text{−}4}\text{mol}$

An old campfire is uncovered during an archaeological dig. Its charcoal is found to contain less than 1/1000 the normal amount of ${}^{14}\text{C}$ . Estimate the minimum age of the charcoal, noting that ${2}^{10}=1024.$

Calculate the activity $R$ , in curies of 1.00 g of ${}^{226}\text{R}\text{a}.$ (b) Explain why your answer is not exactly 1.00 Ci, given that the curie was originally supposed to be exactly the activity of a gram of radium.

a. 0.988 Ci; b. The half-life of ${}^{226}\text{R}\text{a}$ is more precisely known than it was when the Ci unit was established.

Natural uranium consists of ${}^{235}\text{U}$

*** QuickLaTeX cannot compile formula:
\left(\text{percent abundance}=0.7200\text{%}

*** Error message:
File ended while scanning use of \text@.
Emergency stop.

, $\lambda =3.12\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-17}\text{/}\text{s}\right)$ and ${}^{238}\text{U}$

*** QuickLaTeX cannot compile formula:
\left(\text{percent abundance}=99.27\text{%}

*** Error message:
File ended while scanning use of \text@.
Emergency stop.

, $\lambda =4.92\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-18}\text{/}\text{s}\right).$ What were the values for percent abundance of ${}^{235}\text{U}$ and ${}^{238}\text{U}$ when Earth formed $4.5\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{9}$ years ago?

World War II aircraft had instruments with glowing radium-painted dials. The activity of one such instrument was $1.0\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}$ Bq when new. (a) What mass of ${}^{226}\text{R}\text{a}$ was present? (b) After some years, the phosphors on the dials deteriorated chemically, but the radium did not escape. What is the activity of this instrument 57.0 years after it was made?

a. $2.73\mu \text{g}$ ; b. $9.76\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{4}\phantom{\rule{0.2em}{0ex}}\text{Bq}$

The ${}^{210}\text{P}\text{o}$ source used in a physics laboratory is labeled as having an activity of $1.0\phantom{\rule{0.2em}{0ex}}\text{μCi}$ on the date it was prepared. A student measures the radioactivity of this source with a Geiger counter and observes 1500 counts per minute. She notices that the source was prepared 120 days before her lab. What fraction of the decays is she observing with her apparatus?

Armor-piercing shells with depleted uranium cores are fired by aircraft at tanks. (The high density of the uranium makes them effective.) The uranium is called depleted because it has had its ${}^{235}\text{U}$ removed for reactor use and is nearly pure ${}^{238}\text{U}$ . Depleted uranium has been erroneously called nonradioactive. To demonstrate that this is wrong: (a) Calculate the activity of 60.0 g of pure ${}^{238}\text{U}$ . (b) Calculate the activity of 60.0 g of natural uranium, neglecting the ${}^{234}\text{U}$ and all daughter nuclides.

a. $7.46\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\phantom{\rule{0.2em}{0ex}}\text{Bq}$ ; b. $7.75\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{5}\phantom{\rule{0.2em}{0ex}}\text{Bq}$

Glossary

activity: magnitude of the decay rate for radioactive nuclides

becquerel (Bq): SI unit for the decay rate of a radioactive material, equal to 1 decay/second

carbon-14 dating: method to determine the age of formerly living tissue using the ratio ${}^{14}\text{C/}{}^{12}\text{C}$

curie (Ci): unit of decay rate, or the activity of 1 g of ${}^{226}\text{Ra}$ , equal to $3.70\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{10}\phantom{\rule{0.2em}{0ex}}\text{Bq}$

decay: process by which an individual atomic nucleus of an unstable atom loses mass and energy by emitting ionizing particles

decay constant: quantity that is inversely proportional to the half-life and that is used in equation for number of nuclei as a function of time

half-life: time for half of the original nuclei to decay (or half of the original nuclei remain)

lifetime: average time that a nucleus exists before decaying

radioactive dating: application of radioactive decay in which the age of a material is determined by the amount of radioactivity of a particular type that occurs

radioactive decay law: describes the exponential decrease of parent nuclei in a radioactive sample

radioactivity: spontaneous emission of radiation from nuclei

License

Icon for the Creative Commons Attribution 4.0 International License

Learning Objectives

Radioactive Decay Law

Radioactive Dating

Summary

Conceptual Questions

Problems

Glossary

License

Share This Book