Diffraction

Intensity in Single-Slit Diffraction

Samuel J. Ling; Jeff Sanny; and William Moebs

Learning Objectives

By the end of this section, you will be able to:

  • Calculate the intensity relative to the central maximum of the single-slit diffraction peaks
  • Calculate the intensity relative to the central maximum of an arbitrary point on the screen

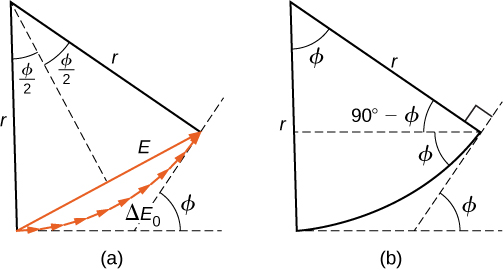
To calculate the intensity of the diffraction pattern, we follow the phasor method used for calculations with ac circuits in Alternating-Current Circuits. If we consider that there are N Huygens sources across the slit shown in (Figure), with each source separated by a distance D/N from its adjacent neighbors, the path difference between waves from adjacent sources reaching the arbitrary point P on the screen is \left(D\text{/}N\right)\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta . This distance is equivalent to a phase difference of \left(2\pi D\text{/}\lambda N\right)\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta . The phasor diagram for the waves arriving at the point whose angular position is \theta is shown in (Figure). The amplitude of the phasor for each Huygens wavelet is \text{Δ}{E}_{0}, the amplitude of the resultant phasor is E, and the phase difference between the wavelets from the first and the last sources is

\varphi =\left(\frac{2\pi }{\lambda }\right)D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta .

With N\to \infty, the phasor diagram approaches a circular arc of length N\text{Δ}{E}_{0} and radius r. Since the length of the arc is N\text{Δ}{E}_{0} for any \varphi, the radius r of the arc must decrease as \varphi increases (or equivalently, as the phasors form tighter spirals).

(a) Phasor diagram corresponding to the angular position \theta in the single-slit diffraction pattern. The phase difference between the wavelets from the first and last sources is \varphi =\left(2\pi \text{/}\lambda \right)D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta. (b) The geometry of the phasor diagram.

Figure a shows an arc with phasors labeled delta E subscript 0. This subtends an angle at the center of the circle, through two lines labeled r. This angle is bisected and each half is labeled phi by 2. The endpoints of the arc are connected by an arrow labeled E. The tangent at one endpoint of the arc is horizontal. The tangent at the other endpoint of the arc makes an angle phi with the horizontal. Figure b shows the arc and the angle phi subtended by it. A dotted line extends from one endpoint of the arc to the opposite line r. It is perpendicular to r. It makes an angle phi with the arc and an angle 90 minus phi with the adjacent line r.

The phasor diagram for \varphi =0 (the center of the diffraction pattern) is shown in (Figure)(a) using N=30. In this case, the phasors are laid end to end in a straight line of length N\text{Δ}{E}_{0}, the radius r goes to infinity, and the resultant has its maximum value E=N\text{Δ}{E}_{0}. The intensity of the light can be obtained using the relation I=\frac{1}{2}c{\epsilon }_{0}{E}^{2} from Electromagnetic Waves. The intensity of the maximum is then

{I}_{0}=\frac{1}{2}c{\epsilon }_{0}{\left(N\text{Δ}{E}_{0}\right)}^{2}=\frac{1}{2{\mu }_{0}c}{\left(N\text{Δ}{E}_{0}\right)}^{2},

where {\epsilon }_{0}=1\text{/}{\mu }_{0}{c}^{2}. The phasor diagrams for the first two zeros of the diffraction pattern are shown in parts (b) and (d) of the figure. In both cases, the phasors add to zero, after rotating through \varphi =2\pi rad for m=1 and 4\pi rad for m=2.

Phasor diagrams (with 30 phasors) for various points on the single-slit diffraction pattern. Multiple rotations around a given circle have been separated slightly so that the phasors can be seen. (a) Central maximum, (b) first minimum, (c) first maximum beyond central maximum, (d) second minimum, and (e) second maximum beyond central maximum.

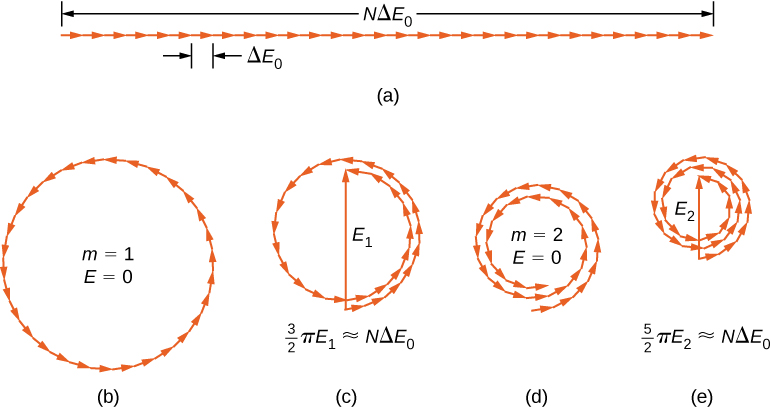
Figure a shows 30 phasors in a line of length N delta E subscript 0. The length of a phasor is delta E subscript 0. Figure b shows a circle with phasors pointing in the anticlockwise direction. This is labeled m equal to 1, E equal to 0. Figure c shows phasors along a circle. They start from the bottom and go one and a half times around the circle in the anticlockwise direction. An arrow from the starting point to the ending point is labeled E1. It forms a diameter of the circle. Figure c is labeled 3 by 2 pi E1 equal to N delta E0. Figure d shows phasors along a circle. They start from the bottom and go twice around the circle in the anticlockwise direction. The figure is labeled m equal to 2, E equal to 0. Figure e shows phasors along a circle. They start from the bottom and go two and a half times around the circle in the anticlockwise direction. An arrow from the starting point to the ending point is labeled E2. It forms a diameter of the circle. Figure c is labeled 5 by 2 pi E2 equal to N delta E0.

The next two maxima beyond the central maxima are represented by the phasor diagrams of parts (c) and (e). In part (c), the phasors have rotated through \varphi =3\pi rad and have formed a resultant phasor of magnitude {E}_{1}. The length of the arc formed by the phasors is N\text{Δ}{E}_{0}. Since this corresponds to 1.5 rotations around a circle of diameter {E}_{1}, we have

\frac{3}{2}\pi {E}_{1}\approx N\text{Δ}{E}_{0},

so

{E}_{1}=\frac{2N\text{Δ}{E}_{0}}{3\pi }

and

{I}_{1}=\frac{1}{2{\mu }_{0}c}{E}_{1}^{2}=\frac{4{\left(N\text{Δ}{E}_{0}\right)}^{2}}{\left(9{\pi }^{2}\right)\left(2{\mu }_{0}c\right)}\approx 0.045{I}_{0},

where

{I}_{0}=\frac{{\left(N\text{Δ}{E}_{0}\right)}^{2}}{2{\mu }_{0}c}.

In part (e), the phasors have rotated through \varphi =5\pi rad, corresponding to 2.5 rotations around a circle of diameter {E}_{2} and arc length N\text{Δ}{E}_{0}. This results in {I}_{2}\approx 0.016{I}_{0}. The proof is left as an exercise for the student ((Figure)).

These two maxima actually correspond to values of \varphi slightly less than 3\pi rad and 5\pi rad. Since the total length of the arc of the phasor diagram is always N\text{Δ}{E}_{0}, the radius of the arc decreases as \varphi increases. As a result, {E}_{1} and {E}_{2} turn out to be slightly larger for arcs that have not quite curled through 3\pi rad and 5\pi rad, respectively. The exact values of \varphi for the maxima are investigated in (Figure). In solving that problem, you will find that they are less than, but very close to, \varphi =3\pi ,5\pi ,7\pi ,\text{…}\phantom{\rule{0.2em}{0ex}}\text{rad}.

To calculate the intensity at an arbitrary point P on the screen, we return to the phasor diagram of (Figure). Since the arc subtends an angle \varphi at the center of the circle,

N\text{Δ}{E}_{0}=r\varphi

and

\text{sin}\phantom{\rule{0.2em}{0ex}}\left(\frac{\varphi }{2}\right)=\frac{E}{2r}.

where E is the amplitude of the resultant field. Solving the second equation for E and then substituting r from the first equation, we find

E=2r\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\frac{\varphi }{2}=2\frac{N\text{Δ}{E}_{o}}{\varphi }\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\frac{\varphi }{2}.

Now defining

\beta =\frac{\varphi }{2}=\frac{\pi D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta }{\lambda }

we obtain

E=N\text{Δ}{E}_{0}\frac{\text{sin}\phantom{\rule{0.2em}{0ex}}\beta }{\beta }

This equation relates the amplitude of the resultant field at any point in the diffraction pattern to the amplitude N\text{Δ}{E}_{0} at the central maximum. The intensity is proportional to the square of the amplitude, so

I={I}_{0}{\left(\frac{\text{sin}\phantom{\rule{0.2em}{0ex}}\beta }{\beta }\right)}^{2}

where {I}_{0}={\left(N\text{Δ}{E}_{0}\right)}^{2}\text{/}2{\mu }_{0}c is the intensity at the center of the pattern.

For the central maximum, \varphi =0, \beta is also zero and we see from l’Hôpital’s rule that {\text{lim}}_{\beta \to 0}\left(\text{sin}\phantom{\rule{0.2em}{0ex}}\beta \text{/}\beta \right)=1, so that {\text{lim}}_{\varphi \to 0}I={I}_{0}. For the next maximum, \varphi =3\pi rad, we have \beta =3\pi \text{/}2 rad and when substituted into (Figure), it yields

{I}_{1}={I}_{0}{\left(\frac{\text{sin}\phantom{\rule{0.2em}{0ex}}3\pi \text{/}2}{3\pi \text{/}2}\right)}^{2}\approx 0.045{I}_{0},

in agreement with what we found earlier in this section using the diameters and circumferences of phasor diagrams. Substituting \varphi =5\pi rad into (Figure) yields a similar result for {I}_{2}.

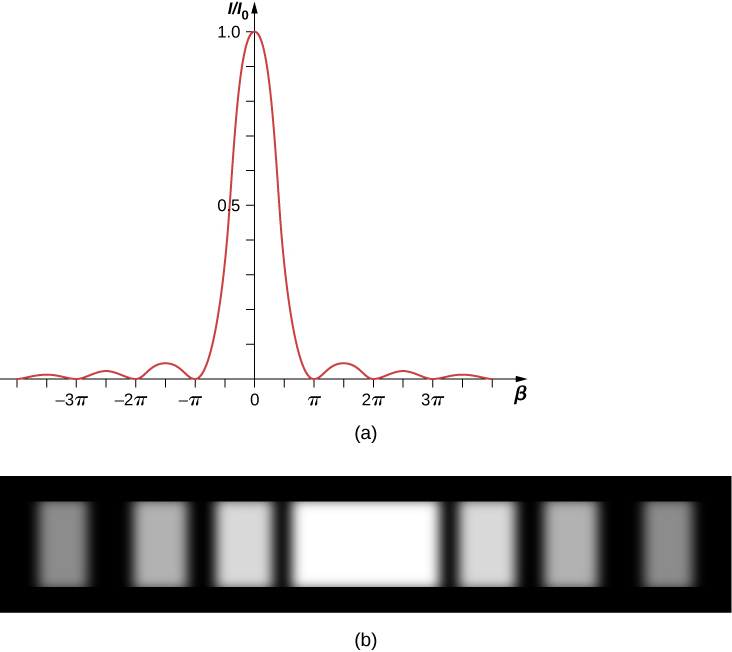
A plot of (Figure) is shown in (Figure) and directly below it is a photograph of an actual diffraction pattern. Notice that the central peak is much brighter than the others, and that the zeros of the pattern are located at those points where \text{sin}\phantom{\rule{0.2em}{0ex}}\beta =0, which occurs when \beta =m\pi rad. This corresponds to

\frac{\pi D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta }{\lambda }=m\pi ,

or

D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta =m\lambda ,

which is (Figure).

(a) The calculated intensity distribution of a single-slit diffraction pattern. (b) The actual diffraction pattern.

Figure a shows a graph of I by I0 versus beta. There is a crest at the center of the graph at beta equal to 0. The y-value of this is 1. The graph has ripples on both sides of this which grow smaller as you go outwards. The graph has zeroes at minus 3 pi, minus 2 pi, minus pi, pi, 2 pi, 3 pi. Figure b shows a strip with alternating light and dark regions. The central portion is brightest.

Intensity in Single-Slit Diffraction Light of wavelength 550 nm passes through a slit of width 2.00\phantom{\rule{0.2em}{0ex}}\mu \text{m} and produces a diffraction pattern similar to that shown in (Figure). (a) Find the locations of the first two minima in terms of the angle from the central maximum and (b) determine the intensity relative to the central maximum at a point halfway between these two minima.

Strategy The minima are given by (Figure), D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta =m\lambda. The first two minima are for m=1 and m=2. (Figure) and (Figure) can be used to determine the intensity once the angle has been worked out.

Solution

  1. Solving (Figure) for \theta gives us {\theta }_{m}={\text{sin}}^{-1}\left(m\lambda \text{/}D\right), so that
    {\theta }_{1}={\text{sin}}^{-1}\left(\frac{\left(+1\right)\left(550\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-9}\phantom{\rule{0.2em}{0ex}}\text{m}\right)}{2.00\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-6}\phantom{\rule{0.2em}{0ex}}\text{m}}\right)=+16.0\text{°}


     and

    {\theta }_{2}={\text{sin}}^{-1}\left(\frac{\left(+2\right)\left(550\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-9}\phantom{\rule{0.2em}{0ex}}\text{m}\right)}{2.00\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-6}\phantom{\rule{0.2em}{0ex}}\text{m}}\right)=+33.4\text{°}.
  2. The halfway point between {\theta }_{1} and {\theta }_{2} is
    \theta =\left({\theta }_{1}+{\theta }_{2}\right)\text{/}2=\left(16.0\text{°}+33.4\text{°}\right)\text{/}2=24.7\text{°}.

(Figure) gives

\beta =\frac{\pi D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta }{\lambda }=\frac{\pi \left(2.00\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-6}\phantom{\rule{0.2em}{0ex}}\text{m}\right)\phantom{\rule{0.2em}{0ex}}\text{sin}\left(24.7\text{°}\right)}{\left(550\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-9}\phantom{\rule{0.2em}{0ex}}\text{m}\right)}=1.52\pi \phantom{\rule{0.2em}{0ex}}\text{or}\phantom{\rule{0.2em}{0ex}}4.77\phantom{\rule{0.2em}{0ex}}\text{rad}.

From (Figure), we can calculate

\frac{I}{{I}_{o}}={\left(\frac{\text{sin}\phantom{\rule{0.2em}{0ex}}\beta }{\beta }\right)}^{2}={\left(\frac{\text{sin}\phantom{\rule{0.2em}{0ex}}\left(4.77\right)}{4.77}\right)}^{2}={\left(\frac{-0.9985}{4.77}\right)}^{2}=0.044.

Significance This position, halfway between two minima, is very close to the location of the maximum, expected near \beta =3\pi \text{/}2,\phantom{\rule{0.2em}{0ex}}\text{or}\phantom{\rule{0.2em}{0ex}}1.5\pi.

Check Your Understanding For the experiment in (Figure), at what angle from the center is the third maximum and what is its intensity relative to the central maximum?

74.3\text{°}, 0.0083{I}_{0}

If the slit width D is varied, the intensity distribution changes, as illustrated in (Figure). The central peak is distributed over the region from \text{sin}\phantom{\rule{0.2em}{0ex}}\theta =\text{−}\lambda \text{/}D to \text{sin}\phantom{\rule{0.2em}{0ex}}\theta =+\lambda \text{/}D. For small \theta, this corresponds to an angular width \text{Δ}\theta \approx 2\lambda \text{/}D. Hence, an increase in the slit width results in a decrease in the width of the central peak. For a slit with D\gg \lambda , the central peak is very sharp, whereas if D\approx \lambda, it becomes quite broad.

Single-slit diffraction patterns for various slit widths. As the slit width D increases from D=\text{λ}\phantom{\rule{0.2em}{0ex}}\text{to}\phantom{\rule{0.2em}{0ex}}5\text{λ} and then to \text{10λ}, the width of the central peak decreases as the angles for the first minima decrease as predicted by (Figure).

Figures a through c show graphs of I by I0 versus theta in degrees. Each has a wave crest with y value 1 at x=0. Figure a, labeled D equal to lambda has a broad arc. Figure b, labeled D equal to 5 lambda has a narrower crest. It has zeroes roughly between 10 and 15 and between minus 10 and minus 15. Figure c, labeled D equal to 10 lambda has a narrow crest. It has zeroes at plus and minus 5, roughly between 10 and 15 and between minus 10 and minus 15.

A diffraction experiment in optics can require a lot of preparation but this simulation by Andrew Duffy offers not only a quick set up but also the ability to change the slit width instantly. Run the simulation and select “Single slit.” You can adjust the slit width and see the effect on the diffraction pattern on a screen and as a graph.

Summary

  • The intensity pattern for diffraction due to a single slit can be calculated using phasors as
    I={I}_{0}{\left(\frac{\text{sin}\phantom{\rule{0.2em}{0ex}}\beta }{\beta }\right)}^{2},


     where \beta =\frac{\varphi }{2}=\frac{\pi D\phantom{\rule{0.2em}{0ex}}\text{sin}\phantom{\rule{0.2em}{0ex}}\theta }{\lambda }, D is the slit width, \lambda is the wavelength, and \theta is the angle from the central peak.

Conceptual Questions

In (Figure), the parameter \beta looks like an angle but is not an angle that you can measure with a protractor in the physical world. Explain what \beta represents.

The parameter \beta =\varphi \text{/}2 is the arc angle shown in the phasor diagram in (Figure). The phase difference between the first and last Huygens wavelet across the single slit is 2\beta and is related to the curvature of the arc that forms the resultant phasor that determines the light intensity.

Problems

A single slit of width 3.0\phantom{\rule{0.2em}{0ex}}\mu \text{m} is illuminated by a sodium yellow light of wavelength 589 nm. Find the intensity at a 15\text{°} angle to the axis in terms of the intensity of the central maximum.

A single slit of width 0.1 mm is illuminated by a mercury light of wavelength 576 nm. Find the intensity at a 10\text{°} angle to the axis in terms of the intensity of the central maximum.

I\text{/}{I}_{0}=2.2\phantom{\rule{0.2em}{0ex}}×\phantom{\rule{0.2em}{0ex}}{10}^{-5}

The width of the central peak in a single-slit diffraction pattern is 5.0 mm. The wavelength of the light is 600 nm, and the screen is 2.0 m from the slit. (a) What is the width of the slit? (b) Determine the ratio of the intensity at 4.5 mm from the center of the pattern to the intensity at the center.

Consider the single-slit diffraction pattern for \text{λ}=600\phantom{\rule{0.2em}{0ex}}\text{nm}, D=0.025\phantom{\rule{0.2em}{0ex}}\text{mm}, and x=2.0\phantom{\rule{0.2em}{0ex}}\text{m}. Find the intensity in terms of {I}_{o} at \theta =0.5\text{°}, 1.0\text{°}, 1.5\text{°}, 3.0\text{°}, and 10.0\text{°}.

0.63{I}_{0},\phantom{\rule{0.2em}{0ex}}0.11{I}_{0},\phantom{\rule{0.2em}{0ex}}0.0067{I}_{0},\phantom{\rule{0.2em}{0ex}}0.0062{I}_{0},\phantom{\rule{0.2em}{0ex}}0.00088{I}_{0}

Glossary

width of the central peak
angle between the minimum for m=1 and m=-1

License

Icon for the Creative Commons Attribution 4.0 International License

Intensity in Single-Slit Diffraction Copyright © by Samuel J. Ling; Jeff Sanny; and William Moebs is licensed under a Creative Commons Attribution 4.0 International License, except where otherwise noted.

Share This Book