Chapter 1 Temperature and Heat
Chapter Review
OpenStax and Paula Herrera-Siklody
Key Terms | Key Equations | Summary | Conceptual Questions | Problems | Additional Problems | Challenge Problems
Key Terms
absolute temperature scale: scale, such as Kelvin, with a zero point that is absolute zero
absolute zero: temperature at which the average kinetic energy of molecules is zero
calorie (cal): energy needed to change the temperature of 1.00 g of water by
calorimeter: container that prevents heat transfer in or out
calorimetry: study of heat transfer inside a container impervious to heat
Celsius scale: temperature scale in which the freezing point of water is
coefficient of linear expansion: (
coefficient of volume expansion: (
conduction: heat transfer through stationary matter by physical contact
convection: heat transfer by the macroscopic movement of fluid
critical point: for a given substance, the combination of temperature and pressure above which the liquid and gas phases are indistinguishable
critical pressure: pressure at the critical point
critical temperature: temperature at the critical point
degree Celsius: (
degree Fahrenheit: (
emissivity: measure of how well an object radiates
Fahrenheit scale: temperature scale in which the freezing point of water is
greenhouse effect: warming of the earth that is due to gases such as carbon dioxide and methane that absorb infrared radiation from Earth’s surface and reradiate it in all directions, thus sending some of it back toward Earth
heat: energy transferred solely due to a temperature difference
heat of fusion: energy per unit mass required to change a substance from the solid phase to the liquid phase, or released when the substance changes from liquid to solid
heat of sublimation: energy per unit mass required to change a substance from the solid phase to the vapor phase
heat of vaporization: energy per unit mass required to change a substance from the liquid phase to the vapor phase
heat transfer: movement of energy from one place or material to another as a result of a difference in temperature
Kelvin scale (K): temperature scale in which 0 K is the lowest possible temperature, representing absolute zero
kilocalorie (kcal): energy needed to change the temperature of 1.00 kg of water between
latent heat coefficient: general term for the heats of fusion, vaporization, and sublimation
mechanical equivalent of heat: work needed to produce the same effects as heat transfer
net rate of heat transfer by radiation:
phase diagram: graph of pressure vs. temperature of a particular substance, showing at which pressures and temperatures the phases of the substance occur
radiation: energy transferred by electromagnetic waves directly as a result of a temperature difference
rate of conductive heat transfer: rate of heat transfer from one material to another
specific heat: amount of heat necessary to change the temperature of 1.00 kg of a substance by
Stefan-Boltzmann law of radiation:
sublimation: phase change from solid to gas
temperature: quantity measured by a thermometer, which reflects the mechanical energy of molecules in a system
thermal conductivity: property of a material describing its ability to conduct heat
thermal equilibrium: condition in which heat no longer flows between two objects that are in contact; the two objects have the same temperature
thermal expansion: change in size or volume of an object with change in temperature
thermal stress: stress caused by thermal expansion or contraction
triple point: pressure and temperature at which a substance exists in equilibrium as a solid, liquid, and gas
vapor: gas at a temperature below the boiling temperature
vapor pressure: pressure at which a gas coexists with its solid or liquid phase
zeroth law of thermodynamics: law that states that if two objects are in thermal equilibrium, and a third object is in thermal equilibrium with one of those objects, it is also in thermal equilibrium with the other object
Key Equations
| Linear thermal expansion | |
| Thermal expansion in two dimensions | |
| Thermal expansion in three dimensions | |
| Heat transfer | |
| Transfer of heat in a calorimeter | |
| Heat due to phase change (melting and freezing) | |
| Heat due to phase change (evaporation and condensation) | |
| Rate of conductive heat transfer | |
| Net rate of heat transfer by radiation |
Summary
1.1 Temperature and Thermal Equilibrium
- Temperature is operationally defined as the quantity measured by a thermometer. It is proportional to the average kinetic energy of atoms and molecules in a system.
- Thermal equilibrium occurs when two bodies are in contact with each other and can freely exchange energy. Systems are in thermal equilibrium when they have the same temperature.
- The zeroth law of thermodynamics states that when two systems, A and B, are in thermal equilibrium with each other, and B is in thermal equilibrium with a third system C, then A is also in thermal equilibrium with C.
1.2 Thermometers and Temperature Scales
- Three types of thermometers are alcohol, liquid crystal, and infrared radiation (pyrometer).
- The three main temperature scales are Celsius, Fahrenheit, and Kelvin. Temperatures can be converted from one scale to another using temperature conversion equations.
- The three phases of water (ice, liquid water, and water vapor) can coexist at a single pressure and temperature known as the triple point.
1.3 Thermal Expansion
- Thermal expansion is the increase of the size (length, area, or volume) of a body due to a change in temperature, usually a rise. Thermal contraction is the decrease in size due to a change in temperature, usually a fall in temperature.
- Thermal stress is created when thermal expansion or contraction is constrained.
1.4 Heat Transfer, Specific Heat, and Calorimetry
- Heat and work are the two distinct methods of energy transfer.
- Heat transfer to an object when its temperature changes is often approximated well by
1.5 Phase Changes
- Most substances have three distinct phases (under ordinary conditions on Earth), and they depend on temperature and pressure.
- Two phases coexist (i.e., they are in thermal equilibrium) at a set of pressures and temperatures.
- Phase changes occur at fixed temperatures for a given substance at a given pressure, and these temperatures are called boiling, freezing (or melting), and sublimation points.
1.6 Mechanisms of Heat Transfer
- Heat is transferred by three different methods: conduction, convection, and radiation.
- Heat conduction is the transfer of heat between two objects in direct contact with each other.
- The rate of heat transfer P (energy per unit time) is proportional to the temperature difference
- Convection is heat transfer by the macroscopic movement of mass. Convection can be natural or forced, and generally transfers thermal energy faster than conduction. Convection that occurs along with a phase change can transfer energy from cold regions to warm ones.
- Radiation is heat transfer through the emission or absorption of electromagnetic waves.
- The rate of radiative heat transfer is proportional to the emissivity e. For a perfect blackbody,
- The rate of heat transfer depends on the surface area and the fourth power of the absolute temperature:
where
where
Conceptual Questions
1.1 Temperature and Thermal Equilibrium
- What does it mean to say that two systems are in thermal equilibrium?
- Give an example in which A has some kind of non-thermal equilibrium relationship with B, and B has the same relationship with C, but A does not have that relationship with C.
1.2 Thermometers and Temperature Scales
- If a thermometer is allowed to come to equilibrium with the air, and a glass of water is not in equilibrium with the air, what will happen to the thermometer reading when it is placed in the water?
- Give an example of a physical property that varies with temperature and describe how it is used to measure temperature.
1.3 Thermal Expansion
- Pouring cold water into hot glass or ceramic cookware can easily break it. What causes the breaking? Explain why Pyrex®, a glass with a small coefficient of linear expansion, is less susceptible.
- One method of getting a tight fit, say of a metal peg in a hole in a metal block, is to manufacture the peg slightly larger than the hole. The peg is then inserted when at a different temperature than the block. Should the block be hotter or colder than the peg during insertion? Explain your answer.
- Does it really help to run hot water over a tight metal lid on a glass jar before trying to open it? Explain your answer.
- When a cold alcohol thermometer is placed in a hot liquid, the column of alcohol goes down slightly before going up. Explain why.
- Calculate the length of a 1-meter rod of a material with thermal expansion coefficient
- Noting the large stresses that can be caused by thermal expansion, an amateur weapon inventor decides to use it to make a new kind of gun. He plans to jam a bullet against an aluminum rod inside a closed invar tube. When he heats the tube, the rod will expand more than the tube and a very strong force will build up. Then, by a method yet to be determined, he will open the tube in a split second and let the force of the rod launch the bullet at very high speed. What is he overlooking?
1.4 Heat Transfer, Specific Heat, and Calorimetry
- How is heat transfer related to temperature?
- Describe a situation in which heat transfer occurs.
- When heat transfers into a system, is the energy stored as heat? Explain briefly.
- The brakes in a car increase in temperature by
1.5 Phase Changes
- A pressure cooker contains water and steam in equilibrium at a pressure greater than atmospheric pressure. How does this greater pressure increase cooking speed?
- As shown below, which is the phase diagram for carbon dioxide, what is the vapor pressure of solid carbon dioxide (dry ice) at
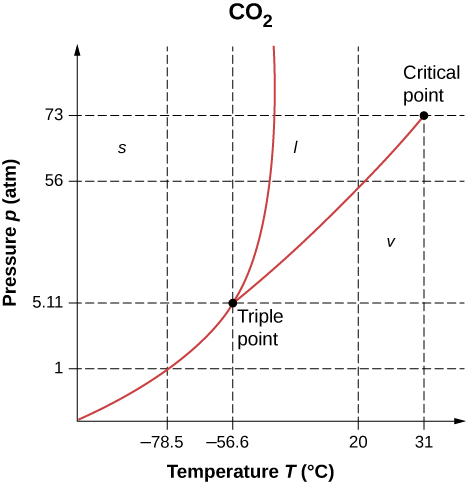
- Can carbon dioxide be liquefied at room temperature (
- What is the distinction between gas and vapor?
- Heat transfer can cause temperature and phase changes. What else can cause these changes?
- How does the latent heat of fusion of water help slow the decrease of air temperatures, perhaps preventing temperatures from falling significantly below
- What is the temperature of ice right after it is formed by freezing water?
- If you place
- What effect does condensation on a glass of ice water have on the rate at which the ice melts? Will the condensation speed up the melting process or slow it down?
- In Miami, Florida, which has a very humid climate and numerous bodies of water nearby, it is unusual for temperatures to rise above about
- In winter, it is often warmer in San Francisco than in Sacramento, 150 km inland. In summer, it is nearly always hotter in Sacramento. Explain how the bodies of water surrounding San Francisco moderate its extreme temperatures.
- Freeze-dried foods have been dehydrated in a vacuum. During the process, the food freezes and must be heated to facilitate dehydration. Explain both how the vacuum speeds up dehydration and why the food freezes as a result.
- In a physics classroom demonstration, an instructor inflates a balloon by mouth and then cools it in liquid nitrogen. When cold, the shrunken balloon has a small amount of light blue liquid in it, as well as some snow-like crystals. As it warms up, the liquid boils, and part of the crystals sublime, with some crystals lingering for a while and then producing a liquid. Identify the blue liquid and the two solids in the cold balloon. Justify your identifications using data from Table 1.4.
1.6 Mechanisms of Heat Transfer
- What are the main methods of heat transfer from the hot core of Earth to its surface? From Earth’s surface to outer space?
- When our bodies get too warm, they respond by sweating and increasing blood circulation to the surface to transfer thermal energy away from the core. What effect will those processes have on a person in a
- Shown below is a cut-away drawing of a thermos bottle (also known as a Dewar flask), which is a device designed specifically to slow down all forms of heat transfer. Explain the functions of the various parts, such as the vacuum, the silvering of the walls, the thin-walled long glass neck, the rubber support, the air layer, and the stopper. Figure shows the cross section of a thermos. Glass walls with silvered surfaces form the inner container. It is suspended within the outer container with springs and rubber supports. There is a layer of air and a layer of vacuum between the two containers. the inner container is filled with hot or cold liquid.

- Some electric stoves have a flat ceramic surface with heating elements hidden beneath. A pot placed over a heating element will be heated, while the surface only a few centimeters away is safe to touch. Why is ceramic, with a conductivity less than that of a metal but greater than that of a good insulator, an ideal choice for the stove top?
- Loose-fitting white clothing covering most of the body, shown below, is ideal for desert dwellers, both in the hot Sun and during cold evenings. Explain how such clothing is advantageous during both day and night.

- One way to make a fireplace more energy-efficient is to have room air circulate around the outside of the fire box and back into the room. Detail the methods of heat transfer involved.
- On cold, clear nights horses will sleep under the cover of large trees. How does this help them keep warm?
- When watching a circus during the day in a large, dark-colored tent, you sense significant heat transfer from the tent. Explain why this occurs.
- Satellites designed to observe the radiation from cold (3 K) dark space have sensors that are shaded from the Sun, Earth, and the Moon and are cooled to very low temperatures. Why must the sensors be at low temperature?
- Why are thermometers that are used in weather stations shielded from the sunshine? What does a thermometer measure if it is shielded from the sunshine? What does it measure if it is not?
- Putting a lid on a boiling pot greatly reduces the heat transfer necessary to keep it boiling. Explain why.
- Your house will be empty for a while in cold weather, and you want to save energy and money. Should you turn the thermostat down to the lowest level that will protect the house from damage such as freezing pipes, or leave it at the normal temperature? (If you don’t like coming back to a cold house, imagine that a timer controls the heating system so the house will be warm when you get back.) Explain your answer.
- You pour coffee into an unlidded cup, intending to drink it 5 minutes later. You can add cream when you pour the cup or right before you drink it. (The cream is at the same temperature either way. Assume that the cream and coffee come into thermal equilibrium with each other very quickly.) Which way will give you hotter coffee? What feature of this question is different from the previous one?
- Broiling is a method of cooking by radiation, which produces somewhat different results from cooking by conduction or convection. A gas flame or electric heating element produces a very high temperature close to the food and above it. Why is radiation the dominant heat-transfer method in this situation?
- On a cold winter morning, why does the metal of a bike feel colder than the wood of a porch?
Problems
1.2 Thermometers and Temperature Scales
- While traveling outside the United States, you feel sick. A companion gets you a thermometer, which says your temperature is 39. What scale is that on? What is your Fahrenheit temperature? Should you seek medical help?
- What are the following temperatures on the Kelvin scale?
(a)
(b)
(c) - (a) Suppose a cold front blows into your locale and drops the temperature by 40.0 Fahrenheit degrees. How many degrees Celsius does the temperature decrease when it decreases by
(b) Show that any change in temperature in Fahrenheit degrees is nine-fifths the change in Celsius degrees - An Associated Press article on climate change said, “Some of the ice shelf’s disappearance was probably during times when the planet was 36 degrees Fahrenheit (2 degrees Celsius) to 37 degrees Fahrenheit (3 degrees Celsius) warmer than it is today.” What mistake did the reporter make?
- (a) At what temperature do the Fahrenheit and Celsius scales have the same numerical value?
(b) At what temperature do the Fahrenheit and Kelvin scales have the same numerical value? - A person taking a reading of the temperature in a freezer in Celsius makes two mistakes: first omitting the negative sign and then thinking the temperature is Fahrenheit. That is, the person reads
1.3 Thermal Expansion
- The height of the Washington Monument is measured to be 170.00 m on a day when the temperature is
- How much taller does the Eiffel Tower become at the end of a day when the temperature has increased by
- What is the change in length of a 3.00-cm-long column of mercury if its temperature changes from
- How large an expansion gap should be left between steel railroad rails if they may reach a maximum temperature
- You are looking to buy a small piece of land in Hong Kong. The price is “only” 60,000 dollars per square meter. The land title says the dimensions are
- Global warming will produce rising sea levels partly due to melting ice caps and partly due to the expansion of water as average ocean temperatures rise. To get some idea of the size of this effect, calculate the change in length of a column of water 1.00 km high for a temperature increase of
- (a) Suppose a meter stick made of steel and one made of aluminum are the same length at
(b) Repeat the calculation for two 30.0-m-long surveyor’s tapes. - (a) If a 500-mL glass beaker is filled to the brim with ethyl alcohol at a temperature of
(b) How much less water would overflow under the same conditions? - Most cars have a coolant reservoir to catch radiator fluid that may overflow when the engine is hot. A radiator is made of copper and is filled to its 16.0-L capacity when at
- A physicist makes a cup of instant coffee and notices that, as the coffee cools, its level drops 3.00 mm in the glass cup. Show that this decrease cannot be due to thermal contraction by calculating the decrease in level if the
- The density of water at
- Show that
1.4 Heat Transfer, Specific Heat, and Calorimetry
- On a hot day, the temperature of an 80,000-L swimming pool increases by
- To sterilize a 50.0-g glass baby bottle, we must raise its temperature from
- The same heat transfer into identical masses of different substances produces different temperature changes. Calculate the final temperature when 1.00 kcal of heat transfers into 1.00 kg of the following, originally at
- Rubbing your hands together warms them by converting work into thermal energy. If a woman rubs her hands back and forth for a total of 20 rubs, at a distance of 7.50 cm per rub, and with an average frictional force of 40.0 N, what is the temperature increase? The mass of tissues warmed is only 0.100 kg, mostly in the palms and fingers.
- A 0.250-kg block of a pure material is heated from
- Suppose identical amounts of heat transfer into different masses of copper and water, causing identical changes in temperature. What is the ratio of the mass of copper to water?
- (a) The number of kilocalories in food is determined by calorimetry techniques in which the food is burned and the amount of heat transfer is measured. How many kilocalories per gram are there in a 5.00-g peanut if the energy from burning it is transferred to 0.500 kg of water held in a 0.100-kg aluminum cup, causing a
(b) Compare your answer to the following labeling information found on a package of dry roasted peanuts: a serving of 33 g contains 200 calories. Comment on whether the values are consistent. - Following vigorous exercise, the body temperature of an 80.0 kg person is
- In a study of healthy young men[1], doing 20 push-ups in 1 minute burned an amount of energy per kg that for a 70.0-kg man corresponds to 8.06 calories (kcal). How much would a 70.0-kg man’s temperature rise if he did not lose any heat during that time?
- A 1.28-kg sample of water at
- Repeat the preceding problem, assuming the water is in a glass beaker with a mass of 0.200 kg, which in turn is in a calorimeter. The beaker is initially at the same temperature as the water. Before doing the problem, should the answer be higher or lower than the preceding answer? Comparing the mass and specific heat of the beaker to those of the water, do you think the beaker will make much difference?
1.5 Phase Changes
- How much heat transfer (in kilocalories) is required to thaw a 0.450-kg package of frozen vegetables originally at
- A bag containing
(a) How much heat transfer is necessary to raise the temperature of 0.800 kg of water from
(b) How much heat transfer is required to first melt 0.800 kg of
(c) Explain how your answer supports the contention that the ice is more effective. - (a) How much heat transfer is required to raise the temperature of a 0.750-kg aluminum pot containing 2.50 kg of water from
(b) How long does this take if the rate of heat transfer is 500 W? - Condensation on a glass of ice water causes the ice to melt faster than it would otherwise. If 8.00 g of vapor condense on a glass containing both water and 200 g of ice, how many grams of the ice will melt as a result? Assume no other heat transfer occurs. Use
- On a trip, you notice that a 3.50-kg bag of ice lasts an average of one day in your cooler. What is the average power in watts entering the ice if it starts at
- On a certain dry sunny day, a swimming pool’s temperature would rise by
- (a) How much heat transfer is necessary to raise the temperature of a 0.200-kg piece of ice from
(b) How much time is required for each stage, assuming a constant 20.0 kJ/s rate of heat transfer?
(c) Make a graph of temperature versus time for this process. - In 1986, an enormous iceberg broke away from the Ross Ice Shelf in Antarctica. It was an approximately rectangular prism 160 km long, 40.0 km wide, and 250 m thick.
(a) What is the mass of this iceberg, given that the density of ice is
(b) How much heat transfer (in joules) is needed to melt it?
(c) How many years would it take sunlight alone to melt ice this thick, if the ice absorbs an average of - How many grams of coffee must evaporate from 350 g of coffee in a 100-g glass cup to cool the coffee and the cup from
- (a) It is difficult to extinguish a fire on a crude oil tanker, because each liter of crude oil releases
(b) Discuss additional complications caused by the fact that crude oil is less dense than water. - The energy released from condensation in thunderstorms can be very large. Calculate the energy released into the atmosphere for a small storm of radius 1 km, assuming that 1.0 cm of rain is precipitated uniformly over this area.
- To help prevent frost damage, 4.00 kg of water at
(a) How much heat transfer occurs as the water freezes?
(b) How much would the temperature of the 200-kg tree decrease if this amount of heat transferred from the tree? Take the specific heat to be - A 0.250-kg aluminum bowl holding
- A 0.0500-kg ice cube at
- If you pour 0.0100 kg of
- Indigenous people sometimes cook in watertight baskets by placing hot rocks into water to bring it to a boil. What mass of
- What would the final temperature of the pan and water be in Example 1.7 if 0.260 kg of water were placed in the pan and 0.0100 kg of the water evaporated immediately, leaving the remainder to come to a common temperature with the pan?
1.6 Mechanisms of Heat Transfer
- (a) Calculate the rate of heat conduction through house walls that are 13.0 cm thick and have an average thermal conductivity twice that of glass wool. Assume there are no windows or doors. The walls’ surface area is
(b) How many 1-kW room heaters would be needed to balance the heat transfer due to conduction? - The rate of heat conduction out of a window on a winter day is rapid enough to chill the air next to it. To see just how rapidly the windows transfer heat by conduction, calculate the rate of conduction in watts through a
- Calculate the rate of heat conduction out of the human body, assuming that the core internal temperature is
- Suppose you stand with one foot on ceramic flooring and one foot on a wool carpet, making contact over an area of
- A man consumes 3000 kcal of food in one day, converting most of it to thermal energy to maintain body temperature. If he loses half this energy by evaporating water (through breathing and sweating), how many kilograms of water evaporate?
- A firewalker runs across a bed of hot coals without sustaining burns. Calculate the heat transferred by conduction into the sole of one foot of a firewalker given that the bottom of the foot is a 3.00-mm-thick callus with a conductivity at the low end of the range for wood and its density is
- (a) What is the rate of heat conduction through the 3.00-cm-thick fur of a large animal having a
(b) What food intake will the animal need in one day to replace this heat transfer? - A walrus transfers energy by conduction through its blubber at the rate of 150 W when immersed in
- Compare the rate of heat conduction through a 13.0-cm-thick wall that has an area of
- Suppose a person is covered head to foot by wool clothing with average thickness of 2.00 cm and is transferring energy by conduction through the clothing at the rate of 50.0 W. What is the temperature difference across the clothing, given the surface area is
- Some stove tops are smooth ceramic for easy cleaning. If the ceramic is 0.600 cm thick and heat conduction occurs through the same area and at the same rate as computed in Example 1.11, what is the temperature difference across it? Ceramic has the same thermal conductivity as glass and brick.
- One easy way to reduce heating (and cooling) costs is to add extra insulation in the attic of a house. Suppose a single-story cubical house already had 15 cm of fiberglass insulation in the attic and in all the exterior surfaces. If you added an extra 8.0 cm of fiberglass to the attic, by what percentage would the heating cost of the house drop? Take the house to have dimensions 10 m by 15 m by 3.0 m. Ignore air infiltration and heat loss through windows and doors, and assume that the interior is uniformly at one temperature and the exterior is uniformly at another.
- Many decisions are made on the basis of the payback period: the time it will take through savings to equal the capital cost of an investment. Acceptable payback times depend upon the business or philosophy one has. (For some industries, a payback period is as small as 2 years.) Suppose you wish to install the extra insulation in the preceding problem. If energy cost 1.00 dollar per million joules and the insulation was 4.00 dollars per square meter, then calculate the simple payback time. Take the average
Additional Problems
- In 1701, the Danish astronomer Ole Rømer proposed a temperature scale with two fixed points, freezing water at 7.5 degrees, and boiling water at 60.0 degrees. What is the boiling point of oxygen, 90.2 K, on the Rømer scale?
- What is the percent error of thinking the melting point of tungsten is
- An engineer wants to design a structure in which the difference in length between a steel beam and an aluminum beam remains at 0.500 m regardless of temperature, for ordinary temperatures. What must the lengths of the beams be?
- How much stress is created in a steel beam if its temperature changes from
- A brass rod (
- A mercury thermometer still in use for meteorology has a bulb with a volume of
- Even when shut down after a period of normal use, a large commercial nuclear reactor transfers thermal energy at the rate of 150 MW by the radioactive decay of fission products. This heat transfer causes a rapid increase in temperature if the cooling system fails
(a) Calculate the rate of temperature increase in degrees Celsius per second (
(b) How long would it take to obtain a temperature increase of - You leave a pastry in the refrigerator on a plate and ask your roommate to take it out before you get home so you can eat it at room temperature, the way you like it. Instead, your roommate plays video games for hours. When you return, you notice that the pastry is still cold, but the game console has become hot. Annoyed, and knowing that the pastry will not be good if it is microwaved, you warm up the pastry by unplugging the console and putting it in a clean trash bag (which acts as a perfect calorimeter) with the pastry on the plate. After a while, you find that the equilibrium temperature is a nice, warm
- Two solid spheres, A and B, made of the same material, are at temperatures of
- In some countries, liquid nitrogen is used on dairy trucks instead of mechanical refrigerators. A 3.00-hour delivery trip requires 200 L of liquid nitrogen, which has a density of
(a) Calculate the heat transfer necessary to evaporate this amount of liquid nitrogen and raise its temperature to
(b) What is this heat transfer rate in kilowatt-hours?
(c) Compare the amount of cooling obtained from melting an identical mass of - Some gun fanciers make their own bullets, which involves melting lead and casting it into lead slugs. How much heat transfer is needed to raise the temperature and melt 0.500 kg of lead, starting from
- A 0.800-kg iron cylinder at a temperature of
- Repeat the preceding problem with 2.00 kg of ice instead of 1.00 kg.
- Repeat the preceding problem with 0.500 kg of ice, assuming that the ice is initially in a copper container of mass 1.50 kg in equilibrium with the ice.
- A 30.0-g ice cube at its melting point is dropped into an aluminum calorimeter of mass 100.0 g in equilibrium at
- (a) Calculate the rate of heat conduction through a double-paned window that has a
(b) Calculate the rate of heat conduction through a 1.60-cm-thick window of the same area and with the same temperatures. Compare your answer with that for part (a). - (a) An exterior wall of a house is 3 m tall and 10 m wide. It consists of a layer of drywall with an R factor of 0.56, a layer 3.5 inches thick filled with fiberglass batts, and a layer of insulated siding with an R factor of 2.6. The wall is built so well that there are no leaks of air through it. When the inside of the wall is at
(b) More realistically, the 3.5-inch space also contains 2-by-4 studs—wooden boards 1.5 inches by 3.5 inches oriented so that 3.5-inch dimension extends from the drywall to the siding. They are “on 16-inch centers,” that is, the centers of the studs are 16 inches apart. What is the heat current in this situation? Don’t worry about one stud more or less. - For the human body, what is the rate of heat transfer by conduction through the body’s tissue with the following conditions: the tissue thickness is 3.00 cm, the difference in temperature is
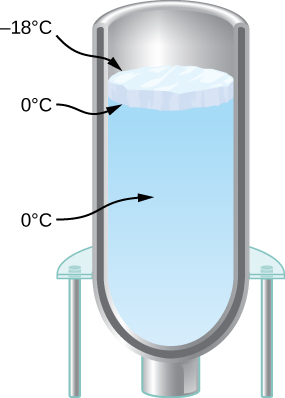
- You have a Dewar flask (a laboratory vacuum flask) that has an open top and straight sides, as shown below. You fill it with water and put it into the freezer. It is effectively a perfect insulator, blocking all heat transfer, except on the top. After a time, ice forms on the surface of the water. The liquid water and the bottom surface of the ice, in contact with the liquid water, are at
- An infrared heater for a sauna has a surface area of
- (a) Determine the power of radiation from the Sun by noting that the intensity of the radiation at the distance of Earth is
(b) Assuming that the Sun’s temperature is 5780 K and that its emissivity is 1, find its radius.
Challenge Problems
- A pendulum is made of a rod of length L and negligible mass, but capable of thermal expansion, and a weight of negligible size.
(a) Show that when the temperature increases by dT, the period of the pendulum increases by a fraction
(b) A clock controlled by a brass pendulum keeps time correctly at - At temperatures of a few hundred kelvins the specific heat capacity of copper approximately follows the empirical formula
- In a calorimeter of negligible heat capacity, 200 g of steam at
- An astronaut performing an extra-vehicular activity (space walk) shaded from the Sun is wearing a spacesuit that can be approximated as perfectly white (
- Find the growth of an ice layer as a function of time in a Dewar flask as seen in Exercise 1.120. Call the thickness of the ice layer L. (a) Derive an equation for dL/dt in terms of L , the temperature T above the ice, and the properties of ice (which you can leave in symbolic form instead of substituting the numbers). (b) Solve this differential equation assuming that at
- As the very first rudiment of climatology, estimate the temperature of Earth. Assume it is a perfect sphere and its temperature is uniform. Ignore the greenhouse effect. Thermal radiation from the Sun has an intensity (the “solar constant” S) of about
(a) Assuming the Sun’s rays are parallel, what area must S be multiplied by to get the total radiation intercepted by Earth? It will be easiest to answer in terms of Earth’s radius, R.
(b) Assume that Earth reflects about 30% of the solar energy it intercepts. In other words, Earth has an albedo with a value of
(c) Find the temperature at which Earth radiates energy at the same rate. Assume that at the infrared wavelengths where it radiates, the emissivity e is 1. Does your result show that the greenhouse effect is important?
(d) How does your answer depend on the the area of Earth? - Let’s stop ignoring the greenhouse effect and incorporate it into the previous problem in a very rough way. Assume the atmosphere is a single layer, a spherical shell around Earth, with an emissivity
- In terms of Earth’s radius, the constant
- What is the power of Earth’s radiation absorbed by the atmosphere?
- In terms of the unknown temperature
- Write an equation that says the power of the radiation the atmosphere absorbs from Earth equals the power of the radiation it emits.
- Half of the power radiated by the atmosphere hits Earth. Write an equation that says that the power Earth absorbs from the atmosphere and the Sun equals the power that it emits.
- Solve your two equations for the unknown temperature of Earth.
For steps that make this model less crude, see for example the lectures by Paul O’Gorman.
- In terms of Earth’s radius, the constant
Media Attributions
- UP2.F1
- JW Vezina, “An examination of the differences between two methods of estimating energy expenditure in resistance training activities,” Journal of Strength and Conditioning Research, April 28, 2014, http://www.ncbi.nlm.nih.gov/pubmed/24402448 ↵

