We have seen in previous chapters that energy is one of the fundamental concepts of physics. Heat is a type of energy transfer that is caused by a temperature difference, and it can change the temperature of an object. As we learned earlier in this chapter, heat transfer is the movement of energy from one place or material to another as a result of a difference in temperature. Heat transfer is fundamental to such everyday activities as home heating and cooking, as well as many industrial processes. It also forms a basis for the topics in the remainder of this chapter.

We also introduce the concept of internal energy, which can be increased or decreased by heat transfer. We discuss another way to change the internal energy of a system, namely doing work on it. Thus, we are beginning the study of the relationship of heat and work, which is the basis of engines and refrigerators and the central topic (and origin of the name) of thermodynamics.

Internal Energy and Heat

A thermal system has internal energy (also called thermal energy), which is the sum of the mechanical energies of its molecules. A system’s internal energy is proportional to its temperature. As we saw earlier in this chapter, if two objects at different temperatures are brought into contact with each other, energy is transferred from the hotter to the colder object until the bodies reach thermal equilibrium (that is, they are at the same temperature). No work is done by either object because no force acts through a distance (as we discussed in Work and Kinetic Energy). These observations reveal that heat is energy transferred spontaneously due to a temperature difference. Figure 1.9 shows an example of heat transfer.

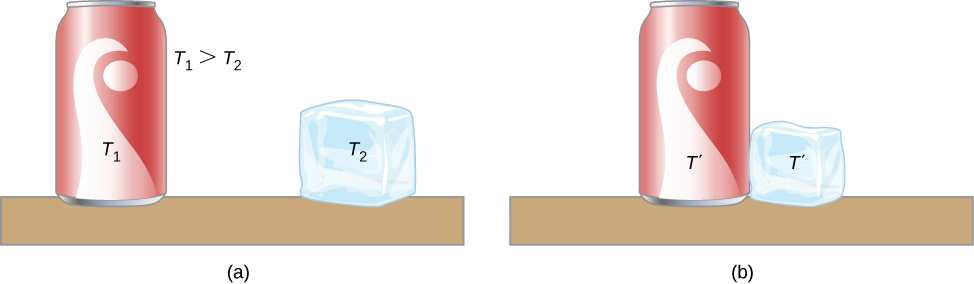
The meaning of “heat” in physics is different from its ordinary meaning. For example, in conversation, we may say “the heat was unbearable,” but in physics, we would say that the temperature was high. Heat is a form of energy flow, whereas temperature is not. Incidentally, humans are sensitive to heat flow rather than to temperature.

Since heat is a form of energy, its SI unit is the joule (J). Another common unit of energy often used for heat is the calorie (cal), defined as the energy needed to change the temperature of 1.00 g of water by ${1.00}^{\circ }\text{C}$—specifically, between ${14.5}^{\circ }\text{C}$ and ${15.5}^{\circ }\text{C}$, since there is a slight temperature dependence. Also commonly used is the kilocalorie (kcal), which is the energy needed to change the temperature of 1.00 kg of water by ${1.00}^{\circ }\text{C}$. Since mass is most often specified in kilograms, the kilocalorie is convenient. Confusingly, food calories (sometimes called “big calories,” abbreviated Cal) are actually kilocalories, a fact not easily determined from package labeling.

Mechanical Equivalent of Heat

It is also possible to change the temperature of a substance by doing work, which transfers energy into or out of a system. This realization helped establish that heat is a form of energy. James Prescott Joule (1818–1889) performed many experiments to establish the mechanical equivalent of heat—the work needed to produce the same effects as heat transfer. In the units used for these two quantities, the value for this equivalence is

$$1.000\text{kcal}=4186\text{J}.$$

We consider this equation to represent the conversion between two units of energy. (Other numbers that you may see refer to calories defined for temperature ranges other than ${14.5}^{\circ }\text{C}$ to ${15.5}^{\circ }\text{C}$.)

Figure 1.10 shows one of Joule’s most famous experimental setups for demonstrating that work and heat can produce the same effects and measuring the mechanical equivalent of heat. It helped establish the principle of conservation of energy. Gravitational potential energy (U) was converted into kinetic energy (K), and then randomized by viscosity and turbulence into increased average kinetic energy of atoms and molecules in the system, producing a temperature increase. Joule’s contributions to thermodynamics were so significant that the SI unit of energy was named after him.

Increasing internal energy by heat transfer gives the same result as increasing it by doing work. Therefore, although a system has a well-defined internal energy, we cannot say that it has a certain “heat content” or “work content.” A well-defined quantity that depends only on the current state of the system, rather than on the history of that system, is known as a state variable. Temperature and internal energy are state variables. To sum up this paragraph, heat and work are not state variables.

Incidentally, increasing the internal energy of a system does not necessarily increase its temperature. As we’ll see in the next section, the temperature does not change when a substance changes from one phase to another. An example is the melting of ice, which can be accomplished by adding heat or by doing frictional work, as when an ice cube is rubbed against a rough surface.

Temperature Change and Heat Capacity

We have noted that heat transfer often causes temperature change. Experiments show that with no phase change and no work done on or by the system, the transferred heat is typically directly proportional to the change in temperature and to the mass of the system, to a good approximation. (Below we show how to handle situations where the approximation is not valid.) The constant of proportionality depends on the substance and its phase, which may be gas, liquid, or solid. We omit discussion of the fourth phase, plasma, because although it is the most common phase in the universe, it is rare and short-lived on Earth.

We can understand the experimental facts by noting that the transferred heat is the change in the internal energy, which is the total energy of the molecules. Under typical conditions, the total kinetic energy of the molecules $K_{\text{total}}$ is a constant fraction of the internal energy (for reasons and with exceptions that we’ll see in the next chapter). The average kinetic energy of a molecule $K_{\text{ave}}$ is proportional to the absolute temperature. Therefore, the change in internal energy of a system is typically proportional to the change in temperature and to the number of molecules, N. Mathematically, $\mathrm{\Delta }U\propto \mathrm{\Delta }{K}_{\text{total}}=N{K}_{\text{ave}}\propto N\mathrm{\Delta }T$ The dependence on the substance results in large part from the different masses of atoms and molecules. We are considering its heat capacity in terms of its mass, but as we will see in the next chapter, in some cases, heat capacities per molecule are similar for different substances. The dependence on substance and phase also results from differences in the potential energy associated with interactions between atoms and molecules.

Values of specific heat must generally be measured, because there is no simple way to calculate them precisely. Table 1.3 lists representative values of specific heat for various substances. We see from this table that the specific heat of water is five times that of glass and 10 times that of iron, which means that it takes five times as much heat to raise the temperature of water a given amount as for glass, and 10 times as much as for iron. In fact, water has one of the largest specific heats of any material, which is important for sustaining life on Earth.

The specific heats of gases depend on what is maintained constant during the heating—typically either the volume or the pressure. In the table, the first specific heat value for each gas is measured at constant volume, and the second (in parentheses) is measured at constant pressure. We will return to this topic in the chapter on the kinetic theory of gases.

In general, specific heat also depends on temperature. Thus, a precise definition of c for a substance must be given in terms of an infinitesimal change in temperature. To do this, we note that $c=\frac{1}{m}\frac{\mathrm{\Delta }Q}{\mathrm{\Delta }T}$ and replace $\mathrm{\Delta }$ with d:

$$c=\frac{1}{m}\frac{dQ}{dT}.$$

Except for gases, the temperature and volume dependence of the specific heat of most substances is weak at normal temperatures. Therefore, we will generally take specific heats to be constant at the values given in the table.

Example 1.6 illustrates a temperature rise caused by doing work. (The result is the same as if the same amount of energy had been added with a blowtorch instead of mechanically.)

Example 1.6

Calculating the Temperature Increase from the Work Done on a Substance
Truck brakes used to control speed on a downhill run do work, converting gravitational potential energy into increased internal energy (higher temperature) of the brake material (Figure 1.11). This conversion prevents the gravitational potential energy from being converted into kinetic energy of the truck. Since the mass of the truck is much greater than that of the brake material absorbing the energy, the temperature increase may occur too fast for sufficient heat to transfer from the brakes to the environment; in other words, the brakes may overheat.

Calculate the temperature increase of 10 kg of brake material with an average specific heat of $800J/\text{ kg}{\cdot }^{\circ }C$ if the material retains 10% of the energy from a 10,000-kg truck descending 75.0 m (in vertical displacement) at a constant speed.

Strategy
We calculate the gravitational potential energy (Mgh) that the entire truck loses in its descent, equate it to the increase in the brakes’ internal energy, and then find the temperature increase produced in the brake material alone.

Solution
First we calculate the change in gravitational potential energy as the truck goes downhill:

$$Mgh=\left(\mathrm{10,000}\text{kg}\right)\left(9.80{\text{m/s}}^2\right)\left(75.0\text{m}\right)=7.35\times {10}^6\text{J}.$$Because the kinetic energy of the truck does not change, conservation of energy tells us the lost potential energy is dissipated, and we assume that 10% of it is transferred to internal energy of the brakes, so take $Q=Mgh/10$. Then we calculate the temperature change from the heat transferred, using

$$\text{Δ}T=\frac{Q}{mc},$$where m is the mass of the brake material. Insert the given values to find

$$\text{Δ}T=\frac{7.35\times {10}^5\text{J}}{(10\text{kg})(800\text{J/kg}\text{°C})}=92\text{°}\text{C}.$$Significance
If the truck had been traveling for some time, then just before the descent, the brake temperature would probably be higher than the ambient temperature. The temperature increase in the descent would likely raise the temperature of the brake material very high, so this technique is not practical. Instead, the truck would use the technique of engine braking. A different idea underlies the recent technology of hybrid and electric cars, where mechanical energy (kinetic and gravitational potential energy) is converted by the brakes into electrical energy in the battery, a process called regenerative braking.

In a common kind of problem, objects at different temperatures are placed in contact with each other but isolated from everything else, and they are allowed to come into equilibrium. A container that prevents heat transfer in or out is called a calorimeter, and the use of a calorimeter to make measurements (typically of heat or specific heat capacity) is called calorimetry.

We will use the term “calorimetry problem” to refer to any problem in which the objects concerned are thermally isolated from their surroundings. An important idea in solving calorimetry problems is that during a heat transfer between objects isolated from their surroundings, the heat gained by the colder object must equal the heat lost by the hotter object, due to conservation of energy:

We express this idea by writing that the sum of the heats equals zero because the heat gained is usually considered positive; the heat lost, negative.